1/6 Divided By 3 As A Fraction
Arias News
May 11, 2025 · 5 min read
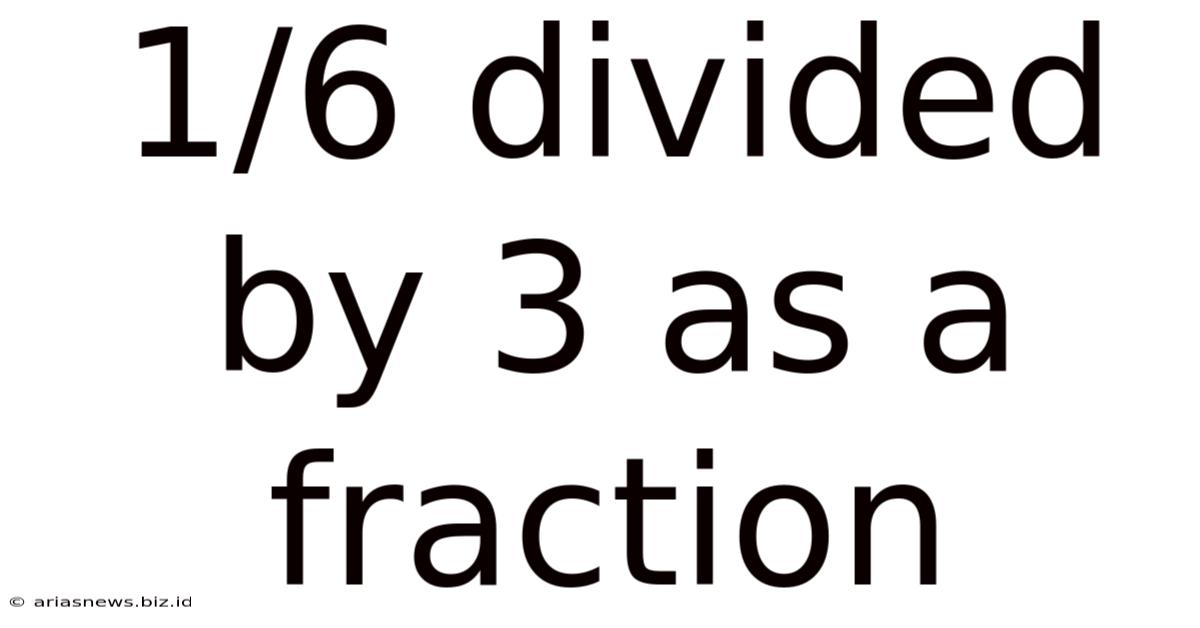
Table of Contents
1/6 Divided by 3: A Comprehensive Guide to Fraction Division
Dividing fractions can seem daunting, but with a clear understanding of the process, it becomes straightforward. This article will thoroughly explore the division of the fraction 1/6 by 3, providing a step-by-step explanation, various approaches, real-world applications, and further exercises to solidify your understanding. We'll also delve into the underlying mathematical principles and address common misconceptions.
Understanding Fraction Division
Before tackling 1/6 divided by 3, let's review the fundamental concept of dividing fractions. When we divide a fraction by a whole number, we're essentially asking: "How many times does the whole number fit into the fraction?" This differs from multiplying fractions, where we are finding a portion of a portion.
The key to dividing fractions is to remember the reciprocal. The reciprocal of a number is simply 1 divided by that number. For example, the reciprocal of 3 is 1/3. The reciprocal of 2/5 is 5/2.
To divide a fraction by a whole number, we follow these steps:
-
Convert the whole number into a fraction: Any whole number can be expressed as a fraction with a denominator of 1. For instance, 3 can be written as 3/1.
-
Invert the second fraction (find its reciprocal): Invert the fraction you are dividing by (the divisor). So, the reciprocal of 3/1 is 1/3.
-
Multiply the first fraction by the reciprocal of the second fraction: This is the crucial step in fraction division. We change the division problem into a multiplication problem.
-
Simplify the resulting fraction (if possible): Reduce the fraction to its lowest terms.
Solving 1/6 Divided by 3
Now, let's apply these steps to solve 1/6 divided by 3:
-
Convert 3 to a fraction: 3 becomes 3/1.
-
Find the reciprocal of 3/1: The reciprocal of 3/1 is 1/3.
-
Multiply 1/6 by 1/3: (1/6) * (1/3) = 1/18
-
Simplify: The fraction 1/18 is already in its simplest form, as 1 and 18 share no common factors other than 1.
Therefore, 1/6 divided by 3 equals 1/18.
Alternative Approaches and Visualizations
While the reciprocal method is the most efficient way to divide fractions, visualizing the problem can improve understanding, especially for beginners.
Visual Representation using a Pie Chart
Imagine a pie cut into six equal slices. 1/6 represents one slice of this pie. Dividing this 1/6 slice by 3 means dividing that single slice into three even smaller pieces. Each of these smaller pieces represents 1/18 of the whole pie.
Using Repeated Subtraction
We can also approach the problem using repeated subtraction. How many times can we subtract 3 from 1/6? This approach is less efficient for fractions but can be helpful in grasping the concept of division. Since 3 is larger than 1/6, we cannot subtract 3 from 1/6 any whole number of times. The result reflects this fact; we end up with a very small fraction, 1/18, indicating that 3 fits into 1/6 a tiny fraction of a time.
Real-World Applications
Understanding fraction division is crucial in various real-world scenarios:
-
Cooking and Baking: If a recipe calls for 1/6 cup of sugar and you want to reduce the recipe by a factor of 3, you would need to divide 1/6 by 3 to find the new amount of sugar needed.
-
Sewing and Crafts: When working with fabric or other materials, you may need to divide fractional lengths or quantities.
-
Construction and Engineering: Accurate measurements are paramount in construction and engineering. Division of fractions helps in scaling down blueprints or calculating material requirements.
-
Data Analysis: In data analysis and statistics, dealing with fractions and their division is commonplace for various calculations.
-
Finance: Dividing fractions is vital in calculating portions of investments or debts.
Common Mistakes and Misconceptions
Here are some common errors to avoid when dividing fractions:
-
Forgetting the reciprocal: Remember to invert (find the reciprocal) the second fraction before multiplying. Simply multiplying the fractions without inverting will yield an incorrect answer.
-
Incorrect simplification: Always simplify the resulting fraction to its lowest terms. Leaving the fraction unsimplified can lead to misunderstandings.
-
Confusing division with multiplication: Dividing fractions is distinct from multiplying them. Remember the crucial step of inverting the divisor before multiplying.
-
Dividing numerators and denominators directly: This only works when dividing fractions by fractions; this does not apply to dividing a fraction by a whole number.
Practice Problems
To solidify your understanding, try solving these problems:
- 1/8 divided by 4
- 2/5 divided by 2
- 3/4 divided by 6
- 5/12 divided by 5
Conclusion
Dividing fractions, particularly a fraction by a whole number, is a fundamental skill with wide-ranging applications. By understanding the concept of the reciprocal and carefully following the steps outlined in this guide, you can confidently tackle these types of problems. Remember to visualize the problem when necessary to enhance your comprehension. Regular practice is key to mastering fraction division, and tackling various problems will build your proficiency and problem-solving skills. Don't hesitate to review these steps and explore the provided practice problems to ensure you have a complete grasp of this important mathematical operation. Through consistent practice and a thorough understanding of the principles involved, you can confidently navigate the world of fraction division and apply your knowledge to real-world situations.
Latest Posts
Latest Posts
-
What Is Four To The Third Power
May 12, 2025
-
Animal Jam What Is A Party Hat Worth
May 12, 2025
-
How Many Rhode Islands Can Fit In Texas
May 12, 2025
-
How Tall Is 176 Cm In Height
May 12, 2025
-
How To Say Do You Miss Me In Spanish
May 12, 2025
Related Post
Thank you for visiting our website which covers about 1/6 Divided By 3 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.