155 Out Of 200 As A Percentage
Arias News
May 10, 2025 · 4 min read
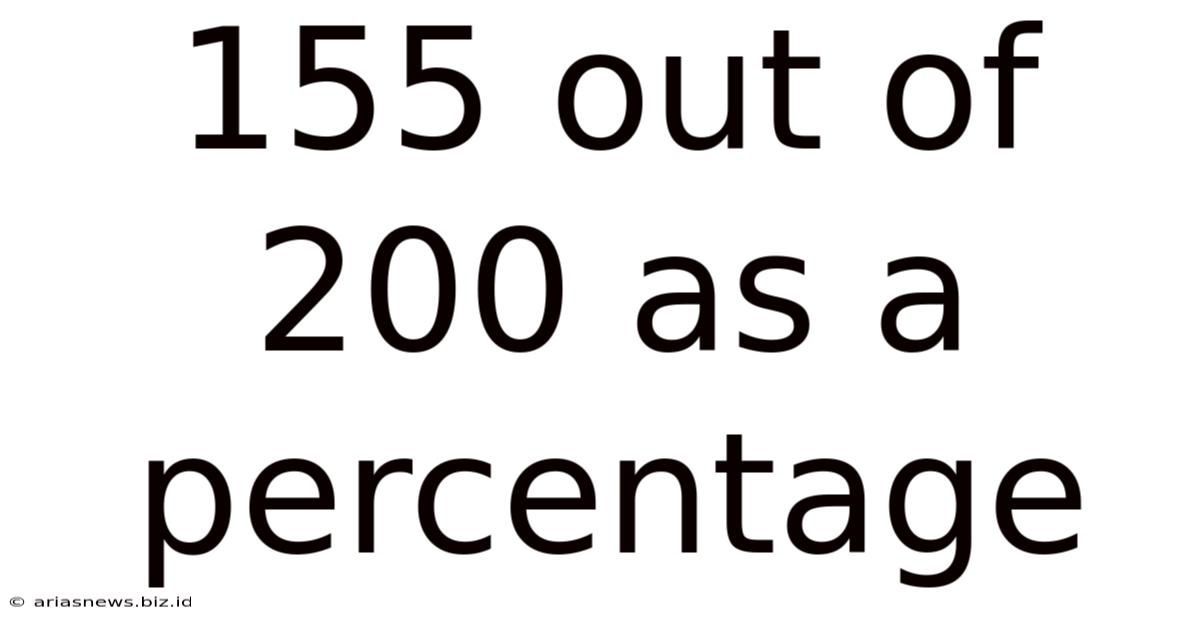
Table of Contents
155 out of 200 as a Percentage: A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with broad applications across various fields. Understanding how to calculate percentages is crucial for everyday life, from calculating discounts and tax to analyzing data and understanding statistics. This article delves into the calculation of 155 out of 200 as a percentage, providing a detailed explanation, different methods, and practical applications.
Understanding Percentages
A percentage is a fraction or a ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred." It's represented by the symbol "%". Percentages are used to express proportions, making it easier to compare and understand different quantities. For example, 50% means 50 out of 100, which is equivalent to ½ or 0.5.
Calculating 155 out of 200 as a Percentage
To calculate 155 out of 200 as a percentage, we can use the following formula:
(Part / Whole) * 100%
In this case:
- Part: 155
- Whole: 200
Substituting these values into the formula, we get:
(155 / 200) * 100% = 0.775 * 100% = 77.5%
Therefore, 155 out of 200 is 77.5%.
Different Methods for Calculation
While the above method is the most straightforward, let's explore other approaches to calculate this percentage:
Method 1: Using a Calculator
Most calculators have a percentage function. Simply input "155 ÷ 200 =" and then multiply the result by 100. This will directly give you the percentage.
Method 2: Simplifying the Fraction
Before multiplying by 100%, you can simplify the fraction 155/200. Both numbers are divisible by 5:
155 ÷ 5 = 31 200 ÷ 5 = 40
This simplifies the fraction to 31/40. Now, calculate (31/40) * 100% = 77.5%
This method can be helpful when dealing with larger numbers, making the calculation easier.
Method 3: Using Proportions
You can set up a proportion to solve for the percentage:
155/200 = x/100
Cross-multiply:
155 * 100 = 200 * x 15500 = 200x x = 15500 / 200 x = 77.5
Therefore, x = 77.5%, confirming our earlier result.
Practical Applications of Percentage Calculations
The ability to calculate percentages has numerous practical applications in everyday life and various professional fields. Here are a few examples:
1. Discounts and Sales
Retail stores frequently offer discounts on products. If a product is marked down by 20%, you'd need to calculate the final price after the discount. Understanding percentages helps you quickly determine the savings.
2. Taxes and Tipping
Calculating sales tax or tipping in restaurants involves percentages. Knowing how to calculate these amounts quickly is essential for managing your finances.
3. Grade Calculations
In education, grades are often expressed as percentages. Calculating your overall grade average requires understanding percentage calculations.
4. Financial Analysis
In finance, percentages are widely used to analyze investments, returns, and financial statements. Understanding percentage changes in stock prices, interest rates, or profits is crucial for making informed financial decisions.
5. Data Analysis and Statistics
Percentages are essential in data analysis and statistics for expressing proportions, trends, and probabilities. For instance, you might use percentages to represent the percentage of people who prefer a particular product or the success rate of a particular treatment.
6. Scientific Research
In scientific research, percentages are used to present experimental results, statistical significance, and error rates.
Further Exploring Percentages: Related Calculations
Understanding the calculation of 155 out of 200 as a percentage opens the door to exploring other related calculations:
-
Finding the percentage increase or decrease: This involves calculating the percentage change between two values. For example, if a stock price increases from $100 to $120, the percentage increase is calculated as ((120-100)/100) * 100% = 20%.
-
Calculating a percentage of a number: This is the reverse calculation of finding a percentage. For example, finding 25% of 200 would be (25/100) * 200 = 50.
-
Working with percentage points: It's crucial to distinguish between percentage change and percentage points. A change from 10% to 15% is a 5 percentage point increase, but a 50% increase in the percentage itself.
-
Compound interest calculations: Compound interest involves calculating interest on both the principal amount and accumulated interest. This requires repeated percentage calculations.
Conclusion: Mastering Percentage Calculations
Mastering the ability to calculate percentages is a valuable skill with applications across numerous aspects of life. Understanding the various methods and their practical applications empowers you to handle everyday financial tasks, analyze data effectively, and make informed decisions in various fields. This comprehensive guide provides a strong foundation for confidently tackling percentage calculations, ensuring you're well-equipped to solve problems involving percentages in your personal and professional life. The example of 155 out of 200, which equates to 77.5%, serves as a clear illustration of these fundamental principles. Remember to practice regularly to build your proficiency and confidence in working with percentages.
Latest Posts
Latest Posts
-
What Is The Hair On An Elephants But Called
May 10, 2025
-
What Is Half Of 2 3 4 Inches
May 10, 2025
-
How To Find The Density Of A Rock
May 10, 2025
-
Do Jeopardy Contestants Get To Keep Their Money
May 10, 2025
-
How Old Are You If Your Born In 1964
May 10, 2025
Related Post
Thank you for visiting our website which covers about 155 Out Of 200 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.