21 Out Of 28 As A Percentage
Arias News
May 10, 2025 · 4 min read
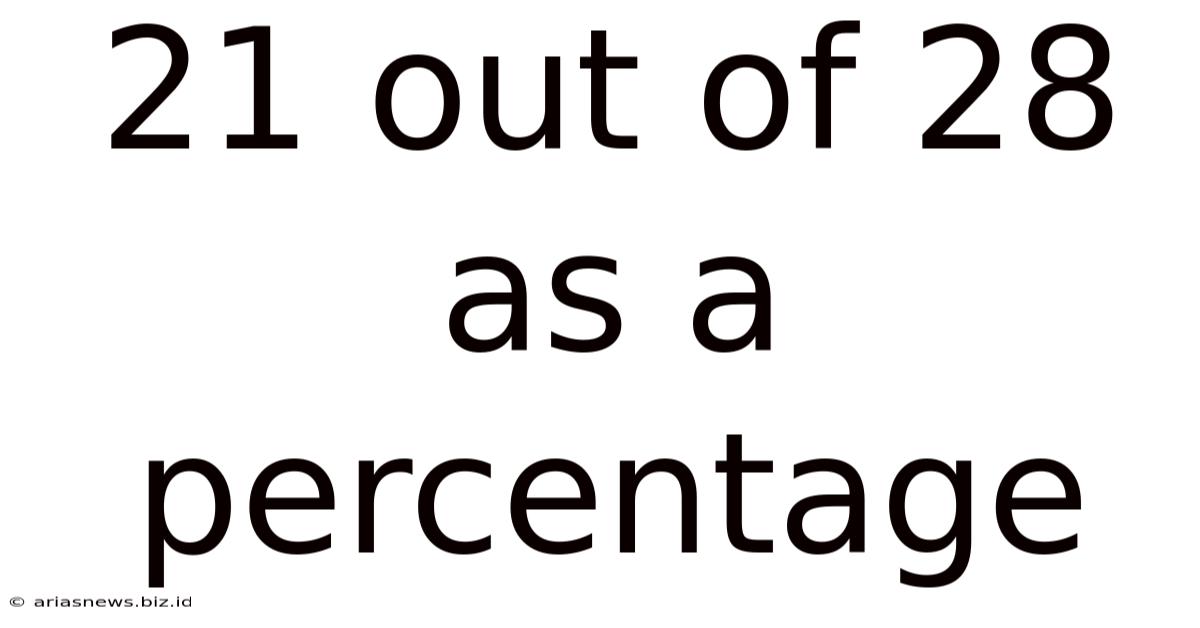
Table of Contents
21 out of 28 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill in many areas of life, from managing finances to understanding statistics. Knowing how to convert fractions into percentages is crucial for interpreting data, making informed decisions, and excelling in various academic and professional fields. This comprehensive guide will delve into the calculation of 21 out of 28 as a percentage, explaining the process in detail and exploring related concepts. We'll cover different methods, address potential confusion, and provide practical examples to solidify your understanding.
Understanding Percentages
Before we jump into the calculation, let's refresh our understanding of percentages. A percentage is simply a fraction expressed as a number out of 100. The word "percent" literally means "out of one hundred." The symbol "%" represents this fraction. For example, 50% means 50 out of 100, which simplifies to 1/2 or 0.5.
Calculating 21 out of 28 as a Percentage: Method 1 (Fraction to Decimal to Percentage)
This is the most common and straightforward method. We'll break it down into three simple steps:
Step 1: Express as a Fraction
The first step is to express the given values as a fraction. "21 out of 28" is written as 21/28.
Step 2: Convert the Fraction to a Decimal
To convert a fraction to a decimal, we divide the numerator (the top number) by the denominator (the bottom number). In this case, we divide 21 by 28:
21 ÷ 28 = 0.75
Step 3: Convert the Decimal to a Percentage
To convert a decimal to a percentage, we multiply the decimal by 100 and add the "%" symbol.
0.75 × 100 = 75%
Therefore, 21 out of 28 is 75%.
Calculating 21 out of 28 as a Percentage: Method 2 (Simplifying the Fraction)
This method involves simplifying the fraction before converting it to a decimal and then a percentage. This can make the calculation easier, especially with larger numbers.
Step 1: Simplify the Fraction
We look for the greatest common divisor (GCD) of 21 and 28. The GCD of 21 and 28 is 7. We divide both the numerator and the denominator by 7:
21 ÷ 7 = 3 28 ÷ 7 = 4
This simplifies the fraction to 3/4.
Step 2: Convert the Simplified Fraction to a Decimal
Now we divide the numerator by the denominator:
3 ÷ 4 = 0.75
Step 3: Convert the Decimal to a Percentage
Finally, we multiply the decimal by 100 and add the "%" symbol:
0.75 × 100 = 75%
Again, we arrive at the answer: 21 out of 28 is 75%.
Understanding the Result: What 75% Means
A score of 75% indicates that out of a total of 28 possible points or items, 21 were achieved or selected. This represents a strong performance, significantly above the average. In many academic and professional settings, a score of 75% or higher is often considered a good result.
Practical Applications of Percentage Calculations
Understanding percentage calculations is essential in various real-life situations, including:
- Academic Performance: Calculating grades, understanding test scores, and assessing progress.
- Financial Management: Calculating interest rates, discounts, taxes, and profits/losses.
- Data Analysis: Interpreting statistical data, creating charts and graphs, and understanding trends.
- Shopping and Sales: Calculating discounts, comparing prices, and understanding sales tax.
- Cooking and Baking: Scaling recipes up or down based on percentages.
Potential Pitfalls and Common Mistakes
While percentage calculations are relatively straightforward, some common mistakes can occur:
- Incorrect Fraction Formation: Ensuring the correct numerator and denominator are used in the initial fraction is crucial.
- Decimal Point Errors: Accuracy in dividing the numerator by the denominator is essential. A misplaced decimal point will lead to an incorrect percentage.
- Misunderstanding Percentage Meaning: Clearly understanding what the percentage represents in the context of the problem is vital for correct interpretation.
Advanced Percentage Calculations: Beyond the Basics
While this article focuses on calculating 21 out of 28 as a percentage, let's briefly touch upon some more advanced percentage calculations:
- Percentage Increase/Decrease: Calculating the percentage change between two values.
- Percentage of a Percentage: Finding a percentage of a percentage (e.g., 20% of 50%).
- Finding the Original Value: Working backward from a percentage to find the original value.
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is a valuable skill that enhances your ability to understand and interpret data in various contexts. By understanding the fundamental methods, such as converting fractions to decimals and then to percentages, and by being aware of potential pitfalls, you can confidently tackle percentage calculations in your daily life, academic pursuits, and professional endeavors. Remember, practice makes perfect! The more you practice these calculations, the more comfortable and proficient you'll become. The ability to quickly and accurately calculate percentages will prove invaluable across numerous aspects of your life.
Latest Posts
Latest Posts
-
Write 2 3 10 As A Decimal Number
May 11, 2025
-
20 X 20 How Many Square Feet
May 11, 2025
-
Provides Both Equilibrium And Aesthetics To A Piece Of Art
May 11, 2025
-
Why Do Lincoln Burrows And Michael Scofield Have Different Surnames
May 11, 2025
-
How Old Was Odysseus In The Odyssey
May 11, 2025
Related Post
Thank you for visiting our website which covers about 21 Out Of 28 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.