271 403 Rounded To The Nearest Ten Thousand
Arias News
Mar 28, 2025 · 5 min read
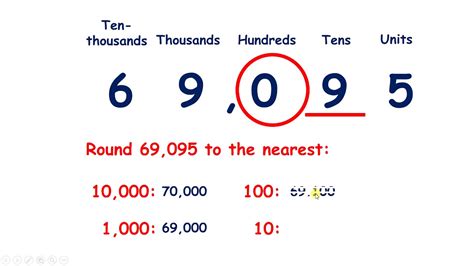
Table of Contents
271,403 Rounded to the Nearest Ten Thousand: A Deep Dive into Rounding and its Applications
Rounding is a fundamental mathematical concept with far-reaching applications in various fields. Understanding how to round numbers accurately is crucial for everyday life, from estimating costs to interpreting statistical data. This article will delve into the process of rounding 271,403 to the nearest ten thousand, exploring the underlying principles and providing practical examples to solidify your understanding. We'll also examine the broader implications of rounding in different contexts, highlighting its importance in various professions and disciplines.
Understanding the Concept of Rounding
Rounding involves approximating a number to a certain place value, simplifying it while retaining a degree of accuracy. The process involves identifying the digit in the place value you're rounding to and then looking at the digit immediately to its right. If the digit to the right is 5 or greater, you round up; if it's less than 5, you round down.
Key Terms:
- Rounding: The process of approximating a number to a specified place value.
- Place Value: The value represented by a digit in a number based on its position (ones, tens, hundreds, thousands, ten thousands, etc.).
- Rounding Up: Increasing the digit in the rounding place value by one.
- Rounding Down: Keeping the digit in the rounding place value the same.
Rounding 271,403 to the Nearest Ten Thousand
Let's apply this knowledge to round 271,403 to the nearest ten thousand.
-
Identify the ten thousands place: In the number 271,403, the digit in the ten thousands place is 7.
-
Look at the digit to the right: The digit immediately to the right of the 7 is 1.
-
Round up or down: Since 1 is less than 5, we round down. This means the 7 in the ten thousands place remains unchanged.
-
Replace digits to the right with zeros: All digits to the right of the ten thousands place become 0.
Therefore, 271,403 rounded to the nearest ten thousand is 270,000.
Practical Applications of Rounding
Rounding is not just an abstract mathematical exercise; it's a practical tool used across many fields:
1. Everyday Estimations:
- Budgeting: When planning a budget, you might round expenses to the nearest ten or hundred dollars to get a quick overview of your spending.
- Shopping: Estimating the total cost of groceries involves rounding individual item prices to simplify calculations.
- Travel: Calculating travel time often involves rounding distances and speeds to make estimations easier.
2. Science and Engineering:
- Measurements: Scientific measurements are often rounded to a specific level of precision based on the instruments used and the desired accuracy.
- Data Analysis: Rounding large datasets simplifies analysis and presentation of results, making it easier to identify trends and patterns.
- Engineering Design: Engineers frequently round measurements in blueprints and designs for practicality and manufacturability.
3. Finance and Accounting:
- Financial Reporting: Financial statements often use rounded figures for clarity and ease of understanding.
- Investment Calculations: Calculating returns on investments may involve rounding for simplification.
- Tax Calculations: Tax calculations frequently involve rounding to the nearest dollar or cent.
4. Statistics and Data Science:
- Data Visualization: Rounding numbers for charts and graphs makes them easier to read and interpret.
- Descriptive Statistics: Summary statistics such as mean, median, and mode are often rounded for presentation.
- Statistical Inference: Rounded data can be used in hypothesis testing and other statistical analyses, although precision should be considered.
Significance of Precision and Accuracy in Rounding
While rounding simplifies numbers, it's crucial to understand that it introduces a degree of inaccuracy. The level of accuracy required depends heavily on the context. Rounding a large sum of money to the nearest thousand dollars for a budget might be acceptable, but rounding the cost of a single item to the nearest dollar for an invoice is inappropriate. The consequences of rounding errors can be significant in various fields, impacting everything from financial transactions to scientific experiments. Therefore, careful consideration of the required precision is paramount.
Advanced Rounding Techniques:
Beyond simple rounding to the nearest ten, hundred, or thousand, more sophisticated rounding techniques exist, including:
- Rounding to significant figures: This method focuses on preserving the accuracy of the most significant digits in a number.
- Rounding half up: This common method rounds a number to the nearest even number when the digit to be rounded is exactly 5. This helps to minimize bias in large datasets.
- Rounding half down: Similar to half up, but rounds to the nearest odd number when the digit is 5.
Error Analysis and Rounding:
Understanding potential errors introduced by rounding is vital for ensuring the reliability of results. In many applications, particularly in scientific and engineering calculations, it is crucial to account for potential rounding errors and propagate them through calculations to assess the overall uncertainty in the final result. Techniques such as error propagation analysis help in quantifying and managing these errors.
Rounding in Different Number Systems:
The principles of rounding extend beyond the decimal number system. Rounding can also be applied to other number systems, such as binary and hexadecimal systems, used extensively in computer science and digital electronics. The underlying concepts remain the same, but the base (radix) changes depending on the system used.
Conclusion: The Importance of Rounding in a Digital Age
In our increasingly data-driven world, the ability to round numbers effectively remains a critical skill. From simple estimations in everyday life to complex calculations in scientific research, rounding serves as a fundamental tool for simplifying numbers while retaining a suitable level of accuracy. A deep understanding of the principles of rounding, its various applications, and potential limitations is essential for anyone working with numbers, regardless of their field of expertise. The example of rounding 271,403 to the nearest ten thousand provides a clear illustration of this fundamental process and its far-reaching implications. By mastering the art of rounding, we enhance our ability to interpret data, make informed decisions, and navigate the complexities of a world awash in numerical information. Remember that precision and context are key when choosing your rounding method. The correct approach will significantly impact the accuracy and reliability of your calculations and analyses.
Latest Posts
Latest Posts
-
How Much Does 5 Gallons Of Concrete Weigh
Mar 31, 2025
-
What Is The Opposite Of An Inverse Relationship
Mar 31, 2025
-
5 Letter Word With Y As Second Letter
Mar 31, 2025
-
How Many Days Is 5 Million Seconds
Mar 31, 2025
-
What Do You Call A Female Bug That Floats
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about 271 403 Rounded To The Nearest Ten Thousand . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
