3 4 Divided By 1 4 In Fraction
Arias News
May 11, 2025 · 5 min read
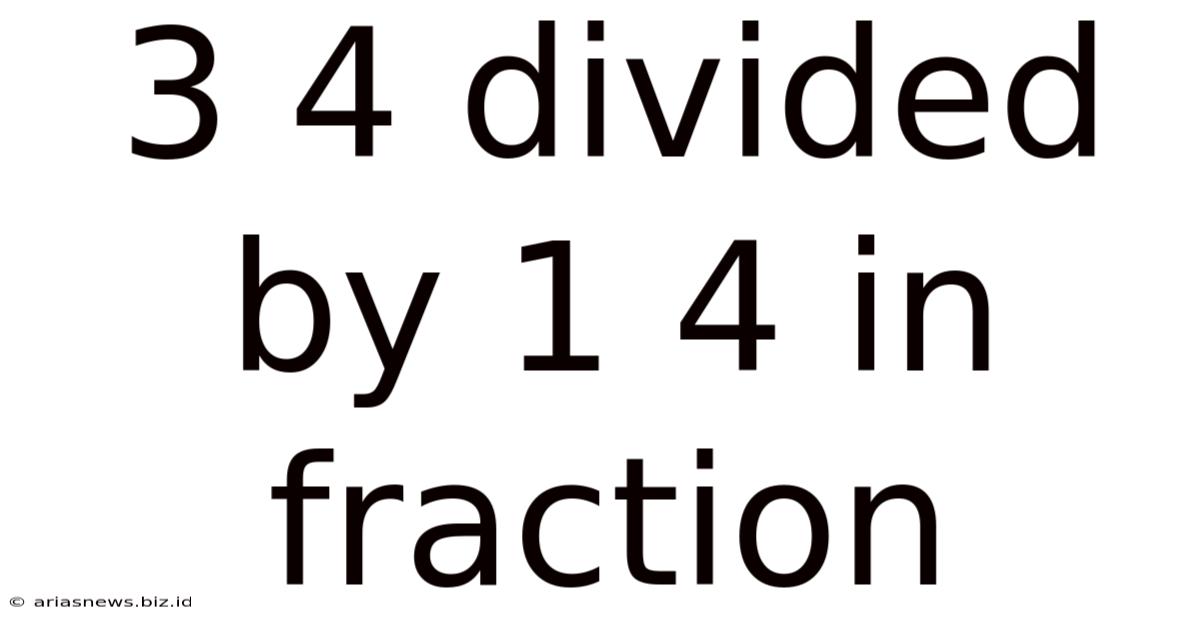
Table of Contents
3/4 Divided by 1/4: A Comprehensive Guide to Fraction Division
Dividing fractions can seem daunting at first, but with a clear understanding of the underlying principles, it becomes a straightforward process. This article delves deep into the division of 3/4 by 1/4, providing a step-by-step explanation, exploring various methods, and offering practical applications to solidify your understanding. We'll also touch upon the broader context of fraction division, ensuring you're equipped to tackle similar problems with confidence.
Understanding Fraction Division
Before diving into the specific problem of 3/4 divided by 1/4, let's establish a foundational understanding of fraction division. The core concept revolves around finding out "how many times" one fraction fits into another. Unlike multiplication, where we find the combined value, division focuses on determining the number of times a fraction is contained within another.
Consider a simple analogy: Imagine you have a pizza cut into 4 equal slices. If you have 3 slices (3/4 of the pizza), and you want to know how many 1/4 slices are in your portion, you're essentially performing the division 3/4 ÷ 1/4.
Method 1: The "Keep, Change, Flip" Method (Reciprocal Method)
This is arguably the most popular and widely used method for dividing fractions. It involves three simple steps:
- Keep: Keep the first fraction (the dividend) exactly as it is: 3/4.
- Change: Change the division sign (÷) to a multiplication sign (×).
- Flip: Flip the second fraction (the divisor) – find its reciprocal. The reciprocal of 1/4 is 4/1 (or simply 4).
Therefore, the problem becomes: 3/4 × 4/1
Now, we multiply the numerators (top numbers) together and the denominators (bottom numbers) together:
(3 × 4) / (4 × 1) = 12/4
Finally, simplify the resulting fraction by dividing both the numerator and the denominator by their greatest common divisor (GCD), which is 4 in this case:
12/4 = 3
Therefore, 3/4 divided by 1/4 equals 3. This means that there are three 1/4 slices within 3/4 of a whole.
Method 2: Visual Representation
A visual approach can enhance understanding, especially for beginners. Imagine a rectangle representing a whole. Divide the rectangle into four equal parts. Shade three of those parts to represent 3/4. Now, consider each of the four parts as a 1/4 slice. By visually inspecting the shaded area (3/4), you can clearly see that it contains three 1/4 slices. This provides a concrete visual confirmation of the answer: 3.
Method 3: Converting to Decimal
Another approach involves converting the fractions to decimals before performing the division.
- 3/4 = 0.75
- 1/4 = 0.25
Now, perform the division: 0.75 ÷ 0.25 = 3
This method provides an alternative way to arrive at the same answer, reinforcing the concept. However, it's crucial to remember that this method can sometimes lead to rounding errors, especially when dealing with fractions that don't convert easily to terminating decimals.
Real-World Applications of Fraction Division
Understanding fraction division isn't just an abstract mathematical exercise; it has practical applications in various aspects of everyday life. Consider these examples:
-
Baking: A recipe calls for 3/4 cup of flour, but you only want to make 1/4 of the recipe. To determine the amount of flour needed, you would divide 3/4 by 4 (which is the same as multiplying by 1/4): 3/4 ÷ 4 = 3/16 cup of flour.
-
Sewing: If you need 3/4 of a yard of fabric and each piece available is 1/4 of a yard, how many pieces do you need? This is simply 3/4 ÷ 1/4 = 3 pieces.
-
Construction: Imagine you're building a wall and need to divide a section of 3/4 meters into pieces of 1/4 meter each. You would divide 3/4 by 1/4 to find out how many pieces you can create: 3/4 ÷ 1/4 = 3 pieces.
-
Time Management: If you have 3/4 of an hour to complete a task, and each sub-task takes 1/4 of an hour, how many sub-tasks can you accomplish? This again is a straightforward division problem: 3/4 ÷ 1/4 = 3 sub-tasks.
Expanding the Concept: Dividing Fractions with Larger Numerators and Denominators
The principles discussed above apply universally to all fraction divisions. Let's consider a more complex example: 15/8 ÷ 5/8.
Using the "Keep, Change, Flip" method:
- Keep: 15/8
- Change: ÷ becomes ×
- Flip: 5/8 becomes 8/5
The problem becomes: 15/8 × 8/5
Multiply the numerators and denominators: (15 × 8) / (8 × 5) = 120/40
Simplify by dividing by the GCD (40): 120/40 = 3
Notice that, even with larger numbers, the process remains the same. The key is to understand the underlying concept and apply the steps systematically.
Dealing with Mixed Numbers
When dealing with mixed numbers (a whole number and a fraction, like 1 1/2), convert them to improper fractions before applying the division method. For instance, if the problem is 1 1/2 ÷ 1/4, first convert 1 1/2 to an improper fraction (3/2). Then, proceed with the "Keep, Change, Flip" method:
3/2 ÷ 1/4 becomes 3/2 × 4/1 = 12/2 = 6.
Conclusion: Mastering Fraction Division
Mastering fraction division is crucial for various mathematical applications and everyday problem-solving. By understanding the fundamental concept, applying the "Keep, Change, Flip" method (or the visual method), and practicing regularly, you can build confidence and proficiency in this essential skill. Remember that the key lies not just in memorizing the steps, but in understanding why these steps work. The more you practice and explore different approaches, the more intuitive and effortless fraction division will become. Remember to always simplify your final answer to its lowest terms for the clearest and most concise representation.
Latest Posts
Latest Posts
-
How Many Ounces Of Fish In A Pound
May 11, 2025
-
What Is Common On A Light Switch
May 11, 2025
-
What Is 175 Degrees Fahrenheit On The Celsius Scale
May 11, 2025
-
All Of The Following Are Benefits Of Reflective Listening Except
May 11, 2025
-
How Much Is 156 Pounds In Kg
May 11, 2025
Related Post
Thank you for visiting our website which covers about 3 4 Divided By 1 4 In Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.