30 Out Of 350 As A Percentage
Arias News
May 08, 2025 · 5 min read
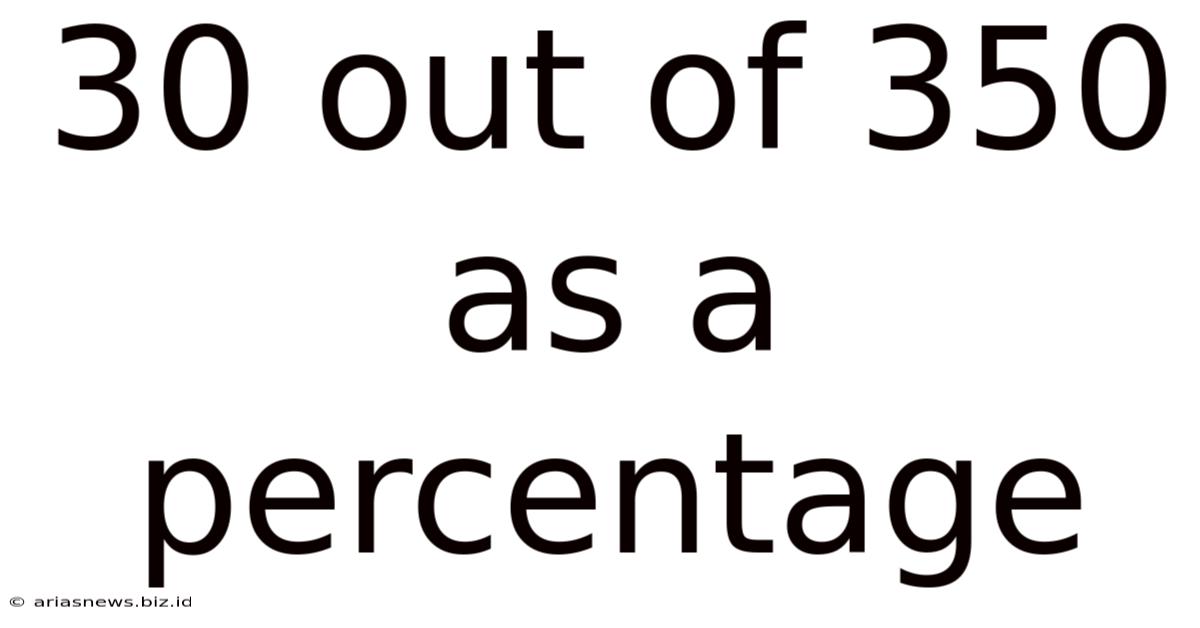
Table of Contents
30 Out of 350 as a Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill applicable across numerous fields, from academic pursuits to financial planning and everyday life. Understanding how to express a portion of a whole as a percentage is crucial for interpreting data, making comparisons, and understanding proportions. This article delves into the calculation of 30 out of 350 as a percentage, providing a step-by-step guide, exploring different calculation methods, and offering practical applications and real-world examples.
Understanding Percentages
Before we delve into the specific calculation, let's establish a solid understanding of percentages. A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For instance, 50% means 50 out of 100, which is equivalent to ½ or 0.5.
Calculating 30 out of 350 as a Percentage: The Basic Method
The most straightforward method for calculating 30 out of 350 as a percentage involves these steps:
Step 1: Set up the Fraction
First, represent the given information as a fraction. The number representing the part (30) becomes the numerator, and the number representing the whole (350) becomes the denominator:
30/350
Step 2: Convert the Fraction to a Decimal
Divide the numerator (30) by the denominator (350):
30 ÷ 350 ≈ 0.0857
Step 3: Convert the Decimal to a Percentage
Multiply the decimal result by 100 to express it as a percentage:
0.0857 × 100 ≈ 8.57%
Therefore, 30 out of 350 is approximately 8.57%.
Alternative Calculation Methods
While the basic method is perfectly adequate, let's explore alternative approaches that might be useful in different contexts or with different levels of mathematical comfort:
Method 2: Using Proportions
This method utilizes the concept of proportions to solve the problem. We can set up a proportion:
x/100 = 30/350
To solve for x (the percentage), cross-multiply:
350x = 3000
Divide both sides by 350:
x = 3000/350 ≈ 8.57
Therefore, x ≈ 8.57%, which confirms our previous result.
Method 3: Simplifying the Fraction First
Before converting to a decimal, we can simplify the fraction 30/350. Both the numerator and the denominator are divisible by 10:
30/350 = 3/35
Now, divide 3 by 35:
3 ÷ 35 ≈ 0.0857
Multiply by 100 to obtain the percentage:
0.0857 × 100 ≈ 8.57%
This method can be particularly helpful when dealing with larger numbers, as simplifying the fraction first can make the subsequent calculations easier.
Understanding the Result: 8.57%
The result, 8.57%, signifies that 30 represents approximately 8.57% of 350. This means that if you divide 350 into 100 equal parts, 30 would occupy approximately 8.57 of those parts.
Practical Applications and Real-World Examples
The ability to calculate percentages is invaluable in various real-world scenarios. Here are a few examples:
1. Academic Performance:
Imagine a student scored 30 out of 350 marks on an exam. Calculating the percentage (8.57%) provides a clear picture of their performance relative to the total marks.
2. Business and Finance:
In business, calculating percentages is crucial for analyzing sales figures, profit margins, and market share. For example, if a company sold 30 units out of a total production of 350 units, the sales percentage would be 8.57%.
3. Statistics and Data Analysis:
Percentages are essential in statistics for representing proportions within datasets. For instance, if 30 out of 350 respondents in a survey answered "yes" to a particular question, the percentage of "yes" responses would be 8.57%.
4. Everyday Life:
Calculating percentages is also relevant in everyday situations such as calculating discounts, tips, or interest rates. For example, an 8.57% discount on a $350 item would amount to a saving of approximately $30.
Rounding and Precision
In many situations, it’s acceptable to round the percentage to a simpler value. For instance, 8.57% could be rounded to 9% for easier comprehension. However, the level of precision required depends on the context. In financial calculations, higher precision might be necessary, while in everyday scenarios, rounding might be sufficient.
Advanced Percentage Calculations and Concepts
While calculating 30 out of 350 is a basic percentage problem, understanding more advanced concepts can broaden your application of percentages:
1. Percentage Increase and Decrease:
These concepts describe the relative change between two values. For instance, if a value increases from 300 to 350, the percentage increase is calculated as [(350-300)/300] * 100% = 16.67%.
2. Compound Interest:
Compound interest involves calculating interest not only on the principal amount but also on accumulated interest. Understanding compound interest is crucial in various financial contexts, including investments and loans.
3. Percentage Points vs. Percentages:
It's crucial to differentiate between percentage points and percentages. A change from 10% to 15% is a 5 percentage point increase, not a 50% increase (which would be [(15-10)/10]*100% = 50%).
Conclusion: Mastering Percentage Calculations
The ability to calculate percentages accurately and efficiently is a valuable skill that extends beyond the confines of mathematics classrooms. Understanding the various methods for percentage calculation, as illustrated through the example of 30 out of 350, empowers you to interpret data effectively, solve real-world problems, and make informed decisions across numerous facets of life. From academic performance to financial planning and data analysis, mastery of percentages is an asset that enhances your comprehension and analytical capabilities. Remember to select the calculation method that best suits your needs and to consider the appropriate level of precision for your specific context.
Latest Posts
Latest Posts
-
The Rhetorical Technique Used In This Excerpt Is
May 08, 2025
-
A White Transverse Line Across Your Lane Means
May 08, 2025
-
How Much Ground Cloves Equals One Whole Clove
May 08, 2025
-
How Many Miles An Hour Is 80 Km
May 08, 2025
-
How Many Grams Are In 160 Lbs
May 08, 2025
Related Post
Thank you for visiting our website which covers about 30 Out Of 350 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.