31 Out Of 45 As A Percentage
Arias News
May 12, 2025 · 5 min read
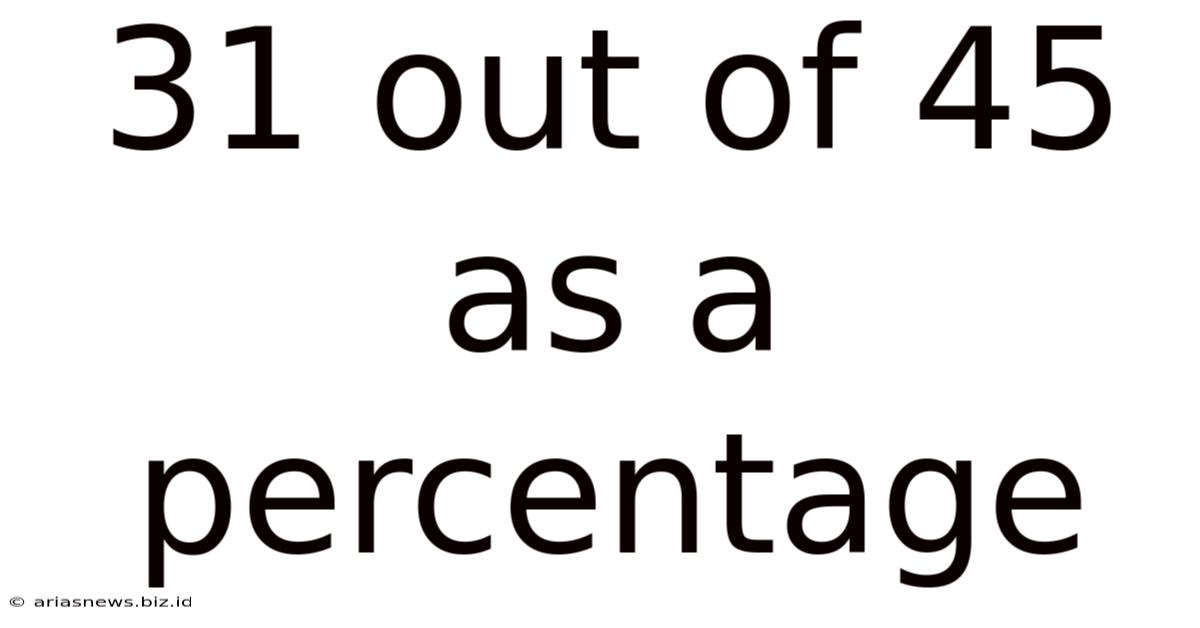
Table of Contents
31 out of 45 as a Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill applicable across numerous fields, from academic studies and financial planning to everyday decision-making. Understanding how to convert fractions and ratios into percentages is crucial for interpreting data, making comparisons, and drawing meaningful conclusions. This comprehensive guide will delve into the process of calculating "31 out of 45 as a percentage," providing step-by-step instructions, real-world examples, and helpful tips to master this essential skill. We'll also explore various methods and tools available to aid in these calculations.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "per hundred," and it's denoted by the symbol "%". For instance, 50% signifies 50 out of 100, which is equivalent to the fraction ½ or the decimal 0.5. Understanding this basic concept is crucial to tackling any percentage calculation.
Calculating 31 out of 45 as a Percentage: The Fundamental Method
The most straightforward method to calculate 31 out of 45 as a percentage involves the following steps:
Step 1: Express the Ratio as a Fraction
First, express the given information as a fraction. In this case, 31 out of 45 is written as 31/45.
Step 2: Convert the Fraction to a Decimal
Divide the numerator (31) by the denominator (45):
31 ÷ 45 ≈ 0.6889
Step 3: Convert the Decimal to a Percentage
Multiply the decimal result by 100:
0.6889 x 100 = 68.89%
Therefore, 31 out of 45 is approximately 68.89%. The slight variation from the exact answer is due to rounding the decimal during the calculation.
Alternative Methods for Percentage Calculation
While the fundamental method is clear and concise, other methods can be employed, particularly when dealing with more complex scenarios or when using calculators or software.
Method 2: Using a Calculator
Most calculators have a percentage function. Simply input "31 ÷ 45 =" and then multiply by 100. This eliminates the manual steps of division and multiplication.
Method 3: Using Online Percentage Calculators
Numerous websites provide free online percentage calculators. These tools are especially useful for complex calculations or when you need to perform multiple percentage calculations. Just input the values and the calculator will automatically provide the percentage.
Method 4: Proportional Reasoning
For those comfortable with ratios and proportions, you can set up a proportion to solve this problem.
Let x represent the percentage:
31/45 = x/100
Cross-multiply:
45x = 3100
Solve for x:
x = 3100/45 ≈ 68.89%
Real-World Applications of Percentage Calculations
Understanding how to calculate percentages is crucial in various real-world scenarios:
- Academic Performance: Calculating grades, test scores, and overall academic standing frequently involves percentage calculations.
- Financial Planning: Calculating interest rates, tax rates, discounts, profits, and losses all rely on percentage calculations. Budgeting and investment strategies heavily depend on accurately understanding and manipulating percentages.
- Business and Sales: Analyzing sales figures, market share, and profit margins requires proficiency in percentage calculations. Pricing strategies and promotional discounts also utilize percentage calculations extensively.
- Healthcare: Calculating medication dosages, monitoring vital signs, and interpreting medical data often involve percentages.
- Everyday Life: Determining tips, sales tax, discounts at stores, and calculating recipe scaling all involve percentage calculations.
Dealing with Rounding and Precision
In many percentage calculations, the result is a decimal number. The level of precision needed depends on the context. For instance:
- Financial calculations: High precision is generally required, often rounding to two decimal places (e.g., 68.89%).
- Academic grading: Precision depends on the grading scale used; sometimes rounding to the nearest whole number is sufficient.
- Everyday estimations: Rounding might be acceptable for rough estimations.
Always consider the context of the calculation to determine the appropriate level of precision and rounding.
Advanced Percentage Calculations: Percentage Increase and Decrease
Beyond calculating a simple percentage, you might need to calculate percentage increase or decrease. Let's consider an example:
Scenario: A product initially cost $50 and its price increased to $65. What is the percentage increase?
Calculation:
- Find the difference: $65 - $50 = $15
- Divide the difference by the original value: $15/$50 = 0.3
- Multiply by 100 to express as a percentage: 0.3 x 100 = 30%
Therefore, the price increased by 30%.
Similarly, you can calculate percentage decrease using the same method, replacing "increase" with "decrease" in the interpretation.
Troubleshooting Common Mistakes in Percentage Calculations
Common mistakes in percentage calculations include:
- Incorrect order of operations: Always follow the order of operations (PEMDAS/BODMAS) to avoid errors in calculations.
- Confusing numerator and denominator: Double-check to ensure that you're using the correct numbers as the numerator and denominator.
- Rounding errors: Be mindful of the level of precision required and apply appropriate rounding techniques.
- Incorrect use of percentage symbols: Ensure you consistently use the percentage symbol (%) where appropriate.
Mastering Percentage Calculations: Tips and Practice
To master percentage calculations, consistent practice is key. Here are a few tips:
- Start with simple problems: Begin with easy percentage calculations and gradually move on to more complex ones.
- Use various methods: Try different methods (calculator, manual calculations, online tools) to improve your understanding and efficiency.
- Practice regularly: Consistent practice reinforces your understanding and improves your accuracy.
- Solve real-world problems: Applying percentage calculations to real-life situations helps solidify your understanding and demonstrates their practicality.
- Seek help when needed: Don't hesitate to ask for clarification if you're struggling with any aspects of the calculation.
Conclusion
Calculating percentages is a crucial skill applicable in numerous fields. Understanding the fundamental method, exploring alternative approaches, and practicing regularly will significantly enhance your proficiency in handling percentages. By mastering this skill, you can confidently tackle a wide range of problems and interpret data more effectively. Remember to always double-check your work and consider the level of precision required for the specific context of your calculations. With consistent practice and attention to detail, you'll become adept at handling percentages efficiently and accurately.
Latest Posts
Related Post
Thank you for visiting our website which covers about 31 Out Of 45 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.