36 Out Of 44 As A Percentage
Arias News
May 10, 2025 · 5 min read
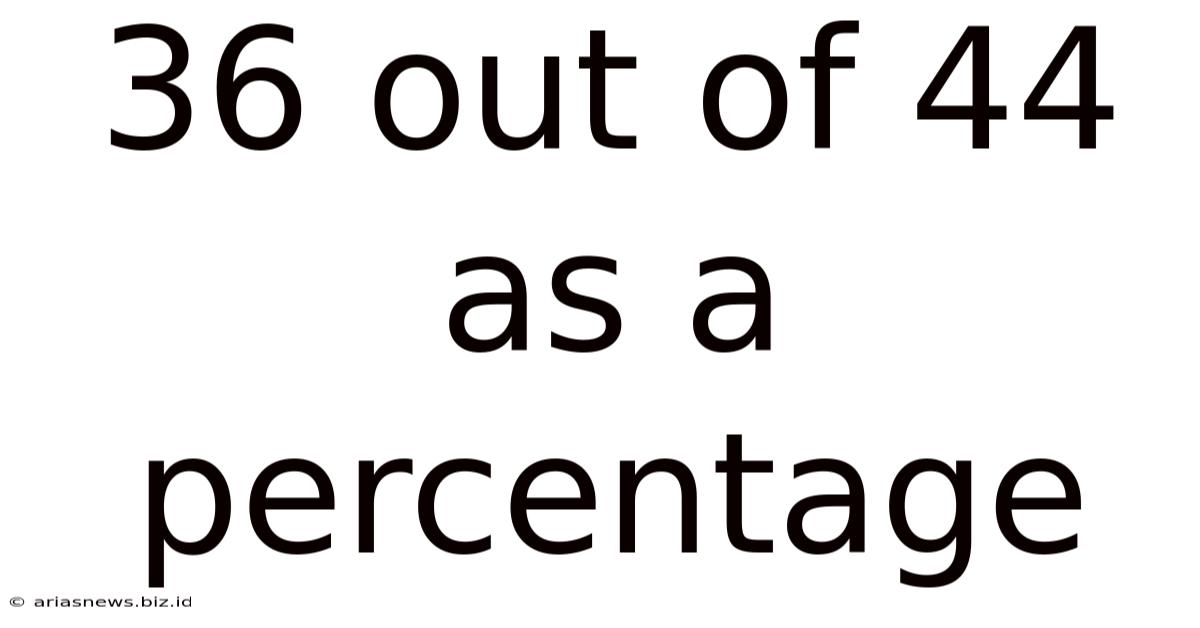
Table of Contents
36 out of 44 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill applicable across numerous fields, from academic studies to professional finance. Understanding how to convert fractions into percentages is crucial for interpreting data, making informed decisions, and communicating effectively. This in-depth guide will not only show you how to calculate "36 out of 44 as a percentage" but also equip you with the knowledge to tackle similar percentage problems with confidence.
Understanding Percentages
Before diving into the calculation, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For instance, 50% signifies 50 out of 100, which is equivalent to ½ or 0.5.
Calculating 36 out of 44 as a Percentage
There are two primary methods to determine the percentage representation of 36 out of 44:
Method 1: Using the Fraction Method
This method involves converting the given numbers into a fraction and then converting that fraction into a percentage.
-
Form a Fraction: Express "36 out of 44" as a fraction: 36/44.
-
Simplify the Fraction (Optional): Simplifying the fraction makes the subsequent calculation easier. Both 36 and 44 are divisible by 4. Therefore, 36/44 simplifies to 9/11.
-
Convert to Decimal: Divide the numerator (9) by the denominator (11): 9 ÷ 11 ≈ 0.818181...
-
Convert Decimal to Percentage: Multiply the decimal by 100: 0.818181... × 100 ≈ 81.82%.
Therefore, 36 out of 44 is approximately 81.82%.
Method 2: Using the Proportion Method
This method uses a proportion to directly calculate the percentage.
-
Set up a Proportion: We want to find x, where x/100 represents the percentage. The proportion is set up as follows:
36/44 = x/100
-
Cross-Multiply: Cross-multiply to solve for x:
44x = 3600
-
Solve for x: Divide both sides by 44:
x = 3600 ÷ 44 ≈ 81.82
Therefore, 36 out of 44 is approximately 81.82%.
Practical Applications of Percentage Calculations
Understanding percentage calculations isn't confined to mathematical exercises; it has far-reaching practical applications in numerous real-world scenarios:
1. Academic Performance:
Calculating grades, especially in a system where points or marks are awarded for various assignments, tests and exams, invariably involves percentages. For example, if a student scores 36 out of 44 marks on a test, their percentage score would be 81.82%, providing a clear indication of their performance. This allows for easy comparison of performance across different subjects and tests.
2. Financial Calculations:
Percentage calculations are integral in various financial domains, including:
- Interest Rates: Banks and financial institutions use percentages to express interest rates on loans and savings accounts. Understanding these percentages is crucial for making informed financial decisions.
- Discounts and Sales: Businesses frequently use percentages to advertise discounts and sales. A "20% off" sale means a reduction of 20% of the original price.
- Profit and Loss Calculations: Determining profit or loss margins requires calculating percentages. The profit margin shows the percentage of revenue that is profit after deducting costs. A high profit margin is generally desired.
- Tax Calculations: Calculating taxes often involves percentage calculations. Sales tax, income tax, and other taxes are typically expressed as a percentage of the taxable amount.
- Investment Returns: Investors use percentages to track the returns on their investments. The percentage return helps evaluate investment performance over time.
3. Data Analysis and Statistics:
Percentages are essential tools for data interpretation and analysis. They are used to represent proportions within a dataset, enabling easy understanding and comparison of different groups or categories within the data. This is especially vital in surveys, polls, scientific research, and market research.
4. Everyday Life:
Beyond formal contexts, percentage calculations are commonly encountered in everyday life, such as:
- Tip Calculations: Calculating tips in restaurants usually involves determining a certain percentage of the total bill.
- Recipe Scaling: Adjusting recipe quantities involves using percentages. For instance, increasing a recipe by 50% means multiplying all ingredients by 1.5.
- Sales Tax: Calculating sales tax on purchases often involves determining a percentage of the total cost.
Avoiding Common Mistakes in Percentage Calculations
While seemingly straightforward, percentage calculations can be prone to errors if not approached carefully. Here are some common mistakes to avoid:
- Incorrect Fraction Formation: Ensure the correct fraction is formed. "36 out of 44" should always be expressed as 36/44, not 44/36.
- Rounding Errors: When rounding off decimal values, avoid premature rounding. Round off only at the final stage to maintain accuracy.
- Incorrect Multiplication/Division: Double-check your calculations to avoid simple arithmetic errors. Using a calculator can help minimise these mistakes.
- Misinterpretation of Percentages: Always clearly understand what the percentage represents. For example, a 20% increase is different from a 20% decrease.
Advanced Percentage Calculations
This section explores more complex percentage calculations that build upon the fundamental understanding discussed earlier:
1. Percentage Increase/Decrease:
Calculating percentage increase or decrease involves finding the difference between two values and then expressing that difference as a percentage of the original value.
For example, if a value increases from 40 to 50, the percentage increase is calculated as follows:
- Find the difference: 50 - 40 = 10
- Divide the difference by the original value: 10 / 40 = 0.25
- Multiply by 100 to express as a percentage: 0.25 * 100 = 25%
Therefore, there's a 25% increase. The same principle applies to percentage decrease calculations.
2. Percentage Change:
Percentage change is a broader term encompassing both percentage increases and decreases. It shows the relative change between two values. The formula is:
[(New Value - Old Value) / Old Value] x 100
3. Percentage of a Percentage:
Calculating a percentage of a percentage involves multiplying the percentages together and then dividing by 100. For example, finding 15% of 20% would be (15/100) * (20/100) * 100 = 3%.
Conclusion
Understanding how to calculate percentages, particularly problems like "36 out of 44 as a percentage," is a valuable skill with numerous practical applications across various aspects of life. By mastering the fundamental methods and avoiding common errors, you can confidently tackle percentage calculations and apply this knowledge to interpret data, make informed decisions, and effectively communicate information. Remember the importance of accuracy and careful execution, particularly when dealing with financial or scientific applications. Consistent practice and a clear understanding of the underlying concepts will lead to mastery of this essential mathematical skill.
Latest Posts
Latest Posts
-
What Is 60 As A Letter Grade
May 11, 2025
-
When Did Taylor Swift Learn To Play Guitar
May 11, 2025
-
How Much Kg Does A Car Weigh
May 11, 2025
-
Is The Square Root Of 19 Rational
May 11, 2025
-
Do Little People Have Normal Sized Genitals
May 11, 2025
Related Post
Thank you for visiting our website which covers about 36 Out Of 44 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.