5 Divided By 3 In A Fraction
Arias News
May 08, 2025 · 5 min read
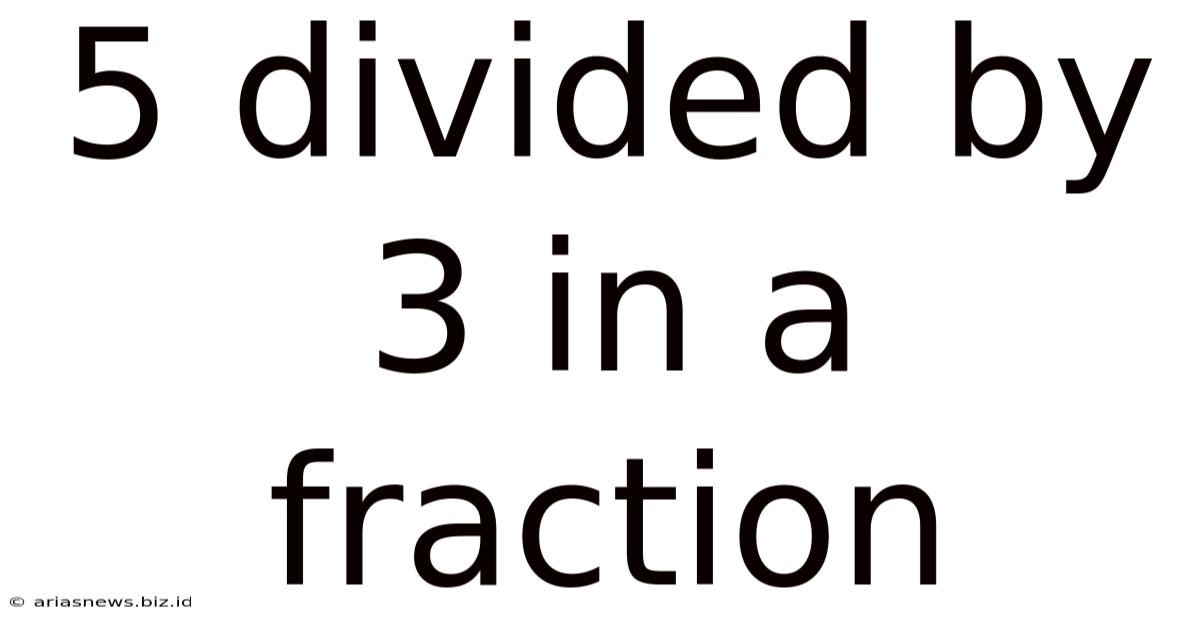
Table of Contents
5 Divided by 3 in a Fraction: A Comprehensive Guide
Dividing 5 by 3 might seem simple at first glance, but understanding the underlying principles and representing the result as a fraction reveals a deeper understanding of fundamental mathematical concepts. This comprehensive guide will explore the different ways to express 5 divided by 3 as a fraction, including its mixed number form, and delve into the practical applications and significance of this seemingly basic calculation.
Understanding the Division Process
Before we jump into expressing 5 divided by 3 as a fraction, let's refresh our understanding of division. Division is essentially the process of splitting a quantity into equal parts. In this case, we're splitting 5 units into 3 equal parts. This cannot be done evenly, resulting in a remainder. This remainder is crucial to our understanding of the fractional representation.
The Long Division Method
Using long division, we find:
1
3 | 5
3
-
2
This shows that 3 goes into 5 one time with a remainder of 2. This remainder represents the portion of the whole that isn't fully divided.
Representing 5 Divided by 3 as a Fraction
The result of 5 divided by 3 can be represented as an improper fraction and a mixed number.
Improper Fraction
An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). In our case, the division problem 5 ÷ 3 is directly represented as the fraction:
5/3
This fraction accurately represents the result of the division: five thirds. It means we have five parts, where each part is one-third of the whole.
Mixed Number
A mixed number combines a whole number and a proper fraction. To convert the improper fraction 5/3 into a mixed number, we perform the division we did with long division:
- Divide the numerator (5) by the denominator (3): 5 ÷ 3 = 1 with a remainder of 2.
- The quotient (1) becomes the whole number part of the mixed number.
- The remainder (2) becomes the numerator of the proper fraction, and the denominator remains the same (3).
Therefore, 5/3 as a mixed number is:
1 2/3
This means we have one whole unit and two-thirds of another unit. Both 5/3 and 1 2/3 represent the same quantity; they are just expressed differently.
Practical Applications of 5/3 and 1 2/3
Understanding how to represent 5 divided by 3 as a fraction has several practical applications across various fields:
Measurement and Units
Imagine you have a rope that's 5 meters long, and you need to cut it into 3 equal pieces. Each piece will be 5/3 meters long, or 1 2/3 meters long. This demonstrates the real-world application of fractional representation in measurement.
Baking and Cooking
Recipes often require fractional measurements. If a recipe calls for 5 cups of flour and you want to reduce the recipe by a factor of 3, you would need to use 5/3 cups of flour, or 1 2/3 cups.
Sharing Resources
If you have 5 pizzas to share equally among 3 people, each person would receive 5/3 pizzas, or 1 2/3 pizzas each. This clarifies the concept of sharing unevenly divisible quantities.
Further Exploration of Fractions
Understanding 5/3 as a fraction opens doors to more complex fractional concepts:
Equivalent Fractions
Multiple fractions can represent the same value. For instance, 5/3 is equivalent to 10/6, 15/9, and so on. These are found by multiplying both the numerator and the denominator by the same number.
Simplifying Fractions
While 5/3 is already in its simplest form (the greatest common divisor of 5 and 3 is 1), other fractions can be simplified by dividing both the numerator and the denominator by their greatest common divisor.
Adding and Subtracting Fractions
Working with fractions often involves adding and subtracting them. To do so, they must have a common denominator. For example, adding 5/3 and 1/3 results in 6/3, which simplifies to 2.
Multiplying and Dividing Fractions
Multiplying fractions involves multiplying the numerators together and the denominators together. Dividing fractions involves inverting the second fraction and then multiplying.
The Significance of Understanding Fractions
The seemingly simple calculation of 5 divided by 3 highlights the importance of a solid grasp of fractional arithmetic. This understanding is fundamental to:
- Problem-solving: Many real-world problems require fractional representation and manipulation to find solutions.
- Algebra: Fractions are fundamental to algebraic equations and manipulations.
- Calculus: Fractional concepts form the basis of more advanced mathematical concepts in calculus.
- Data analysis: Fractions and percentages, which are closely related, are crucial for interpreting and analyzing data.
Conclusion
Representing 5 divided by 3 as a fraction – whether as the improper fraction 5/3 or the mixed number 1 2/3 – is more than just a simple arithmetic calculation. It underscores the importance of understanding fractional concepts, which are building blocks for more advanced mathematical studies and practical applications in various fields. Mastering the representation and manipulation of fractions is essential for success in numerous academic and professional endeavors. Through a thorough understanding of the division process, the different ways to express the result, and the practical applications, we gain a deeper appreciation of this fundamental mathematical concept. The seemingly simple act of dividing 5 by 3 opens a world of mathematical possibilities.
Latest Posts
Latest Posts
-
Half A Gallon Of Water In Oz
May 08, 2025
-
What Is The Square Root Of 185
May 08, 2025
-
How Much Do People On Naked And Afraid Make
May 08, 2025
-
How Many Yards Are In 13 Feet
May 08, 2025
-
How Do You Spell Notebook In Spanish
May 08, 2025
Related Post
Thank you for visiting our website which covers about 5 Divided By 3 In A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.