5 To The Power Of Negative 1
Arias News
Mar 31, 2025 · 5 min read
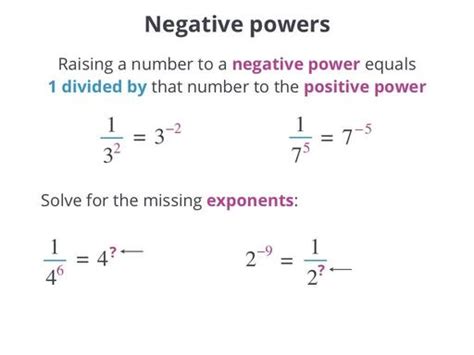
Table of Contents
Decoding 5 to the Power of Negative 1: A Deep Dive into Negative Exponents
Understanding exponents, especially negative ones, can be a stumbling block for many. This comprehensive guide will illuminate the meaning and application of 5 to the power of negative 1 (5⁻¹), exploring its mathematical foundation, practical uses, and broader implications within the realm of algebra and beyond. We'll delve into the core concepts, providing clear explanations and examples to solidify your grasp of this seemingly complex topic.
What Does 5⁻¹ Actually Mean?
At its core, 5⁻¹ represents the reciprocal of 5. The negative exponent doesn't signify a negative number; instead, it indicates an inverse operation. Remember the fundamental rule of exponents: a⁻ⁿ = 1/aⁿ. Applying this rule to 5⁻¹, we get:
5⁻¹ = 1/5¹ = 1/5
Therefore, 5 to the power of negative 1 is simply one-fifth or 0.2. This seemingly simple equation unlocks a gateway to understanding more complex exponential expressions.
The Reciprocal: The Heart of Negative Exponents
The concept of the reciprocal is crucial to grasping negative exponents. The reciprocal of a number is simply 1 divided by that number. For example:
- The reciprocal of 5 is 1/5.
- The reciprocal of 10 is 1/10.
- The reciprocal of 1/2 is 2/1 or 2. (Notice how reciprocals 'flip' fractions.)
This "flipping" is key. When we encounter a negative exponent, we essentially "flip" the base and make the exponent positive. This provides a practical method for simplifying expressions with negative exponents.
Working with Negative Exponents: Practical Examples
Let's expand our understanding with some practical examples. Understanding how to manipulate negative exponents is essential for simplifying complex algebraic expressions.
Example 1: Simplifying Expressions
Simplify the expression: (2x⁻³y²)⁻²
Solution:
First, apply the power rule to the entire expression, distributing the -2 exponent:
(2⁻²x⁶y⁻⁴)
Then, rewrite the negative exponents as reciprocals:
1/2² * x⁶ * 1/y⁴ = x⁶ / (4y⁴)
This demonstrates how negative exponents become positive when moved to the denominator.
Example 2: Solving Equations
Solve for x: 5⁻ˣ = 1/125
Solution:
We know that 125 is 5³, so we can rewrite the equation as:
5⁻ˣ = 1/5³ = 5⁻³
Since the bases are equal, the exponents must be equal:
-x = -3
Therefore, x = 3.
Example 3: Scientific Notation
Negative exponents frequently appear in scientific notation, a concise way to represent very large or very small numbers. For instance, the speed of light, approximately 299,792,458 meters per second, can be written in scientific notation as 2.99792458 x 10⁸. Conversely, a very small number like 0.0000000001 can be written as 1 x 10⁻¹⁰. This notation relies heavily on understanding negative exponents to represent the decimal placement.
Negative Exponents and the Laws of Exponents
Negative exponents seamlessly integrate with all the standard laws of exponents. Let's review how they work in conjunction:
-
Product Rule: aᵐ * aⁿ = aᵐ⁺ⁿ (This holds true even with negative exponents. For example, 5⁻² * 5³ = 5¹ = 5)
-
Quotient Rule: aᵐ / aⁿ = aᵐ⁻ⁿ (Again, applicable to negative exponents. For example, 5⁻²/5⁻⁴ = 5²)
-
Power Rule: (aᵐ)ⁿ = aᵐⁿ (This also works perfectly with negative exponents. For instance, (5⁻²)³ = 5⁻⁶)
Mastering these rules with both positive and negative exponents is key to confidently manipulating algebraic expressions.
Beyond the Basics: Applications in Advanced Mathematics
The seemingly simple concept of 5⁻¹ has profound implications throughout various branches of mathematics. It forms the foundation for:
-
Calculus: Understanding negative exponents is essential for working with derivatives and integrals, crucial concepts in calculus.
-
Linear Algebra: Negative exponents appear in matrix operations and linear transformations.
-
Complex Numbers: Negative exponents can be applied to complex numbers, expanding their applications in advanced mathematical fields.
-
Differential Equations: Many differential equations involve exponential functions with both positive and negative exponents.
Practical Applications in Real-World Scenarios
Beyond pure mathematics, the understanding of negative exponents proves valuable in various practical fields:
-
Physics: Describing radioactive decay, where the amount of substance decreases exponentially, often employs negative exponents.
-
Engineering: Many engineering calculations, especially those involving exponential growth or decay, utilize this concept.
-
Finance: Compound interest calculations, including scenarios with declining balances, often rely on negative exponents.
-
Computer Science: Algorithms and data structures frequently use exponential functions, encompassing negative exponents.
Common Mistakes to Avoid
Several common pitfalls can hinder your understanding of negative exponents. Be mindful of these:
-
Confusing negative exponents with negative numbers: Remember, a negative exponent indicates a reciprocal, not a negative value.
-
Incorrectly applying exponent rules: Pay close attention to the order of operations and ensure you're applying the laws of exponents correctly.
-
Neglecting the base: The base of the exponent is equally important. Don't forget to apply the negative exponent to the entire base.
Practice Makes Perfect
The best way to truly grasp the concept of 5⁻¹ and negative exponents in general is through consistent practice. Work through various examples, solve equations, and challenge yourself with increasingly complex problems. The more you practice, the more comfortable and proficient you'll become.
Conclusion: Unlocking the Power of Negative Exponents
5⁻¹—while seemingly a simple expression—serves as a gateway to a deeper understanding of exponentiation. Its significance extends far beyond basic arithmetic, finding practical applications across diverse fields. By mastering the concept of reciprocals and the laws of exponents, you unlock a powerful tool for solving complex problems and advancing your mathematical proficiency. Remember, consistent practice is the key to fluency. Embrace the challenges, and you'll find that the seemingly intimidating world of negative exponents becomes remarkably accessible and rewarding.
Latest Posts
Latest Posts
-
5 Ft 4 In Inches In Meters
Apr 01, 2025
-
What Is The Answer For A Subtraction Problem Called
Apr 01, 2025
-
How Do You Say Say Cheese In Spanish
Apr 01, 2025
-
How Tall Is 33 Inches In Feet
Apr 01, 2025
-
How Many Zeros Are In 1 5 Million
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about 5 To The Power Of Negative 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
