55 Out Of 80 As A Percentage
Arias News
May 11, 2025 · 5 min read
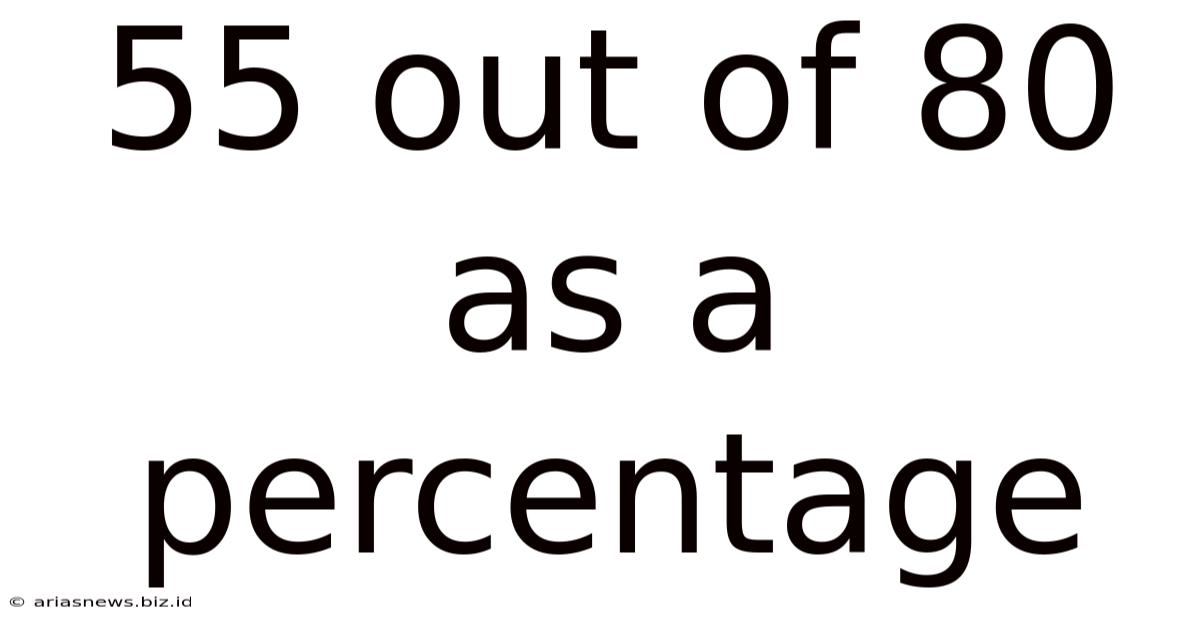
Table of Contents
55 out of 80 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill applicable across various fields, from academic assessments to financial analysis and everyday life. Understanding how to convert fractions into percentages is crucial for interpreting data, making informed decisions, and communicating effectively. This comprehensive guide will meticulously explain how to calculate 55 out of 80 as a percentage, explore different calculation methods, and provide practical applications. We'll delve into the underlying concepts, address common misconceptions, and offer helpful tips to master percentage calculations.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "per hundred" or "out of one hundred." Therefore, a percentage represents the proportional relationship between a part and a whole, where the whole is always considered to be 100. For instance, 50% means 50 out of 100, or half.
Calculating 55 out of 80 as a Percentage: The Basic Method
The most straightforward method to calculate 55 out of 80 as a percentage involves two simple steps:
-
Form a Fraction: Express the given numbers as a fraction. In this case, 55 out of 80 is written as 55/80.
-
Convert to a Decimal: Divide the numerator (55) by the denominator (80). 55 ÷ 80 = 0.6875
-
Convert to a Percentage: Multiply the decimal result by 100. 0.6875 x 100 = 68.75%
Therefore, 55 out of 80 is equal to 68.75%.
Alternative Calculation Methods
While the basic method is effective, other methods can provide a slightly different perspective or simplify the calculation depending on the context.
Using Proportions
Proportions offer a powerful method for solving percentage problems. We can set up a proportion to find the percentage:
55/80 = x/100
Cross-multiplying, we get:
80x = 5500
Solving for x:
x = 5500/80 = 68.75
Therefore, x = 68.75%, confirming our previous result.
Using a Calculator
Calculators are indispensable tools for handling percentage calculations, especially with more complex numbers. Most calculators have a percentage function (%) that simplifies the process. Simply enter 55 ÷ 80 and then press the % button. The calculator will directly display the result as 68.75%.
Practical Applications of Percentage Calculations
The ability to calculate percentages has far-reaching applications in numerous real-world scenarios. Here are a few examples:
-
Academic Performance: Calculating grades, understanding class averages, and assessing individual progress often involve percentage calculations. If you score 55 out of 80 on a test, knowing your percentage (68.75%) provides a clear picture of your performance.
-
Financial Calculations: Percentages are essential in finance for calculating interest rates, discounts, taxes, profit margins, and investment returns. Understanding how much you're earning or losing as a percentage allows for better financial planning and decision-making.
-
Data Analysis: In business, statistics, and research, percentages are used to represent data proportions, track trends, and compare different groups. Analyzing sales figures, market shares, or survey results often requires converting raw data into percentages for meaningful interpretation.
-
Everyday Life: Calculating tips in restaurants, determining sale discounts in stores, understanding tax rates, or even comparing the nutritional value of food products all involve applying percentage calculations.
Common Mistakes to Avoid
While percentage calculations are relatively straightforward, common mistakes can occur. Here are some pitfalls to avoid:
-
Incorrect Order of Operations: Always remember to divide the part (55) by the whole (80) before multiplying by 100. Reversing the order leads to an incorrect result.
-
Misunderstanding the "Whole": Accurately identifying the "whole" in a problem is crucial. The whole is the total amount or the base value against which the part is being compared.
-
Ignoring Decimal Places: Rounding off decimals prematurely can lead to significant inaccuracies, particularly when dealing with financial or scientific calculations. It's advisable to retain at least two decimal places for accuracy.
-
Confusing Percentage Increase/Decrease with Absolute Values: Be cautious when interpreting percentage changes. A 10% increase followed by a 10% decrease does not result in the original value; the overall result will be lower.
Advanced Percentage Calculations: Building on the Foundation
Beyond the basic calculation of 55 out of 80, you can build your skills by tackling more complex scenarios.
-
Percentage Increase/Decrease: Calculating the percentage increase or decrease between two values helps analyze changes over time or compare different sets of data. For example, you could calculate the percentage increase in sales from one year to the next.
-
Percentage Change: This calculation determines the relative change between an old and a new value. It's particularly useful when analyzing fluctuations in stock prices, population growth, or inflation rates.
-
Compound Interest: This calculation determines the total accumulated value of an investment or loan, taking into account the effects of repeated interest payments over time. Understanding compound interest is essential for long-term financial planning.
Mastering Percentage Calculations: Tips and Resources
Consistent practice is key to mastering percentage calculations. Here are some tips to improve your skills:
-
Practice regularly: Solve various percentage problems from different contexts to build your proficiency.
-
Use visual aids: Diagrams and charts can help visualize percentage relationships, making calculations easier to understand.
-
Utilize online resources: Numerous online calculators and tutorials are available to support your learning.
-
Seek help when needed: Don't hesitate to ask for clarification from teachers, tutors, or online communities if you encounter difficulties.
Conclusion
Calculating 55 out of 80 as a percentage, which equals 68.75%, is a fundamental skill applicable across a wide range of contexts. Understanding the different calculation methods, avoiding common mistakes, and practicing regularly are key to mastering this skill. By developing proficiency in percentage calculations, you'll be better equipped to analyze data, make informed decisions, and navigate various aspects of your personal and professional life. Remember that the ability to work with percentages is not just about mathematical computation; it's about understanding and applying proportional relationships to solve real-world problems.
Latest Posts
Latest Posts
-
Five Letter Word Second Letter E Fourth Letter A
May 12, 2025
-
Is The Diameter Half Of The Circumference
May 12, 2025
-
How Are The Elements Arranged In The Modern Periodic Table
May 12, 2025
-
How Many Miles Is 25 Laps Around A Track
May 12, 2025
-
How Many Mg In A 1 8 Teaspoon
May 12, 2025
Related Post
Thank you for visiting our website which covers about 55 Out Of 80 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.