6 Times The Square Root Of 2
Arias News
Apr 02, 2025 · 6 min read
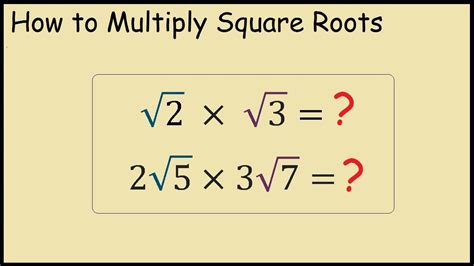
Table of Contents
6 Times the Square Root of 2: Unpacking a Seemingly Simple Number
The expression "6 times the square root of 2" might seem deceptively simple. It's a concise mathematical statement, but beneath its brevity lies a wealth of mathematical concepts and applications. This article will delve deep into this seemingly simple number, exploring its properties, its presence in various fields, and its significance in both theoretical and applied mathematics. We will unravel its mysteries and uncover its surprising connections to geometry, trigonometry, and even the real world.
Understanding the Basics: What is 6√2?
Before we embark on our exploration, let's establish a firm understanding of the core concept: 6√2. This expression represents the product of 6 and the square root of 2. The square root of 2 (√2) is an irrational number, meaning it cannot be expressed as a simple fraction. Its approximate value is 1.41421356..., and it continues infinitely without repeating. Multiplying this by 6 gives us approximately 8.48528137..., another irrational number. This seemingly simple calculation opens up a world of mathematical possibilities.
Irrational Numbers: A Quick Recap
The irrationality of √2 is a cornerstone of mathematics. It's a classic example of a number that cannot be expressed as the ratio of two integers. Its discovery by the ancient Greeks was a pivotal moment in the history of mathematics, forcing a reassessment of their understanding of numbers and proportions. The proof of its irrationality relies on the method of contradiction, demonstrating that assuming √2 is rational leads to a logical contradiction. This highlights the richness and complexity even within seemingly simple numerical expressions.
6√2 in Geometry: Diagonal of a Square and Beyond
The number 6√2 finds frequent appearances in geometric contexts. Its most straightforward connection lies with squares and their diagonals.
The Diagonal of a Square: A Fundamental Relationship
Consider a square with sides of length 6 units. Using the Pythagorean theorem (a² + b² = c²), where a and b are the lengths of the sides of a right-angled triangle and c is the length of the hypotenuse, we can calculate the length of the square's diagonal. In this case, a = 6 and b = 6. Therefore, c² = 6² + 6² = 72, and c = √72 = √(36 x 2) = 6√2. Thus, the diagonal of a square with sides of length 6 is precisely 6√2 units. This simple geometric relationship underscores the significance of this number in spatial calculations.
Extending the Concept: Other Geometric Applications
The appearance of 6√2 isn't limited to just squares. This number can emerge in calculations involving other geometric shapes, particularly those that involve right-angled triangles or can be broken down into right-angled triangles. For example, it could represent a specific dimension in a more complex polygon, or even a component of a three-dimensional structure. Understanding its connection to the Pythagorean theorem allows us to easily recognize and utilize this number in various geometric problems.
6√2 in Trigonometry: Angles and Relationships
Trigonometry, the study of triangles, also incorporates 6√2 in various scenarios. While not as directly apparent as in geometry, its presence often arises in calculations involving specific angles and triangle properties.
Working with Special Angles
Specific angles in trigonometry, particularly those involving multiples of 45 degrees, often lead to expressions involving √2. When working with triangles containing these angles, calculations might result in numbers like 6√2 representing a side length or a specific trigonometric function value. The relationship between these angles and the square root of 2 stems from the properties of isosceles right-angled triangles.
Applications in Vector Analysis
In vector analysis, which is crucial in physics and engineering, vectors often have components that can involve irrational numbers like 6√2. This number might represent the magnitude of a vector or a specific component of a vector in a two-dimensional or three-dimensional space.
6√2 in Physics and Engineering: Real-World Applications
While seemingly abstract, the number 6√2 finds real-world applications in various fields, especially in those relying heavily on geometric calculations and vector analysis.
Structural Engineering and Architecture
In structural engineering and architecture, precise calculations are essential for ensuring stability and functionality. The dimensions of structures often involve numbers like 6√2, arising from the need to build stable and balanced designs. For instance, the diagonal bracing in a structure might be calculated using this number to ensure optimal strength.
Mechanical Engineering and Robotics
Mechanical engineering and robotics utilize vector analysis extensively. Calculating forces, velocities, and movements often leads to expressions involving irrational numbers, including 6√2. This number might represent a specific component of force, a distance traveled, or a component of velocity in a complex mechanical system.
6√2 in Computer Science and Programming
Although not as directly visible as in geometry or physics, 6√2 plays a subtle role in computer science, particularly in areas that involve numerical computations and simulations.
Numerical Algorithms and Computations
Numerical algorithms often rely on approximations of irrational numbers. While computers can't store irrational numbers perfectly, they can approximate them to a high degree of accuracy. 6√2 might be used in computations within numerical methods, such as solving equations or performing simulations.
Simulations and Modeling
In simulations and modeling, where real-world phenomena are approximated, 6√2 could represent a specific parameter in a model. The accuracy of such models hinges on the precision of numerical calculations, and this number contributes to the accuracy of calculations within those models.
Approximations and Practical Considerations
While the exact value of 6√2 is irrational and non-terminating, in practical applications, we often need to work with approximations.
Using Decimal Approximations
A common approach involves using a decimal approximation, such as 8.485. The level of precision required depends on the context. For some applications, a rough approximation suffices; for others, higher accuracy is needed. The choice of precision is crucial in ensuring the reliability and accuracy of calculations.
Error Analysis and Accuracy
It's important to consider the implications of using approximations. The error introduced by using an approximation of 6√2 can propagate through calculations, potentially leading to inaccuracies in the final result. Therefore, a careful analysis of the error introduced by the approximation is often essential.
Conclusion: The Significance of 6√2
The number 6√2, while initially appearing simple, reveals a rich mathematical tapestry upon closer examination. From its fundamental connection to the geometry of squares and the Pythagorean theorem to its applications in trigonometry, physics, engineering, and even computer science, its significance extends far beyond its concise mathematical expression. Understanding this number allows us to appreciate the interconnectedness of various mathematical fields and their practical applications in the world around us. Its apparent simplicity belies its profound and widespread influence on a multitude of disciplines. It serves as a perfect example of how seemingly basic mathematical concepts can underpin complex and crucial calculations and applications across diverse fields. Further exploration into its properties and applications would undoubtedly uncover even more fascinating connections and relationships within the vast landscape of mathematics and its applications.
Latest Posts
Latest Posts
-
Things That Look Like The Letter A
Apr 03, 2025
-
How Long Can Your Hair Grow In A Lifetime
Apr 03, 2025
-
How Many Teaspoons In A Pound Of Salt
Apr 03, 2025
-
One And One Tenth As A Decimal
Apr 03, 2025
-
How Many Grams Are In One Liter Of Water
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about 6 Times The Square Root Of 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
