8 Divided By 6 As A Fraction
Arias News
Apr 13, 2025 · 5 min read
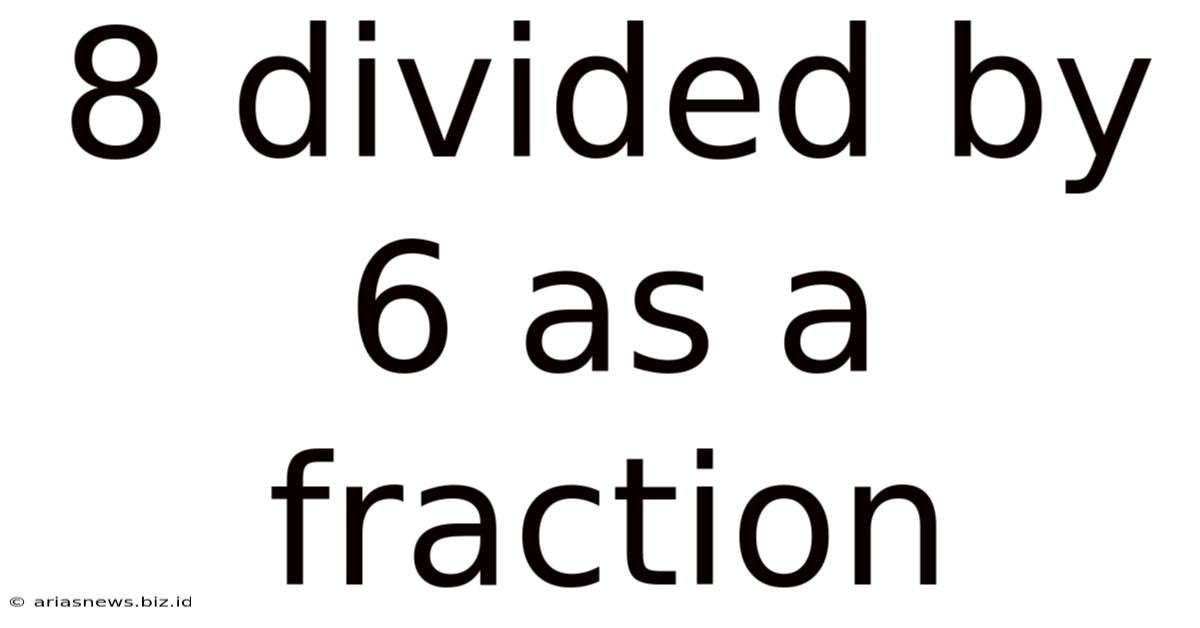
Table of Contents
8 Divided by 6 as a Fraction: A Comprehensive Guide
Understanding how to represent division as a fraction is a fundamental concept in mathematics. This comprehensive guide delves into the process of expressing 8 divided by 6 as a fraction, exploring the underlying principles, simplification techniques, and practical applications. We'll cover everything from the basics to more advanced concepts, ensuring a thorough understanding for all levels.
Understanding Fractions and Division
Before diving into the specifics of 8 divided by 6, let's refresh our understanding of fractions and their relationship to division. A fraction represents a part of a whole. It's composed of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts we have, while the denominator indicates the total number of equal parts the whole is divided into.
Division, on the other hand, is the process of splitting a quantity into equal groups. The crucial connection between fractions and division is that division can always be expressed as a fraction. The dividend (the number being divided) becomes the numerator, and the divisor (the number we're dividing by) becomes the denominator.
Expressing 8 Divided by 6 as a Fraction
Following this principle, 8 divided by 6 can be easily represented as a fraction:
8/6
This fraction indicates that we have 8 parts out of a total of 6 equal parts. This might seem counterintuitive at first – how can we have more parts than the whole is divided into? This highlights the importance of simplification and understanding improper fractions.
Simplifying Fractions: Finding the Lowest Terms
The fraction 8/6 is an improper fraction because the numerator (8) is larger than the denominator (6). Improper fractions are perfectly valid, but they are often simplified to make them easier to understand and work with. Simplification involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it.
The GCD of 8 and 6 is 2. Dividing both the numerator and the denominator by 2, we get:
8 ÷ 2 = 4 6 ÷ 2 = 3
Therefore, the simplified fraction is 4/3.
Converting Improper Fractions to Mixed Numbers
While 4/3 is a perfectly acceptable way to represent the result of 8 divided by 6, it's often beneficial to convert improper fractions into mixed numbers. A mixed number combines a whole number and a proper fraction.
To convert 4/3 to a mixed number, we perform the division:
4 ÷ 3 = 1 with a remainder of 1
This means that 4/3 contains one whole unit and one-third of another unit. So, the mixed number representation of 4/3 is:
1 1/3
Decimals and Their Relationship to Fractions
It's also useful to understand the decimal equivalent of 8/6 (or 4/3). To convert a fraction to a decimal, we simply divide the numerator by the denominator:
4 ÷ 3 ≈ 1.333...
The result is a repeating decimal, indicated by the ellipsis (...). This demonstrates that some fractions cannot be perfectly represented as finite decimals.
Real-World Applications
The concept of representing division as a fraction is widely applicable in various real-world scenarios:
-
Sharing Resources: Imagine you have 8 pizzas to share equally among 6 friends. The fraction 8/6 (or 4/3) represents the amount of pizza each friend receives – more than one whole pizza each.
-
Measurement: In construction or cooking, you might need to divide quantities. For example, if a recipe calls for 8 cups of flour but you only have 6-cup measuring cups, you'll need to use 8/6 (or 4/3) cups per measuring cup.
-
Data Analysis: In statistics and data analysis, representing proportions as fractions is common. If 8 out of 60 people surveyed answered "yes" to a question, the fraction 8/60 (simplified to 2/15) represents the proportion of "yes" responses.
-
Financial Calculations: Dividing profits or expenses among partners often leads to fractional representations.
Advanced Concepts and Further Exploration
Beyond the basics, exploring more advanced concepts can deepen your understanding:
-
Equivalent Fractions: Different fractions can represent the same value. For instance, 8/6, 4/3, and 16/12 are all equivalent fractions.
-
Complex Fractions: These are fractions where the numerator or denominator (or both) are also fractions. For example, (8/6)/(2/3) is a complex fraction. Solving complex fractions involves multiplying the numerator by the reciprocal of the denominator.
-
Operations with Fractions: Adding, subtracting, multiplying, and dividing fractions require specific techniques. Understanding these techniques is crucial for solving more complex mathematical problems involving fractions.
-
Ratio and Proportion: Fractions are intimately related to ratios and proportions, which are fundamental tools in various fields like science, engineering, and finance.
Addressing Common Misconceptions
-
The order of operations: Remember that the order of operations (PEMDAS/BODMAS) applies to calculations involving fractions and division. Parentheses/brackets, exponents/orders, multiplication and division (from left to right), addition and subtraction (from left to right).
-
Improper fractions are not "wrong": Improper fractions are perfectly valid mathematical representations. Their simplification to mixed numbers often offers a more intuitive interpretation, but both forms are correct.
-
Simplifying to the lowest terms: Always simplify fractions to their lowest terms to make calculations easier and interpretations clearer.
Conclusion: Mastering Fractions and Division
Representing 8 divided by 6 as a fraction (8/6, simplified to 4/3 or 1 1/3) is a foundational skill in mathematics with numerous real-world applications. Understanding the process, including simplification techniques and the relationship between fractions, division, and decimals, equips you with essential tools for problem-solving across various disciplines. By mastering these concepts, you'll build a strong foundation for more advanced mathematical explorations. Remember to practice regularly to solidify your understanding and build confidence in working with fractions. Continuously revisiting these concepts and exploring related topics will strengthen your mathematical abilities. The journey to mastering fractions is a continuous one, full of opportunities for learning and growth.
Latest Posts
Latest Posts
-
1 Pint Of Sour Cream Is How Many Cups
May 09, 2025
-
What Personality Trait Most Likely Leads To Victors Downfall
May 09, 2025
-
Is It Illegal To Put A Flyer In A Mailbox
May 09, 2025
-
How Many Oz In 1 Lb Of Meat
May 09, 2025
-
How Long Is 50 Months In Years
May 09, 2025
Related Post
Thank you for visiting our website which covers about 8 Divided By 6 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.