A Triangle With At Least Two Congruent Sides
Arias News
May 08, 2025 · 6 min read
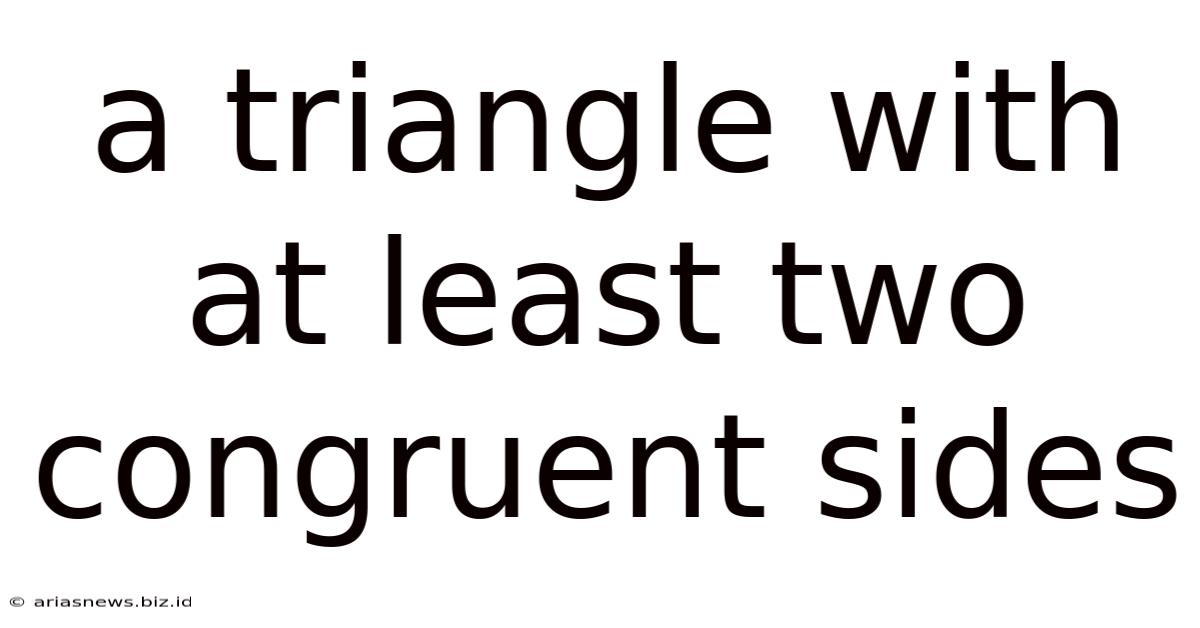
Table of Contents
A Triangle with at Least Two Congruent Sides: Exploring Isosceles Triangles
A triangle, the fundamental building block of geometry, captivates with its simplicity and versatility. Among the diverse family of triangles, a particular type stands out due to its unique properties: the isosceles triangle. This article delves deep into the fascinating world of isosceles triangles, exploring their definition, characteristics, theorems, and various applications in mathematics and beyond. We will examine its properties, explore related theorems, and uncover its significance in diverse fields.
Defining an Isosceles Triangle: More Than Just Two Equal Sides
An isosceles triangle is defined as a triangle with at least two congruent sides. These congruent sides are called legs, and the third side is called the base. The angles opposite the congruent sides are called base angles, and the angle opposite the base is called the vertex angle. It's crucial to note the "at least two" part of the definition. This subtly implies that an equilateral triangle, possessing three congruent sides, is also considered a special case of an isosceles triangle. This seemingly minor detail has significant implications when analyzing the properties of these shapes.
Distinguishing Isosceles from Other Triangles
To fully understand isosceles triangles, it’s essential to differentiate them from other types of triangles:
- Equilateral Triangles: These triangles have all three sides congruent, making them a special subset of isosceles triangles. All angles in an equilateral triangle are also congruent (60° each).
- Scalene Triangles: These triangles have no congruent sides, and consequently, no congruent angles.
- Right-Angled Triangles: These triangles have one angle equal to 90°. A right-angled triangle can be isosceles (with two legs of equal length) or scalene.
Understanding these distinctions is crucial for accurately applying theorems and solving problems related to triangles.
Key Properties of Isosceles Triangles: Unveiling the Symmetry
Isosceles triangles exhibit a striking symmetry, leading to several key properties:
-
Congruent Base Angles: The most fundamental property of an isosceles triangle is that its base angles are congruent. This is a cornerstone theorem used extensively in geometric proofs and problem-solving. The converse is also true: if a triangle has two congruent angles, then it is an isosceles triangle.
-
Altitude Bisects the Vertex Angle: The altitude drawn from the vertex angle to the base bisects both the vertex angle and the base. This property creates two congruent right-angled triangles, simplifying many geometric calculations.
-
Median Bisects the Base: The median drawn from the vertex angle to the base bisects the base. This median is also the altitude and the angle bisector, highlighting the inherent symmetry of the isosceles triangle.
-
Perpendicular Bisector of the Base: The perpendicular bisector of the base passes through the vertex angle. This property provides a useful construction method for drawing an isosceles triangle given specific parameters.
Exploring Theorems Related to Isosceles Triangles: Proof and Application
Several important geometric theorems directly relate to isosceles triangles, providing powerful tools for solving complex geometric problems:
-
Isosceles Triangle Theorem: This theorem formally states that in an isosceles triangle, the base angles are congruent. This theorem forms the foundation for many other proofs and constructions. Its proof often utilizes congruent triangles and the properties of angle bisectors.
-
Converse of the Isosceles Triangle Theorem: This theorem states that if two angles of a triangle are congruent, then the sides opposite those angles are also congruent, making the triangle isosceles. This theorem provides a powerful method for proving triangle congruence and determining the nature of triangles based solely on their angles.
-
Exterior Angle Theorem: While not exclusive to isosceles triangles, the exterior angle theorem is particularly useful when dealing with isosceles triangles. The exterior angle of a triangle is equal to the sum of the two opposite interior angles. This becomes especially helpful when determining the measure of unknown angles in an isosceles triangle.
Applications of Isosceles Triangles: From Geometry to Real-World Scenarios
The elegance and inherent symmetry of isosceles triangles extend beyond theoretical geometry, finding practical applications in various fields:
-
Architecture and Construction: Isosceles triangles are frequently used in architectural designs, particularly in roof structures and supporting frameworks. Their inherent strength and stability make them ideal for constructing durable and aesthetically pleasing structures. The isosceles triangle’s geometry enables efficient load distribution.
-
Engineering: Engineering applications leverage the predictable properties of isosceles triangles in structural design, ensuring stability and load-bearing capacity in bridges, buildings, and other constructions.
-
Art and Design: The balanced aesthetic of isosceles triangles finds applications in various art forms, from painting and sculpture to graphic design and logo creation. The symmetry contributes to visual harmony and balance.
-
Computer Graphics and Game Development: The mathematical precision of isosceles triangles allows for precise representation of shapes and objects in computer graphics and game development, enabling realistic simulations and compelling visual experiences.
Solving Problems Involving Isosceles Triangles: A Step-by-Step Guide
Let's consider a few example problems to illustrate the application of the properties and theorems discussed:
Problem 1: Find the measure of the base angles in an isosceles triangle if the vertex angle measures 70°.
Solution: Since the sum of angles in a triangle is 180°, and the base angles are congruent, we can deduce that:
180° - 70° = 110° (sum of base angles)
110° / 2 = 55° (measure of each base angle)
Therefore, each base angle measures 55°.
Problem 2: Prove that if two angles of a triangle are congruent, the triangle is isosceles.
Solution: This requires a geometric proof, often involving constructing an altitude and using congruent triangle postulates (like SAS or ASA). The proof establishes the congruence of the sides opposite the equal angles, hence proving the triangle is isosceles. The details of such a proof would be beyond the scope of this introductory article but are readily available in standard geometry textbooks.
Problem 3: In an isosceles triangle, the length of the base is 10 cm, and the length of each leg is 13 cm. Find the height of the triangle.
Solution: The altitude from the vertex angle to the base bisects the base. This creates two right-angled triangles. Using the Pythagorean theorem on one of these right-angled triangles (with legs of 5 cm and h cm, and hypotenuse of 13 cm), we can solve for h (the height):
5² + h² = 13² 25 + h² = 169 h² = 144 h = 12 cm
Advanced Concepts and Further Exploration: Delving Deeper into Isosceles Triangles
This introduction has scratched the surface of the rich mathematical landscape surrounding isosceles triangles. For a more in-depth exploration, consider investigating these advanced topics:
-
Isosceles Triangle Area Formulas: Deriving and applying different formulas for calculating the area of an isosceles triangle, depending on the known parameters (base and height, sides, etc.).
-
Isosceles Triangles in Coordinate Geometry: Representing and analyzing isosceles triangles using coordinate systems, applying coordinate geometry techniques to solve problems involving their vertices and sides.
-
Isosceles Triangles and Trigonometry: Utilizing trigonometric functions (sine, cosine, tangent) to solve problems involving angles and side lengths in isosceles triangles.
-
Applications in Higher Mathematics: Exploring the role of isosceles triangles in more advanced mathematical concepts like geometry, trigonometry, and calculus.
Conclusion: The Enduring Significance of Isosceles Triangles
The seemingly simple isosceles triangle holds a position of profound significance in geometry and beyond. Its inherent symmetry, coupled with its well-defined properties and theorems, provides a powerful tool for problem-solving, analysis, and design. From architectural marvels to computer-generated imagery, the impact of the isosceles triangle is undeniable. Understanding its properties is crucial for anyone seeking a deeper understanding of geometric principles and their applications in the real world. This article has only touched upon the many facets of this fascinating geometric shape, encouraging further exploration and a deeper appreciation of its mathematical elegance and real-world relevance.
Latest Posts
Latest Posts
-
Lowest Common Multiple Of 16 And 24
May 08, 2025
-
Why Was Madonna Not Credited In Die Another Day
May 08, 2025
-
Calories In 1 Lb Of Ground Beef
May 08, 2025
-
A Parking Brake System Is Not Required If
May 08, 2025
-
How Much Is 2 3 Cup In Ounces
May 08, 2025
Related Post
Thank you for visiting our website which covers about A Triangle With At Least Two Congruent Sides . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.