Are 3 And 8 Prime To Each Other
Arias News
Mar 31, 2025 · 5 min read
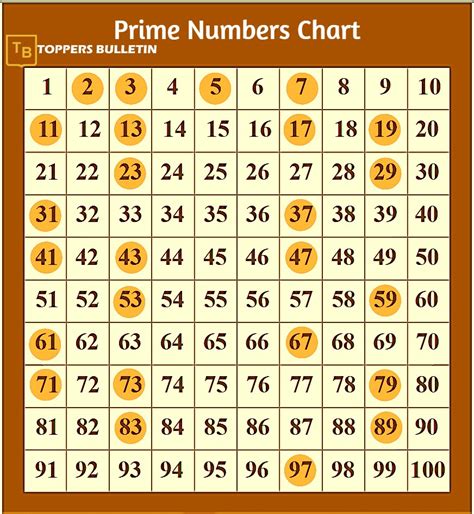
Table of Contents
Are 3 and 8 Prime to Each Other? A Deep Dive into Coprime Numbers
The question, "Are 3 and 8 prime to each other?" might seem simple at first glance. However, it opens the door to a fascinating area of number theory: the concept of coprime numbers, also known as relatively prime numbers. Understanding coprime numbers is crucial in various mathematical fields and has practical applications in cryptography and computer science. This article will not only answer the question definitively but also delve into the underlying theory, exploring related concepts and providing examples to solidify your understanding.
What are Prime Numbers? A Quick Refresher
Before we tackle the core question, let's briefly revisit the definition of prime numbers. A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. Prime numbers are fundamental building blocks in number theory, forming the basis for many important theorems and applications.
Understanding Coprime (Relatively Prime) Numbers
Two integers are said to be coprime, or relatively prime, if the only positive integer that divides both of them is 1. In other words, their greatest common divisor (GCD) is 1. This doesn't mean that the numbers themselves have to be prime. One or both can be composite (non-prime). The key is that they share no common factors other than 1.
Determining if 3 and 8 are Coprime
Now, let's address the central question: Are 3 and 8 prime to each other?
To determine this, we need to find the greatest common divisor (GCD) of 3 and 8. We can use a few methods to achieve this:
1. Listing Factors:
- Factors of 3: 1, 3
- Factors of 8: 1, 2, 4, 8
The only common factor between 3 and 8 is 1. Therefore, their greatest common divisor (GCD) is 1.
2. Euclidean Algorithm:
The Euclidean algorithm is a highly efficient method for finding the GCD of two integers. It's based on the principle that the GCD of two numbers doesn't change if the larger number is replaced by its difference with the smaller number. This process is repeated until the two numbers are equal, and that number is the GCD.
Let's apply the Euclidean algorithm to 3 and 8:
- 8 = 2 * 3 + 2
- 3 = 1 * 2 + 1
- 2 = 2 * 1 + 0
The last non-zero remainder is 1, so the GCD(3, 8) = 1.
Conclusion: Since the GCD(3, 8) = 1, we can definitively say that yes, 3 and 8 are coprime (relatively prime) to each other.
Examples of Coprime and Non-Coprime Numbers
Let's look at a few more examples to solidify our understanding:
Coprime Examples:
- 15 and 28: Factors of 15 are 1, 3, 5, 15. Factors of 28 are 1, 2, 4, 7, 14, 28. The GCD is 1.
- 9 and 16: Factors of 9 are 1, 3, 9. Factors of 16 are 1, 2, 4, 8, 16. The GCD is 1.
- 7 and 10: Factors of 7 are 1, 7. Factors of 10 are 1, 2, 5, 10. The GCD is 1.
Non-Coprime Examples:
- 12 and 18: Factors of 12 are 1, 2, 3, 4, 6, 12. Factors of 18 are 1, 2, 3, 6, 9, 18. The GCD is 6.
- 25 and 35: Factors of 25 are 1, 5, 25. Factors of 35 are 1, 5, 7, 35. The GCD is 5.
- 14 and 21: Factors of 14 are 1, 2, 7, 14. Factors of 21 are 1, 3, 7, 21. The GCD is 7.
Applications of Coprime Numbers
The concept of coprime numbers extends beyond theoretical mathematics; it finds significant applications in various fields:
1. Cryptography:
Coprime numbers play a vital role in RSA cryptography, one of the most widely used public-key cryptosystems. The security of RSA relies on the difficulty of factoring large composite numbers into their prime factors. The algorithm involves selecting two large prime numbers and using their product to generate the public and private keys.
2. Computer Science:
In computer science, coprime numbers are used in algorithms for simplifying fractions and solving Diophantine equations. These equations involve finding integer solutions, and the coprime condition often simplifies the solution process.
3. Music Theory:
Surprisingly, coprime numbers even appear in music theory. Intervals in music are often described using ratios of frequencies, and coprime ratios frequently represent consonant intervals, leading to more pleasing-sounding combinations.
Beyond the Basics: Deeper Exploration of Coprime Numbers
The topic of coprime numbers extends much further than the basic definition and examples provided above. Here are some advanced concepts worth exploring:
1. Euler's Totient Function: This function, denoted by φ(n), counts the number of positive integers less than or equal to n that are coprime to n. It has many significant applications in number theory and cryptography.
2. Chinese Remainder Theorem: This theorem provides a solution to systems of congruences, where the moduli (the divisors) are pairwise coprime.
3. Continued Fractions: Coprime numbers arise naturally in the study of continued fractions, providing a powerful way to represent irrational numbers.
Conclusion: The Significance of Coprime Numbers
In conclusion, the seemingly simple question of whether 3 and 8 are prime to each other unveils a rich and multifaceted area of mathematics. The concept of coprime numbers, their properties, and their applications in diverse fields highlight their fundamental importance in number theory and beyond. Understanding coprime numbers is essential for anyone interested in exploring the intricacies of mathematics and its practical applications in cryptography, computer science, and other related areas. The fact that 3 and 8 share only 1 as a common divisor underscores the crucial role of the greatest common divisor (GCD) in determining coprimality, a concept with far-reaching consequences in various branches of knowledge.
Latest Posts
Latest Posts
-
What Is A 44 Out Of 60
Apr 01, 2025
-
What Do You Call Your Aunts Husband
Apr 01, 2025
-
How Much Is 3 4 Oz In Tablespoons
Apr 01, 2025
-
How Many Oz In An Egg White
Apr 01, 2025
-
Which Sentence Is Written In Second Person Point Of View
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Are 3 And 8 Prime To Each Other . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
