How Many 1/3 Are There In 9
Arias News
May 09, 2025 · 5 min read
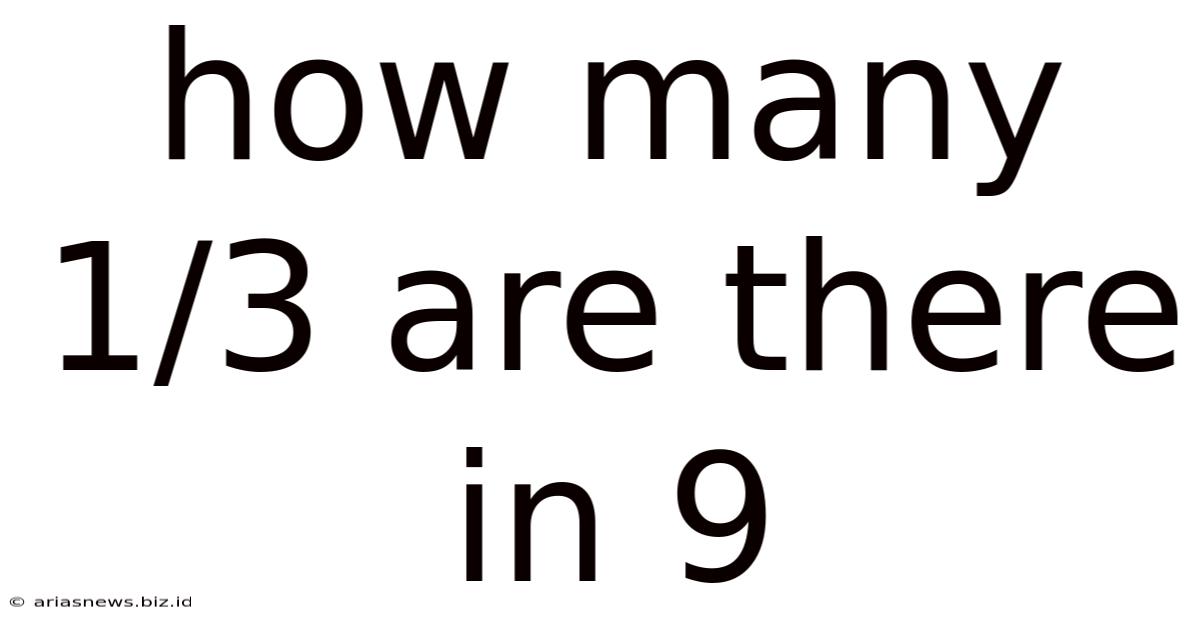
Table of Contents
How Many 1/3s Are There in 9? A Deep Dive into Fraction Division
This seemingly simple question, "How many 1/3s are there in 9?", opens the door to a fascinating exploration of fraction division, a fundamental concept in mathematics with far-reaching applications. While the answer itself is straightforward, understanding the underlying principles and exploring various approaches to solving this problem provides a valuable foundation for tackling more complex fractional calculations. This article will delve into multiple methods, offering a comprehensive understanding of the concept and its practical implications.
Understanding the Problem: Visualizing 1/3 in 9
Before diving into the mathematical solutions, let's visualize the problem. Imagine you have 9 whole pizzas. Each pizza is divided into 3 equal slices. Each slice represents 1/3 of a pizza. The question, "How many 1/3s are there in 9?", is essentially asking how many of these 1/3 slices you can get from 9 whole pizzas.
Intuitively, you can likely see that each pizza yields 3 slices (3 x 1/3 = 1). Since there are 9 pizzas, the total number of 1/3 slices is 9 * 3 = 27. This visual approach helps solidify the understanding before tackling the more formal mathematical methods.
Method 1: Converting to a Division Problem
The question "How many 1/3s are there in 9?" can be directly translated into a division problem: 9 ÷ (1/3). This is a crucial step in understanding how to approach fraction division.
To solve this, we recall the rule of dividing by a fraction: We invert the second fraction (the divisor) and multiply.
Therefore, the problem becomes:
9 ÷ (1/3) = 9 x (3/1) = 9 x 3 = 27
This confirms our intuitive answer from the pizza analogy. There are 27 one-third slices in 9 whole pizzas.
Understanding the Inversion Principle
Why do we invert and multiply when dividing fractions? This stems from the reciprocal relationship between a fraction and its inverse. The reciprocal of a fraction, a/b, is b/a. Multiplying a fraction by its reciprocal always results in 1.
For example: (2/5) x (5/2) = 10/10 = 1
This principle is used to eliminate the division operation and turn it into multiplication, which is often simpler to compute.
Method 2: Using Common Denominators
Another approach involves finding a common denominator. While perhaps less efficient for this particular problem, understanding this method is crucial for solving more complex fraction division problems.
To begin, we rewrite 9 as a fraction with a denominator of 3:
9 = 9/1 = (9 x 3) / (1 x 3) = 27/3
Now, the question becomes: How many 1/3s are there in 27/3? This is equivalent to:
(27/3) ÷ (1/3)
When dividing fractions with a common denominator, we can simply divide the numerators:
27 ÷ 1 = 27
Again, we arrive at the answer: 27.
Method 3: Using the Concept of Reciprocals
This method directly utilizes the concept of reciprocals to understand the division process. Recall that dividing by a fraction is equivalent to multiplying by its reciprocal.
We can represent the problem as:
9 / (1/3) = 9 * (3/1) = 27
This highlights the core relationship between division and multiplication with fractions. Dividing by a fraction is fundamentally the same as multiplying by its inverse.
Expanding the Concept: Real-World Applications
The concept of dividing by fractions is not merely an abstract mathematical exercise. It has numerous practical applications in everyday life and various professional fields.
-
Cooking: If a recipe calls for 1/3 cup of sugar, and you want to triple the recipe, you would calculate 3 x (1/3) cup = 1 cup.
-
Sewing: If you need to cut a piece of fabric into pieces that are 1/3 of a yard long, and you have 9 yards of fabric, you would calculate 9 / (1/3) = 27 to find the number of pieces you can cut.
-
Construction: Many construction projects involve fractional measurements. Dividing by fractions is essential for accurately calculating materials needed and determining the dimensions of components.
-
Data Analysis: Analyzing data often requires working with fractions and percentages. Understanding fraction division is crucial for accurately interpreting data and drawing meaningful conclusions.
Beyond the Basics: More Complex Problems
While this article focuses on the relatively simple problem of 9 ÷ (1/3), the principles discussed here can be applied to more complex fraction division problems. For instance:
-
Dividing fractions by fractions: This involves applying the "invert and multiply" rule twice, if necessary.
-
Dividing mixed numbers: This requires first converting mixed numbers into improper fractions before applying the division rule.
-
Dividing fractions involving decimals: This often necessitates converting decimals to fractions first to simplify the calculation.
Conclusion: Mastering Fraction Division
The seemingly simple question, "How many 1/3s are there in 9?", serves as a gateway to understanding the crucial concept of fraction division. Through various approaches, including visualization, direct conversion to division, using common denominators, and employing the principle of reciprocals, we have demonstrated that there are 27 one-third units within 9. Mastering fraction division is essential not only for success in mathematics but also for practical applications in numerous fields. By grasping the underlying principles and practicing different methods, one can confidently tackle more challenging fraction problems and appreciate the versatility of this fundamental mathematical operation. The key is to practice regularly and to understand the 'why' behind each calculation method, thus fostering a deeper comprehension that extends beyond rote memorization.
Latest Posts
Latest Posts
-
What Percentage Is 44 Out Of 60
May 09, 2025
-
What Is The Shortest Book In The Old Testament
May 09, 2025
-
What Does In Launcher Mean In Fortnite
May 09, 2025
-
How Many Cups Are In 1 Pound Of Citric Acid
May 09, 2025
-
Civil War Words That Start With X
May 09, 2025
Related Post
Thank you for visiting our website which covers about How Many 1/3 Are There In 9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.