How Many Sixths Are Equivalent To 2/3
Arias News
Apr 01, 2025 · 5 min read
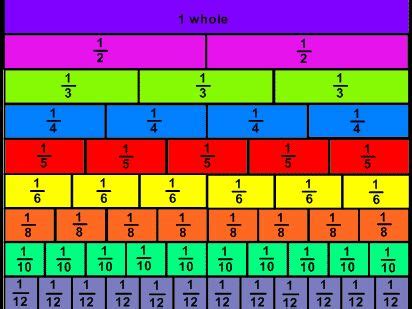
Table of Contents
How Many Sixths Are Equivalent to 2/3? A Deep Dive into Fraction Equivalence
Understanding fractions is a cornerstone of mathematics, crucial for everything from baking a cake to complex engineering calculations. This article delves into the seemingly simple question: how many sixths are equivalent to 2/3? While the answer itself is straightforward, exploring the process reveals fundamental concepts of fraction equivalence, simplification, and common denominators—essential skills for anyone looking to master fractions.
Understanding Fractions: A Quick Refresher
Before we tackle the central question, let's refresh our understanding of fractions. A fraction represents a part of a whole. It consists of two main components:
- Numerator: The top number, indicating how many parts we have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
For example, in the fraction 2/3, the numerator (2) represents the number of parts we possess, and the denominator (3) indicates that the whole is divided into three equal parts.
Finding Equivalent Fractions: The Core Concept
Equivalent fractions represent the same value even though they look different. They are essentially different ways of expressing the same portion of a whole. To find equivalent fractions, we multiply or divide both the numerator and the denominator by the same non-zero number. This process doesn't change the fundamental value of the fraction.
For instance, 1/2 is equivalent to 2/4, 3/6, 4/8, and so on. We obtain these equivalent fractions by multiplying both the numerator and the denominator by the same number (2, 3, 4, etc.). Conversely, we can simplify fractions by dividing both the numerator and denominator by their greatest common divisor (GCD).
Solving the Puzzle: How Many Sixths are in 2/3?
Now, let's address the core question: how many sixths are equivalent to 2/3? We need to find an equivalent fraction for 2/3 that has a denominator of 6.
There are two primary methods to achieve this:
Method 1: Finding the Common Denominator
The key is to find a common denominator for both fractions – in this case, 6. We need to determine what number we can multiply the denominator of 2/3 (which is 3) by to get 6. That number is 2 (3 x 2 = 6).
Crucially, whatever we do to the denominator, we must do to the numerator to maintain the fraction's value. So, if we multiply the denominator by 2, we also multiply the numerator by 2:
(2 x 2) / (3 x 2) = 4/6
Therefore, 4/6 is equivalent to 2/3. This means there are four sixths in 2/3.
Method 2: Using Proportions
Another way to approach this is using proportions. We can set up a proportion to solve for the unknown number of sixths:
2/3 = x/6
To solve for 'x', we can cross-multiply:
3x = 2 * 6
3x = 12
x = 12 / 3
x = 4
Again, this confirms that four sixths are equivalent to 2/3.
Visualizing the Equivalence: A Pictorial Representation
Visual aids can significantly enhance understanding. Imagine a circle divided into three equal parts. Shading two of these parts represents 2/3.
Now, imagine the same circle divided into six equal parts. To represent the same area as the shaded portion in the first circle (2/3), you would need to shade four of the six parts. This visually demonstrates that 2/3 and 4/6 are equivalent fractions.
Expanding on Fraction Equivalence: Further Exploration
The concept of equivalent fractions extends beyond this simple example. It’s a fundamental concept used in various mathematical operations, including:
- Adding and Subtracting Fractions: To add or subtract fractions, they must have a common denominator. Finding equivalent fractions with a common denominator is a crucial first step.
- Comparing Fractions: Determining which of two fractions is larger or smaller often involves converting them to equivalent fractions with a common denominator for easier comparison.
- Simplifying Fractions: Reducing fractions to their simplest form involves finding the greatest common divisor of the numerator and denominator and dividing both by it. This results in an equivalent fraction but with smaller, more manageable numbers.
Real-World Applications of Fraction Equivalence
The ability to work with equivalent fractions isn't just a theoretical skill; it has numerous practical applications in various aspects of life:
- Cooking and Baking: Recipes often require precise measurements, and understanding equivalent fractions is crucial for adjusting ingredient quantities based on the available measuring tools. For example, if a recipe calls for 1/2 cup of flour and you only have a 1/4 cup measuring cup, you know you need to use two 1/4 cups.
- Construction and Engineering: Precision is paramount in construction and engineering. Accurate measurements and calculations using fractions are essential for creating stable and functional structures.
- Finance and Budgeting: Understanding fractions is critical for managing personal finances and understanding percentages, interest rates, and other financial concepts.
- Data Analysis: When working with data, fractions often represent proportions or percentages, and the ability to manipulate and understand equivalent fractions is essential for interpreting data accurately.
Advanced Concepts: Ratios and Proportions
The principles of fraction equivalence are intimately linked to ratios and proportions. A ratio compares two quantities, often expressed as a fraction. A proportion is a statement that two ratios are equal. Solving proportions involves applying the principles of equivalent fractions to find unknown quantities.
Conclusion: Mastering Fractions for a Brighter Future
The seemingly simple question of how many sixths are equivalent to 2/3 opens a door to a deeper understanding of fractions and their pervasive role in mathematics and daily life. Mastering the concepts of equivalent fractions, common denominators, and simplification is essential for success in various fields. By understanding these principles and practicing regularly, you can build a solid mathematical foundation and confidently tackle more complex challenges. Remember, the ability to work comfortably with fractions is a valuable asset that will serve you well throughout your life. Keep practicing, and soon, you'll find yourself effortlessly navigating the world of fractions.
Latest Posts
Latest Posts
-
How Many Meters Are In A Block
Apr 02, 2025
-
How To Give Yourself A Hanging Wedgie
Apr 02, 2025
-
How Much Is 14 Milligrams Of Gold Worth
Apr 02, 2025
-
How Many 500 Ml In A Liter
Apr 02, 2025
-
How Many Fl Oz In 3 Liters
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about How Many Sixths Are Equivalent To 2/3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
