How Much Is 1 3 Cup Plus 1 3 Cup
Arias News
May 12, 2025 · 5 min read
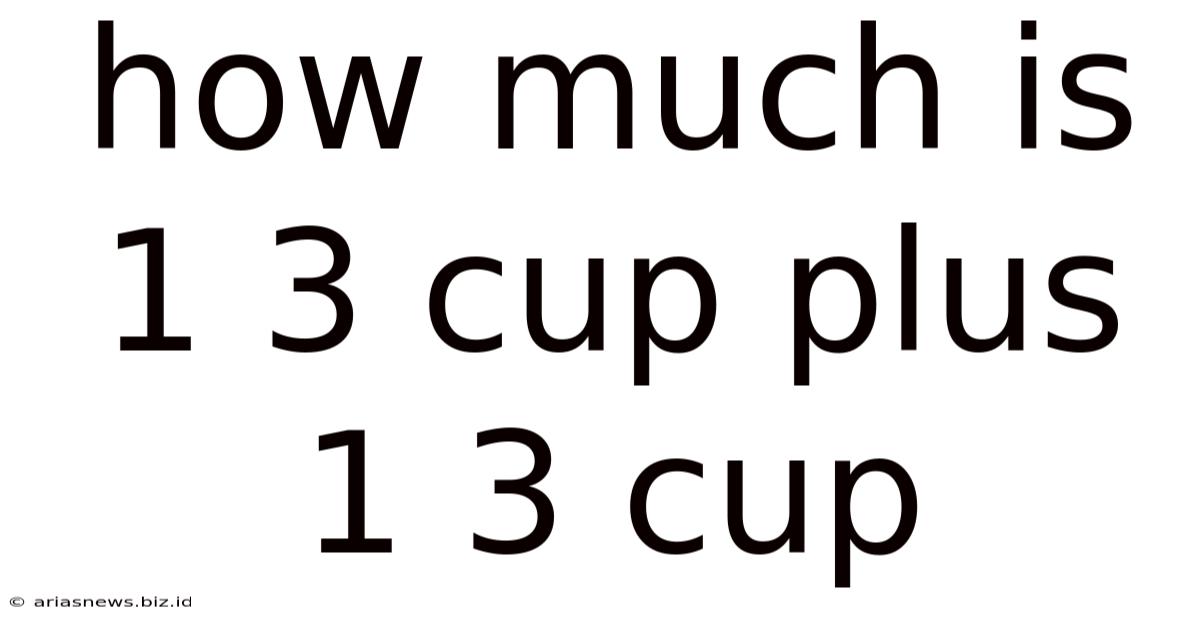
Table of Contents
How Much is ⅓ Cup Plus ⅓ Cup? A Deep Dive into Fraction Addition and Culinary Applications
This seemingly simple question, "How much is ⅓ cup plus ⅓ cup?", opens a door to a surprisingly rich exploration of fractions, their practical applications in everyday life, especially in cooking and baking, and even touches upon advanced mathematical concepts. While the immediate answer is straightforward, understanding the underlying principles allows for greater confidence and accuracy in various situations.
The Straightforward Answer: ⅔ Cup
Adding ⅓ cup and ⅓ cup is a basic addition problem involving fractions. Simply put:
⅓ + ⅓ = ⅔
Therefore, ⅓ cup plus ⅓ cup equals two-thirds (⅔) of a cup.
This answer is crucial for anyone working with recipes, particularly those that call for fractional measurements. Understanding this fundamental addition prevents inaccurate measurements and potentially disastrous results in the kitchen.
Understanding Fractions: A Foundation for Culinary Accuracy
Fractions are a fundamental part of mathematics, representing parts of a whole. In the context of cooking, the "whole" is often a cup, a tablespoon, or a teaspoon. Mastering fractions is vital for accurate recipe scaling and avoiding culinary mishaps.
What is a Fraction?
A fraction consists of two parts: a numerator (the top number) and a denominator (the bottom number). The numerator indicates the number of parts you have, while the denominator indicates the total number of equal parts that make up the whole.
In our example, ⅓, the numerator is 1 (indicating one part), and the denominator is 3 (indicating the whole is divided into three equal parts).
Adding Fractions with the Same Denominator
Adding fractions with the same denominator is relatively straightforward. You simply add the numerators and keep the denominator the same. For example:
- 2/5 + 1/5 = (2+1)/5 = 3/5
This principle directly applies to our original problem:
- ⅓ + ⅓ = (1+1)/3 = ⅔
Practical Applications in Cooking and Baking
Accurate measurements are paramount in cooking and baking. A slight variation in the amount of an ingredient can significantly impact the final product's taste, texture, and overall quality. Understanding fraction addition helps achieve consistent and delicious results.
Recipe Scaling
Many recipes are designed for a specific number of servings. If you need to adjust the recipe to feed more or fewer people, you'll need to scale the ingredient quantities accordingly. This frequently involves working with fractions. For example, if a recipe calls for ⅓ cup of sugar per serving and you want to make two servings, you'll need to calculate ⅓ + ⅓ = ⅔ cup of sugar.
Ingredient Substitutions
Sometimes, you might need to substitute one ingredient for another. If the substitution isn't a direct 1:1 replacement, you'll need to adjust the quantity, often using fractions. Proper fraction calculation ensures that the substitution doesn't negatively impact the final product.
Precise Measurements
In baking, particularly, precision is key. Even small differences in ingredient quantities can affect the texture and rise of baked goods. Understanding fractions allows for precise measurements, leading to more consistent and predictable results.
Beyond the Basics: More Complex Fraction Problems
While adding ⅓ + ⅓ is relatively simple, let's explore more complex scenarios that might arise in cooking and baking, solidifying your understanding of fractions and their applications:
Adding Fractions with Different Denominators
When dealing with fractions that have different denominators (like ½ + ⅓), you must find a common denominator before adding them. A common denominator is a number that is a multiple of both denominators. For example, for ½ and ⅓, a common denominator is 6. You then convert each fraction to an equivalent fraction with the common denominator:
- ½ = 3/6
- ⅓ = 2/6
Now you can add them:
- 3/6 + 2/6 = 5/6
Mixed Numbers and Improper Fractions
Recipes might use mixed numbers (a whole number and a fraction, like 1 ½) or improper fractions (where the numerator is larger than the denominator, like 5/3). You'll need to convert these into a consistent format (usually improper fractions) before performing addition or other operations.
Subtraction, Multiplication, and Division of Fractions
While our initial question focused on addition, understanding fraction subtraction, multiplication, and division is also important for accurate recipe adjustments and ingredient substitutions.
-
Subtraction: Subtracting fractions follows a similar process to addition. If the denominators are the same, subtract the numerators. If different, find a common denominator first.
-
Multiplication: To multiply fractions, multiply the numerators together and then multiply the denominators together.
-
Division: To divide fractions, invert the second fraction (flip the numerator and denominator) and then multiply.
Advanced Mathematical Concepts: Exploring the Underlying Principles
While cooking primarily involves basic fraction arithmetic, exploring the underlying mathematical principles enriches understanding and opens doors to more complex scenarios.
Decimal Equivalents
Fractions can be represented as decimals. For example, ⅓ is approximately 0.333..., and ⅔ is approximately 0.666.... Understanding decimal equivalents can be helpful when using digital kitchen scales or working with recipes that provide measurements in both fractions and decimals.
Ratio and Proportion
The concept of ratio and proportion is deeply intertwined with fractions. Recipes often represent ingredient quantities as ratios (e.g., a 2:1 ratio of flour to water). Understanding ratio and proportion allows for scaling recipes accurately based on the desired quantity.
Percentage Calculations
Percentages are another way to represent fractions. For instance, ⅓ is approximately 33.33%. Understanding the relationship between fractions and percentages is useful in adjusting recipes and understanding nutritional information.
Conclusion: Mastering Fractions for Culinary Success
While the simple addition of ⅓ cup plus ⅓ cup results in ⅔ cup, the underlying principles and applications are far-reaching. A strong grasp of fractions is essential for anyone who enjoys cooking and baking, ensuring accurate measurements, consistent results, and the creation of delicious and satisfying meals. The ability to work confidently with fractions not only enhances culinary skills but also strengthens mathematical understanding, demonstrating the practical relevance of mathematical concepts in everyday life. By mastering fraction operations, you unlock a world of precision and culinary creativity.
Latest Posts
Related Post
Thank you for visiting our website which covers about How Much Is 1 3 Cup Plus 1 3 Cup . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.