How To Find The Height Of The Trapezoid
Arias News
Apr 06, 2025 · 7 min read
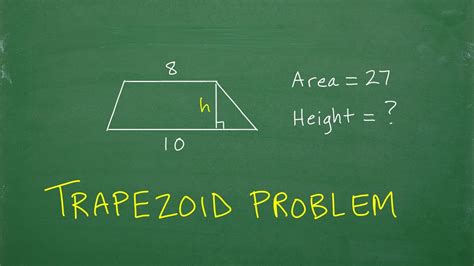
Table of Contents
How to Find the Height of a Trapezoid: A Comprehensive Guide
Finding the height of a trapezoid might seem like a simple geometry problem, but understanding the different approaches and their applications is crucial. This comprehensive guide will delve into various methods for calculating the height, catering to different scenarios and levels of mathematical understanding. We'll cover everything from basic formulas to more advanced techniques, ensuring you're equipped to tackle any trapezoid height challenge.
Understanding the Trapezoid and its Height
A trapezoid (or trapezium) is a quadrilateral with at least one pair of parallel sides. These parallel sides are called bases (often denoted as 'b1' and 'b2'), while the non-parallel sides are called legs. The height (often denoted as 'h') of a trapezoid is the perpendicular distance between the two parallel bases. This is the crucial measurement we'll be focusing on calculating.
It's important to differentiate the height from the lengths of the legs. The height is always perpendicular to the bases. This distinction is fundamental to correctly applying the formulas.
Method 1: Using the Area and Base Lengths
This is arguably the most common method, especially when the area of the trapezoid is known. The formula for the area (A) of a trapezoid is:
A = (1/2) * (b1 + b2) * h
Where:
- A is the area of the trapezoid
- b1 and b2 are the lengths of the parallel bases
- h is the height of the trapezoid
To find the height (h), we simply rearrange the formula:
h = (2 * A) / (b1 + b2)
Example:
Let's say a trapezoid has an area of 30 square centimeters, and its bases measure 8 cm and 4 cm. To find the height:
h = (2 * 30) / (8 + 4) = 60 / 12 = 5 cm
Therefore, the height of the trapezoid is 5 cm.
Practical Applications of this Method
This method is frequently used in practical scenarios involving land surveying, architecture, and engineering. For example:
- Land Surveying: Calculating the area of a plot of land shaped like a trapezoid requires knowing the height. This method allows for accurate area determination using base measurements and the overall land area.
- Architecture: Determining the height of a trapezoidal window or wall section is essential for accurate construction plans. The area might be known from architectural blueprints, allowing for the calculation of the necessary height.
- Engineering: In structural engineering, understanding the height of trapezoidal components is crucial for calculating load-bearing capabilities and stability.
Method 2: Using Trigonometry in Right-Angled Triangles
If you know the length of one leg and the angle it makes with one of the bases, you can use trigonometry to find the height. Imagine drawing a perpendicular line from one end of the shorter base to the longer base. This creates two right-angled triangles.
Let's say:
- l is the length of the leg
- θ is the angle between the leg and the base
- h is the height
Then, using trigonometry:
h = l * sin(θ)
Example:
Suppose a trapezoid has a leg length of 10 cm and the angle between this leg and the base is 30 degrees. The height would be:
h = 10 * sin(30°) = 10 * 0.5 = 5 cm
Applications of the Trigonometric Method
This method proves invaluable in situations where direct measurement of the height is difficult or impossible. Consider:
- Inaccessible Heights: When dealing with large trapezoidal structures or terrains, direct height measurement may be impractical. Trigonometry offers a solution using readily measurable angles and distances.
- Complex Shapes: This method is particularly useful when the trapezoid is part of a more complex geometrical structure, enabling the calculation of the height within a broader context.
- Indirect Measurement: Surveyors often rely on trigonometric methods for accurate height determination in situations where direct measurement is challenging due to obstacles or terrain.
Method 3: Dividing the Trapezoid into Rectangles and Triangles
This method involves dividing the trapezoid into simpler shapes whose heights are easier to determine. You can divide the trapezoid into a rectangle and two right-angled triangles.
- Identify a rectangle: Draw a line perpendicular to the bases from one of the shorter base ends to the longer base. This creates a rectangle.
- Calculate the rectangle's height: The rectangle's height is equal to the shorter base's length.
- Find the triangle's height: The difference between the longer and shorter base lengths divided by 2 gives you the base of each of the two right triangles. Using the leg length and the calculated base of the triangle, you can apply the Pythagorean theorem or trigonometric functions to find the height of the triangle.
- Combine the heights: The height of the trapezoid is the sum of the height of the rectangle and the height of the triangle.
This is a more complex method but highly effective when working with known leg lengths and base differences.
When to Use this Method
This is a particularly useful method when:
- Leg lengths are known: Having the leg lengths allows for the calculation of the dimensions of the created triangles.
- Visual decomposition aids understanding: Breaking the trapezoid down into simpler shapes can improve visual understanding, especially for beginners.
- Multiple solutions exist: This method provides an alternative approach, useful when comparing results with other methods for error checking.
Method 4: Using Coordinate Geometry
If the vertices of the trapezoid are defined by coordinates in a Cartesian plane, we can use the distance formula and the concept of slopes to determine the height.
- Find the equation of the line representing one of the bases: Use two points on one of the parallel bases to determine the line's equation using the point-slope form or the two-point form.
- Find the perpendicular distance: Use the formula for the perpendicular distance from a point to a line. Choose a point on the other base and calculate its perpendicular distance to the line representing the first base. This distance is the height of the trapezoid.
This is a more advanced method requiring knowledge of coordinate geometry.
Applications of Coordinate Geometry
This is a powerful method when:
- Coordinates are available: When dealing with digital representations of the trapezoid, such as in CAD software or geographic information systems (GIS), coordinate data is readily available.
- Precise calculations are needed: Coordinate geometry methods allow for very precise height calculations, minimizing errors.
- Automated calculations: This method is readily adaptable for automation within software programs for efficient and repeatable trapezoid height determination.
Choosing the Right Method
The best method for finding the height of a trapezoid depends on the information available.
- Area and base lengths known: Use Method 1.
- Leg length and angle known: Use Method 2.
- Leg lengths and base lengths known: Use Method 3.
- Coordinates of vertices known: Use Method 4.
Remember to always carefully consider the given information and choose the most efficient and accurate method accordingly. Practicing with different examples will solidify your understanding and build your problem-solving skills.
Beyond the Basics: Advanced Trapezoid Problems
While the methods described above cover the most common scenarios, you might encounter more complex trapezoid problems. These might involve:
- Isosceles Trapezoids: In isosceles trapezoids (where the legs are equal in length), there are additional relationships between the angles and sides that can be exploited to find the height.
- Cyclic Trapezoids: If a trapezoid is cyclic (meaning all four vertices lie on a circle), specific geometric properties can simplify the height calculation.
- Trapezoids within complex shapes: Often, trapezoids are components of larger geometric figures. In such cases, understanding the overall shape and its properties is crucial for determining the trapezoid's height.
Conclusion: Mastering Trapezoid Height Calculations
Finding the height of a trapezoid is a fundamental geometrical problem with wide-ranging applications. By mastering the different methods outlined in this guide – from using the area and base lengths to applying trigonometry or coordinate geometry – you'll be well-equipped to tackle a variety of challenges. Remember to always carefully analyze the available information before selecting the most appropriate method. The key is to understand the underlying principles and choose the approach best suited to the given data and context. With practice, you’ll become confident and efficient in your trapezoid height calculations.
Latest Posts
Latest Posts
-
How Many Hours Is 2pm To 6pm
Apr 06, 2025
-
Who Is The Mother Of Yannis Daughter Krystal
Apr 06, 2025
-
What Is The Lcm Of 25 And 15
Apr 06, 2025
-
What Was One Question Gilderoy Lockhart Asked
Apr 06, 2025
-
Michael Myers Theme Song On Piano Notes
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about How To Find The Height Of The Trapezoid . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
