If 40 Is Equal To The Fraction X/30
Arias News
May 10, 2025 · 5 min read
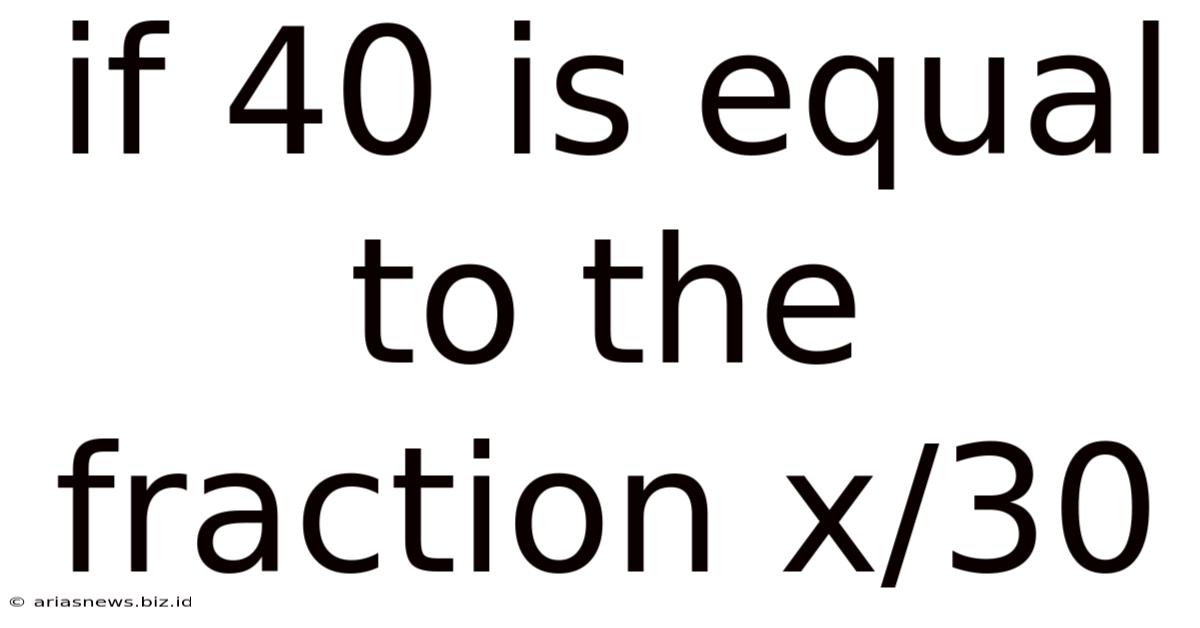
Table of Contents
If 40 is Equal to the Fraction x/30: A Comprehensive Exploration of Ratios, Proportions, and Problem-Solving
This article delves into the seemingly simple equation, 40 = x/30, exploring its implications within the broader context of ratios, proportions, and fundamental mathematical problem-solving techniques. We'll move beyond the immediate solution to examine the underlying principles, demonstrate various solution methods, and highlight the practical applications of such equations in everyday life and various fields.
Understanding Ratios and Proportions
Before tackling the equation directly, let's establish a firm understanding of ratios and proportions. A ratio is a comparison of two or more quantities. It expresses the relative sizes of those quantities. We can represent a ratio using a colon (e.g., 2:3), as a fraction (e.g., 2/3), or using the word "to" (e.g., 2 to 3).
A proportion is a statement that two ratios are equal. Proportions are incredibly useful for solving a wide range of problems involving scaling, scaling up, and scaling down, percentage calculations, and comparing quantities. They form the backbone of many real-world applications, from cooking and construction to finance and scientific research.
Our equation, 40 = x/30, is a simple proportion. It states that the ratio of x to 30 is equal to the ratio of 40 to 1. Understanding this fundamental equivalence is key to unlocking the solution and appreciating its broader significance.
Solving the Equation: Method 1 - Cross-Multiplication
The most straightforward method to solve 40 = x/30 is through cross-multiplication. This technique involves multiplying both sides of the equation by the denominator of the fraction to isolate the variable 'x'.
Here's how it works:
-
Start with the equation: 40 = x/30
-
Multiply both sides by 30: 40 * 30 = (x/30) * 30
-
Simplify: 1200 = x
Therefore, the value of x that satisfies the equation is 1200.
Solving the Equation: Method 2 - Inverting the Fraction
Another approach involves manipulating the equation by inverting the fraction. While seemingly less intuitive than cross-multiplication, this method demonstrates a different facet of algebraic manipulation.
-
Start with the equation: 40 = x/30
-
Invert both sides (reciprocate): 1/40 = 30/x
-
Cross-multiply (or simply solve for x): x = 30 * 40
-
Simplify: x = 1200
This method yields the same result, confirming the accuracy of our solution. It highlights the flexibility of algebraic manipulation and allows us to explore different problem-solving strategies.
Visualizing the Proportion: A Real-World Analogy
Let's consider a real-world analogy to further solidify our understanding. Imagine you're scaling a recipe. The original recipe calls for 30 units of ingredient A to make a certain quantity of food. You want to make a larger batch that requires 40 units of the final product. The proportion 40 = x/30 helps you determine how much of ingredient A you need for the larger batch.
Solving for x (x = 1200) means you'll need 1200 units of ingredient A to achieve your desired larger batch size. This demonstrates the practical application of proportions in everyday tasks like cooking, baking, and other scaling operations.
Beyond the Solution: Extending the Concepts
While finding x = 1200 is the primary objective, the equation 40 = x/30 provides a springboard to explore more advanced concepts:
Understanding Scale Factors:
The equation reveals a scale factor of 40/1, meaning the quantity represented by 'x' is 40 times larger than 30. This concept of scale factors is crucial in various fields, including:
- Cartography: Maps utilize scale factors to represent large geographical areas on smaller surfaces.
- Engineering: Scale models are used to design and test structures before full-scale construction.
- Computer Graphics: Scaling images and objects is a fundamental aspect of digital design and animation.
Proportional Reasoning:
Solving this proportion strengthens proportional reasoning skills. This is a crucial cognitive ability that involves understanding relationships between quantities and applying this understanding to solve problems. It’s vital for:
- Problem-solving in general: Many real-world problems involve proportional relationships.
- Data analysis: Interpreting data often requires understanding proportions and ratios.
- Critical thinking: Analyzing arguments and making informed decisions often depends on proportional reasoning.
Applications in Various Fields:
The principles underlying this simple equation are applicable across numerous fields:
- Finance: Calculating interest, returns on investment, and exchange rates frequently involves proportions.
- Science: Proportions are used extensively in chemistry, physics, and biology for calculations involving concentrations, ratios of reactants and products, and population dynamics.
- Business: Estimating costs, sales projections, and market share often relies on proportional reasoning.
Potential Challenges and Misconceptions
While seemingly straightforward, solving equations like 40 = x/30 can present challenges for some learners:
- Fractions and decimals: Students unfamiliar with manipulating fractions might struggle to solve the equation efficiently.
- Algebraic manipulation: A solid understanding of algebraic principles, such as cross-multiplication and solving for a variable, is crucial.
- Contextual understanding: Applying the solution to real-world scenarios requires understanding the context and correctly interpreting the resulting value of 'x'.
Addressing these potential challenges through targeted teaching methods and real-world examples enhances the learning experience and fosters deeper understanding.
Conclusion: The Significance of a Simple Equation
The equation 40 = x/30, seemingly elementary at first glance, serves as a powerful gateway to a deeper understanding of ratios, proportions, and their widespread applications. Mastering the solution methods and appreciating the underlying principles allows for more effective problem-solving, enhancing skills crucial for various academic and professional endeavors. By exploring the different solution methods and considering real-world analogies, we can solidify our understanding and appreciate the practical significance of this fundamental mathematical concept. The ability to interpret and solve such equations isn't just about mathematical prowess; it's about developing critical thinking skills and fostering a stronger grasp of numerical relationships that permeate our daily lives.
Latest Posts
Latest Posts
-
Appleton Farms Spiral Sliced Ham Aldi Cooking Instructions
May 11, 2025
-
What Can You Multiply To Get 12
May 11, 2025
-
8 1 3 As An Improper Fraction
May 11, 2025
-
Greatest Common Factor Of 63 And 45
May 11, 2025
-
What Is The Decimal For 9 100
May 11, 2025
Related Post
Thank you for visiting our website which covers about If 40 Is Equal To The Fraction X/30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.