Is 1 3 Bigger Than 2 5
Arias News
May 09, 2025 · 5 min read
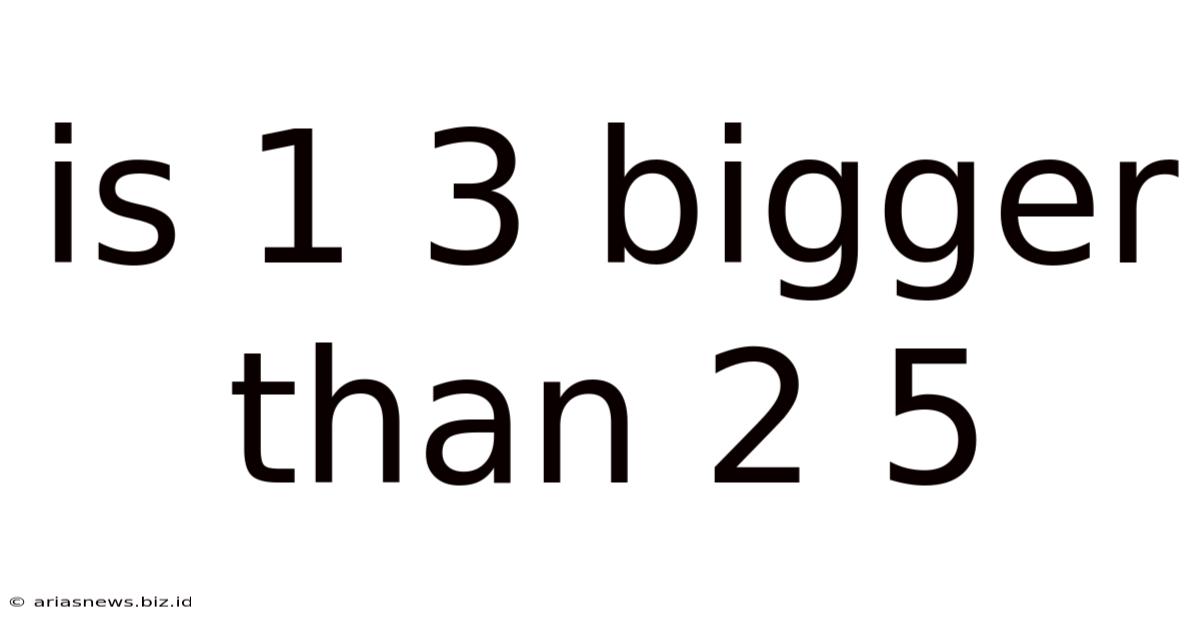
Table of Contents
Is 1/3 Bigger Than 2/5? A Deep Dive into Fraction Comparison
Determining whether 1/3 is bigger than 2/5 might seem like a simple task, especially for those comfortable with fractions. However, understanding the underlying principles of fraction comparison is crucial for a solid grasp of mathematical concepts. This article provides a comprehensive exploration of this seemingly simple question, delving into various methods for comparing fractions and highlighting the importance of such comparisons in various fields.
Understanding Fractions
Before comparing 1/3 and 2/5, let's refresh our understanding of fractions. A fraction represents a part of a whole. It's composed of two key components:
- Numerator: The top number, indicating the number of parts considered.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
Therefore, 1/3 signifies one part out of three equal parts, while 2/5 represents two parts out of five equal parts. The challenge lies in comparing these parts when they are from differently sized wholes.
Method 1: Finding a Common Denominator
The most common and widely accepted method for comparing fractions is to find a common denominator. This involves converting both fractions to equivalent fractions with the same denominator. The least common multiple (LCM) of the denominators is the most efficient common denominator to use.
Steps:
-
Find the LCM of the denominators: The denominators are 3 and 5. The LCM of 3 and 5 is 15 (3 x 5 = 15).
-
Convert each fraction:
- For 1/3, multiply both the numerator and the denominator by 5: (1 x 5) / (3 x 5) = 5/15
- For 2/5, multiply both the numerator and the denominator by 3: (2 x 3) / (5 x 3) = 6/15
-
Compare the numerators: Now that both fractions have the same denominator, compare their numerators. 5/15 is smaller than 6/15.
Conclusion: Therefore, 1/3 (5/15) is smaller than 2/5 (6/15).
Method 2: Converting to Decimals
Another effective method is to convert both fractions into decimals and then compare them. This method is particularly useful when dealing with more complex fractions or when a numerical comparison is required.
Steps:
-
Convert 1/3 to a decimal: Divide the numerator (1) by the denominator (3): 1 ÷ 3 ≈ 0.333...
-
Convert 2/5 to a decimal: Divide the numerator (2) by the denominator (5): 2 ÷ 5 = 0.4
-
Compare the decimals: 0.333... is smaller than 0.4.
Conclusion: Again, we find that 1/3 is smaller than 2/5.
Method 3: Visual Representation
Visualizing fractions can be a helpful method, particularly for beginners. You can represent each fraction using diagrams, such as circles or rectangles, divided into equal parts.
Visualizing 1/3: Draw a circle and divide it into three equal parts. Shade one part to represent 1/3.
Visualizing 2/5: Draw another circle and divide it into five equal parts. Shade two parts to represent 2/5.
By visually comparing the shaded portions, it becomes apparent that the shaded area representing 2/5 is larger than the shaded area representing 1/3.
Conclusion: This visual comparison also confirms that 1/3 is smaller than 2/5.
The Importance of Fraction Comparison
The ability to compare fractions isn't just a theoretical exercise; it's a fundamental skill with practical applications across various fields:
-
Everyday Life: Comparing prices per unit, measuring ingredients in recipes, understanding discounts, and many other daily activities involve comparing fractions.
-
Engineering and Construction: Precise measurements and calculations are essential in these fields, requiring a strong understanding of fraction comparison for accurate results. For instance, comparing tolerances in manufacturing.
-
Finance: Understanding interest rates, calculating profits and losses, and analyzing financial statements often require comparing fractions and percentages (which are essentially fractions).
-
Computer Science: In areas like image processing and data analysis, comparing fractional values is crucial for making accurate calculations and interpreting results.
-
Science: Many scientific measurements and calculations involve fractions. For instance, comparing experimental results, analyzing data from experiments, and interpreting graphs.
Beyond Simple Fractions: Extending the Concepts
The principles of fraction comparison extend to more complex fractions, including mixed numbers (numbers with a whole number part and a fractional part) and improper fractions (where the numerator is greater than or equal to the denominator). The same methods – finding a common denominator or converting to decimals – can be applied. For mixed numbers, it's often helpful to convert them to improper fractions first before applying these methods.
For example, to compare 1 1/2 and 2 1/3:
-
Convert to improper fractions: 1 1/2 = 3/2 and 2 1/3 = 7/3
-
Find a common denominator: The LCM of 2 and 3 is 6.
-
Convert to equivalent fractions: 3/2 = 9/6 and 7/3 = 14/6
-
Compare: 9/6 < 14/6, therefore 1 1/2 < 2 1/3
Conclusion: Mastering Fraction Comparison
The seemingly simple question of whether 1/3 is bigger than 2/5 highlights the importance of understanding fundamental mathematical concepts. We've explored three methods – finding a common denominator, converting to decimals, and visual representation – to effectively compare fractions. Mastering these methods is crucial not only for academic success but also for navigating various aspects of everyday life and specialized fields. Remember that consistent practice and a thorough understanding of the underlying principles will ensure proficiency in comparing fractions of all types. The ability to confidently and accurately compare fractions is a key skill in various aspects of life and a testament to a strong mathematical foundation.
Latest Posts
Latest Posts
-
What Is The Greatest Common Factor Of 28 And 70
May 09, 2025
-
How Old Is Someone If They Were Born In 1973
May 09, 2025
-
How Many Grams Are In 250 Ml
May 09, 2025
-
How Many Liters Are In 75 Milliliters
May 09, 2025
-
Which Coordinates Best Estimate The Location Of Woonsocket Ri
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is 1 3 Bigger Than 2 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.