Is 53 A Prime Or Composite Number
Arias News
Mar 28, 2025 · 5 min read
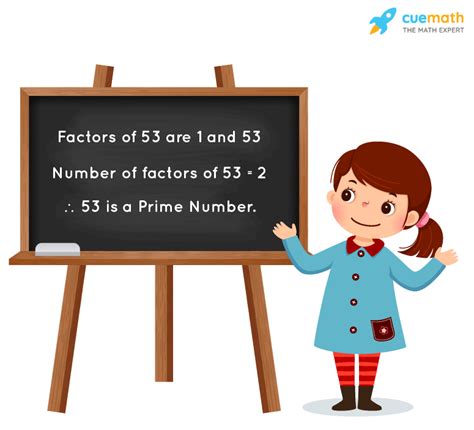
Table of Contents
Is 53 a Prime or Composite Number? A Deep Dive into Prime Numbers and Divisibility
Determining whether a number is prime or composite is a fundamental concept in number theory. This article will thoroughly explore whether 53 is a prime or composite number, providing a detailed explanation and delving into the broader concepts of prime numbers, composite numbers, and divisibility rules. We'll also touch upon the practical applications and historical significance of prime numbers.
Understanding Prime and Composite Numbers
Before we determine the nature of 53, let's establish the definitions:
Prime Number: A prime number is a whole number greater than 1 that has only two divisors: 1 and itself. This means it's only divisible by 1 and the number itself without leaving a remainder. Examples include 2, 3, 5, 7, 11, and so on.
Composite Number: A composite number is a whole number greater than 1 that has more than two divisors. In other words, it can be divided evenly by numbers other than 1 and itself. Examples include 4 (divisible by 1, 2, and 4), 6 (divisible by 1, 2, 3, and 6), 9 (divisible by 1, 3, and 9), etc.
The Number 1: The number 1 is neither prime nor composite. It's a unique case in number theory.
Determining if 53 is Prime or Composite
To determine if 53 is prime or composite, we need to check if it's divisible by any whole number other than 1 and itself. We can systematically check for divisibility by prime numbers less than the square root of 53. The square root of 53 is approximately 7.28. Therefore, we only need to test divisibility by prime numbers up to 7 (which are 2, 3, 5, and 7).
- Divisibility by 2: 53 is not divisible by 2 because it's an odd number.
- Divisibility by 3: The sum of the digits of 53 is 5 + 3 = 8. Since 8 is not divisible by 3, 53 is not divisible by 3.
- Divisibility by 5: 53 does not end in 0 or 5, so it's not divisible by 5.
- Divisibility by 7: We can perform the division: 53 ÷ 7 ≈ 7.57. This is not a whole number, meaning 53 is not divisible by 7.
Since 53 is not divisible by any prime number less than its square root, we can conclude that 53 is a prime number.
Further Verification: Sieve of Eratosthenes
A more systematic approach to finding prime numbers is using the Sieve of Eratosthenes. This ancient algorithm allows for efficiently identifying all prime numbers up to a specified limit. While manually applying it to 53 might seem excessive for this single number, it's a powerful tool for finding larger sets of primes.
The Sieve of Eratosthenes involves:
- Listing all numbers up to the desired limit (in this case, at least 53).
- Crossing out 1 (as it's neither prime nor composite).
- Starting with the first prime number, 2, cross out all multiples of 2 (except 2 itself).
- Move to the next uncrossed number (3), and cross out all multiples of 3.
- Continue this process with subsequent uncrossed numbers until you reach the square root of the limit. All remaining uncrossed numbers are prime.
Applying this method confirms that 53 remains uncrossed, further validating its prime status.
The Significance of Prime Numbers
Prime numbers hold immense significance in various fields, including:
1. Cryptography
Prime numbers are the cornerstone of modern cryptography. Public-key cryptography systems, such as RSA, rely heavily on the difficulty of factoring large numbers into their prime factors. The security of online transactions, data encryption, and secure communication protocols depends on this property of prime numbers. The larger the primes used, the more secure the encryption.
2. Number Theory and Abstract Algebra
Prime numbers are fundamental building blocks in number theory. Many theorems and concepts in this field are built upon the properties of prime numbers, their distribution, and relationships. They also play a crucial role in abstract algebra, forming the basis for various algebraic structures.
3. Computer Science and Algorithms
Prime numbers are used in various computer science algorithms and data structures. Hash tables, for example, often use prime numbers to minimize collisions and improve efficiency. Algorithms for testing primality are also an active area of research in computer science, with significant implications for cryptography and other fields.
4. Mathematics Education
Understanding prime and composite numbers is a crucial component of elementary and secondary mathematics education. It builds a foundation for more advanced mathematical concepts, developing logical reasoning and problem-solving skills.
Identifying Prime Numbers: Practical Tips and Tricks
While determining whether small numbers like 53 are prime is relatively straightforward, identifying larger numbers requires more sophisticated techniques. Here are some helpful tips and considerations:
-
Trial Division: While effective for smaller numbers, trial division becomes computationally expensive for large numbers. It involves systematically checking for divisibility by all primes up to the square root of the number.
-
Probabilistic Primality Tests: For larger numbers, probabilistic tests like the Miller-Rabin test are more efficient. These tests don't guarantee primality with 100% certainty but provide a high probability of correctness.
-
Deterministic Primality Tests: Algorithms like the AKS primality test provide a definitive answer but are generally slower than probabilistic tests.
-
Using Online Prime Number Checkers: Many websites and calculators can efficiently determine whether a number is prime. These often utilize optimized algorithms to handle large numbers effectively.
Conclusion
In conclusion, 53 is a prime number. Its indivisibility by any number other than 1 and itself confirms its status as a prime. Understanding prime numbers and their properties is essential not only for a deeper understanding of mathematics but also for appreciating their vital role in various fields, particularly cryptography and computer science. The exploration of prime numbers continues to be a fascinating and active area of mathematical research, with ongoing discoveries and advancements shaping our understanding of numbers and their profound implications. The simple question of whether 53 is prime opens the door to a vast and intricate world of mathematical concepts and applications.
Latest Posts
Latest Posts
-
How Much Does 5 Gallons Of Concrete Weigh
Mar 31, 2025
-
What Is The Opposite Of An Inverse Relationship
Mar 31, 2025
-
5 Letter Word With Y As Second Letter
Mar 31, 2025
-
How Many Days Is 5 Million Seconds
Mar 31, 2025
-
What Do You Call A Female Bug That Floats
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Is 53 A Prime Or Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
