Is Square Root Of 15 A Rational Number
Arias News
May 08, 2025 · 5 min read
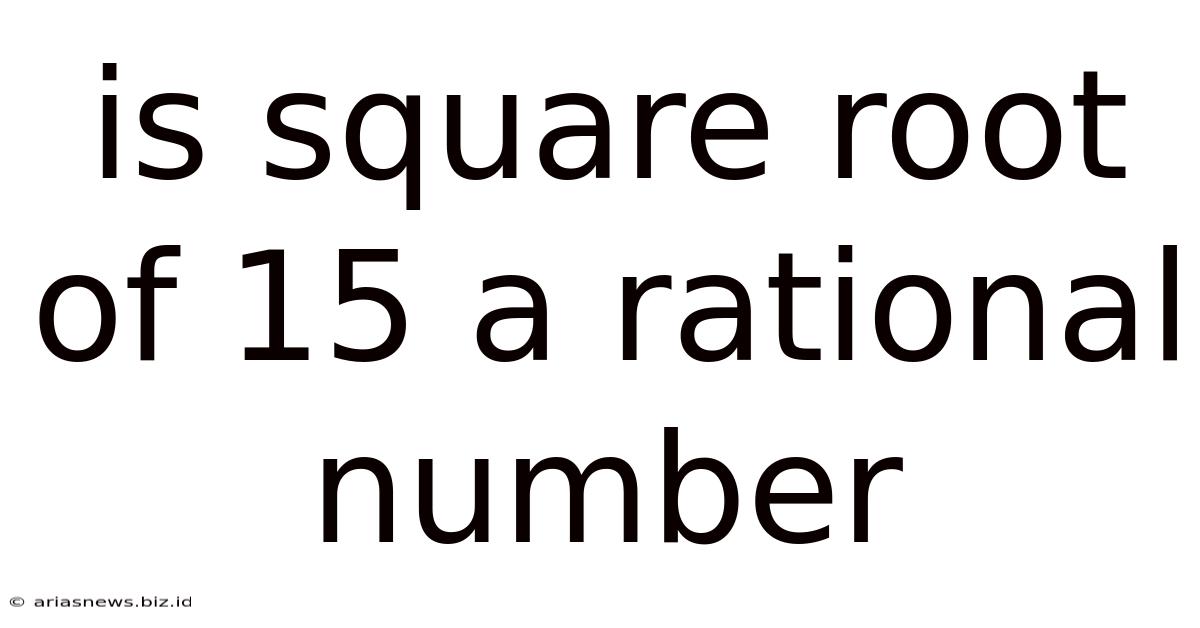
Table of Contents
Is the Square Root of 15 a Rational Number? A Deep Dive into Irrationality
The question of whether the square root of 15 is a rational number is a fundamental one in mathematics, touching upon the core concepts of number theory and algebra. Understanding this requires a grasp of what constitutes a rational number and the properties of square roots. This article will explore this question in detail, providing a clear and comprehensive explanation accessible to a broad audience, including those with a limited mathematical background. We'll delve into the proof, explore related concepts, and highlight the broader implications of this seemingly simple problem.
Understanding Rational and Irrational Numbers
Before we tackle the square root of 15, let's define our terms. A rational number is any number that can be expressed as a fraction p/q, where p and q are integers (whole numbers), and q is not zero. Examples include 1/2, 3/4, -5/7, and even whole numbers like 5 (which can be expressed as 5/1).
An irrational number, conversely, cannot be expressed as a fraction of two integers. These numbers have decimal representations that are non-terminating (they don't end) and non-repeating (they don't have a pattern that repeats indefinitely). Famous examples include π (pi), e (Euler's number), and the square root of 2 (√2).
Proving the Irrationality of √15
To determine if √15 is rational or irrational, we'll use a method of proof by contradiction. This method assumes the opposite of what we want to prove and then shows that this assumption leads to a contradiction, thus proving the original statement.
1. The Assumption: Let's assume, for the sake of contradiction, that √15 is a rational number. This means it can be expressed as a fraction p/q, where p and q are integers, q ≠ 0, and the fraction is in its simplest form (meaning p and q share no common factors other than 1).
2. Squaring Both Sides: If √15 = p/q, then squaring both sides gives us:
15 = p²/q²
3. Rearranging the Equation: Multiplying both sides by q² gives:
15q² = p²
This equation tells us that p² is a multiple of 15. Since 15 = 3 x 5, this means p² must be divisible by both 3 and 5. A number is divisible by 3 if the sum of its digits is divisible by 3, and a number is divisible by 5 if it ends in 0 or 5.
4. Implication for p: If p² is divisible by 3, then p itself must also be divisible by 3 (this is a property of prime factorization). We can express this as p = 3k, where k is another integer.
5. Substituting and Simplifying: Substituting p = 3k into the equation 15q² = p², we get:
15q² = (3k)²
15q² = 9k²
Dividing both sides by 3 gives:
5q² = 3k²
6. Implication for q: This equation shows that 3k² is a multiple of 5. Similarly, following the same logic as before, this means k² must be divisible by 5, and consequently, k must also be divisible by 5.
7. The Contradiction: We've now shown that both p and q are divisible by 3 and 5. This contradicts our initial assumption that p/q is in its simplest form (that p and q share no common factors). Since our assumption leads to a contradiction, it must be false.
8. Conclusion: Therefore, our initial assumption that √15 is rational is false. This proves that √15 is an irrational number.
Exploring Related Concepts
Understanding the irrationality of √15 opens doors to exploring several related mathematical concepts:
-
Perfect Squares: The proof hinges on the fact that 15 is not a perfect square (a number that is the square of an integer). Only the square roots of perfect squares are rational.
-
Prime Factorization: The prime factorization of 15 (3 x 5) plays a crucial role in the proof. The properties of prime numbers are fundamental to number theory.
-
Proof by Contradiction: This is a powerful method of proof used extensively in mathematics to establish the truth of a statement by showing that its opposite leads to a contradiction.
-
Continued Fractions: Irrational numbers can be represented as continued fractions, offering another way to understand their non-repeating, non-terminating decimal expansions. The continued fraction representation of √15 provides further insight into its irrationality.
The Significance of Irrational Numbers
The existence of irrational numbers like √15 challenges our intuitive understanding of numbers. While we can approximate √15 with decimal values (approximately 3.873), we can never express it precisely as a fraction. This highlights the richness and complexity of the number system. Irrational numbers are essential in many areas of mathematics and its applications, including:
-
Geometry: Irrational numbers are ubiquitous in geometric calculations, especially when dealing with circles (π) and diagonals of squares (√2).
-
Calculus: Many fundamental concepts in calculus rely on irrational numbers, including limits and derivatives.
-
Physics: Irrational numbers appear in various physical constants and equations.
Practical Implications and Further Exploration
While the theoretical implications of irrational numbers are significant, their practical applications are often less obvious. However, understanding their nature is vital for accurately modeling and solving problems in various fields. For instance, in engineering and construction, understanding the limitations of using rational approximations for irrational numbers is crucial for precision.
This detailed exploration of the irrationality of √15 has provided not only a proof but also a deeper understanding of rational and irrational numbers, their properties, and their significance in mathematics and its applications. Further exploration could delve into the different methods of approximating irrational numbers, their representation in different number systems, and their role in more advanced mathematical concepts. The seemingly simple question of whether √15 is rational unveils a rich tapestry of mathematical ideas that continue to fascinate and challenge mathematicians and learners alike.
Latest Posts
Latest Posts
-
How Many Quarts Are In A 2 Liter
May 08, 2025
-
You Are The Potter I Am The Clay Song
May 08, 2025
-
Algebra With Pizzazz Did You Hear About
May 08, 2025
-
What Year Were You Born If You Re 18
May 08, 2025
-
A Trapezoid Always Has Two Congruent Sides
May 08, 2025
Related Post
Thank you for visiting our website which covers about Is Square Root Of 15 A Rational Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.