Polygon With 5 Sides And 1 Right Angle
Arias News
Apr 04, 2025 · 6 min read
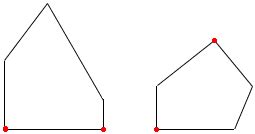
Table of Contents
Exploring the Enigmatic Pentagon with One Right Angle
A pentagon, a five-sided polygon, is a geometric shape rich in mathematical properties and possibilities. While regular pentagons, with their equal sides and angles, are relatively well-understood, exploring pentagons with specific constraints, like the presence of a single right angle, unveils a fascinating realm of geometric complexity and unique characteristics. This article delves deep into the properties, construction, and potential applications of a pentagon possessing exactly one right angle. We will uncover the intricacies of its angles, side lengths, area calculations, and even touch upon its relevance in various fields.
Defining the Problem: The One-Right-Angled Pentagon
The challenge lies in understanding the implications of a single right angle within a five-sided polygon. Unlike a rectangle or square where right angles dictate specific properties, the introduction of a right angle into a pentagon significantly alters its characteristics. This means we can't rely on simple formulas for regular polygons. Instead, we must employ a more nuanced approach, drawing upon trigonometric principles and geometric constructions.
The existence of one right angle immediately suggests that the pentagon is irregular. This irregular nature implies that the lengths of its sides will vary, and its interior angles (excluding the right angle) will also be unequal and non-90 degrees. This variability introduces a significant degree of freedom, making the analysis more intricate yet far more interesting.
Possible Configurations and Constraints
Because of its irregularity, the pentagon with one right angle can exist in numerous configurations. The location of the right angle greatly influences the shape and proportions of the pentagon. Imagine, for instance, the right angle positioned at one of the vertices, with the other angles and sides adjusting accordingly. We could also envision scenarios where the right angle is "internal," formed by the intersection of two non-adjacent sides.
The crucial constraint remains the presence of exactly one right angle. This restriction significantly narrows the possible configurations while still allowing for a large variety of shapes and sizes.
Calculating Angles and Side Lengths
Determining the angles and side lengths of a pentagon with one right angle demands a more detailed approach. We can't directly use standard pentagon formulas. Instead, we must use the following:
-
Sum of Interior Angles: The sum of interior angles in any polygon with 'n' sides is given by the formula (n-2) * 180°. For a pentagon (n=5), the sum is (5-2) * 180° = 540°. Since one angle is 90°, the remaining four angles must add up to 540° - 90° = 450°.
-
Trigonometric Relationships: Once we have one angle and a pair of sides, we can utilize trigonometric functions (sine, cosine, tangent) to calculate other angles and side lengths, especially within the triangles formed by the diagonals of the pentagon.
-
Geometric Construction: Constructing the pentagon using geometric tools like compass and straightedge is also a valuable approach to visualize and solve specific cases. Starting with the right angle and adding sides and angles according to constraints, we can obtain various forms of such a pentagon.
However, it's crucial to note that a unique solution is not guaranteed. The single constraint of one right angle leaves enough flexibility for infinitely many unique pentagons to exist. To obtain a specific solution, we need further constraints like side lengths or other angles.
Area Calculation: The Challenge of Irregularity
Calculating the area of a regular polygon is straightforward. However, determining the area of an irregular pentagon with one right angle presents a much greater challenge. There's no single formula, and the approach varies depending on the available information. We can use several methods:
-
Triangulation: Divide the pentagon into triangles using diagonals. Calculate the area of each triangle individually using the formula ½ * base * height, then add up the areas. This requires knowing the lengths of the necessary sides and possibly some angles.
-
Coordinate Geometry: If we assign Cartesian coordinates to the vertices of the pentagon, we can use the shoelace formula (also known as Gauss's area formula) to calculate the area. This formula provides a direct computation based on the coordinates.
-
Using Trigonometry: If we know sufficient angles and side lengths, we can leverage trigonometric identities to calculate the area. This method usually involves breaking down the pentagon into smaller, easily solvable triangles.
Applications and Real-World Examples
Although less common than regular polygons, pentagons with one right angle can appear in various unexpected situations:
-
Architectural Design: Irregular pentagonal shapes can be incorporated into building designs for aesthetic or structural reasons. The unique angles could allow for interesting light play or fit specific spatial constraints.
-
Engineering Applications: In engineering, creating custom components or solving geometric problems might involve irregular polygons. The design of certain mechanical parts or structural elements might unexpectedly result in such a shape.
-
Computer Graphics and Game Development: Generating realistic-looking landscapes or environments in computer graphics often uses irregularly shaped polygons. These polygons can represent complex terrain features or objects with unusual geometries.
-
Tessellations and Pattern Design: While not as straightforward as with regular shapes, manipulating irregular pentagons can still lead to interesting and complex tessellations or patterns in artistic or design contexts.
Advanced Considerations: Mathematical Exploration
For those interested in deeper mathematical exploration, we can consider the following:
-
Mathematical Proof: Proving certain relationships between angles and side lengths within the pentagon requires careful application of geometric theorems and trigonometric identities.
-
Optimization Problems: This involves finding pentagons with a specific right angle that satisfy other conditions, such as minimizing or maximizing area for given side lengths.
-
Relationship to other Geometric Shapes: The pentagon with one right angle can be explored in relation to other shapes, like quadrilaterals or other irregular polygons, to uncover any shared properties or transformations.
-
Generalized Approach: Developing a generalized algorithm or formula that can handle various cases of pentagons with one right angle remains a challenging but fascinating pursuit.
Conclusion: A Journey into Geometric Complexity
The pentagon with one right angle, despite its simple initial definition, proves to be a complex and fascinating geometric entity. Its irregularity allows for a wide variety of shapes and sizes, requiring sophisticated mathematical techniques to fully analyze its properties. Through triangulation, trigonometric calculations, or coordinate geometry, we can approach its area and other characteristics. Understanding these types of irregular polygons expands our grasp of geometry, highlighting the versatility and mathematical richness that lies beyond the familiar regular shapes. Further research into its properties could lead to unexpected applications in various scientific and artistic fields. The journey into understanding this intriguing polygon is far from over, promising a continuous source of geometric exploration and discovery.
Latest Posts
Latest Posts
-
How Many Grams In A Kilogram Of Gold
Apr 05, 2025
-
How Many Miles In A 6k Run
Apr 05, 2025
-
How Big Is 31 Cm In Inches
Apr 05, 2025
-
How Long Is 90 Days In Months And Weeks
Apr 05, 2025
-
A Brief Vacation As In A Weekend
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Polygon With 5 Sides And 1 Right Angle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
