Square Root Of 0.52 As A Fraction
Arias News
Apr 05, 2025 · 4 min read
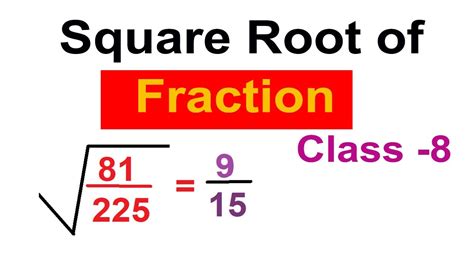
Table of Contents
The Square Root of 0.52 as a Fraction: A Deep Dive
Finding the square root of a decimal like 0.52 and expressing it as a fraction might seem daunting at first, but it's a straightforward process involving a combination of algebraic manipulation, simplification, and potentially approximation techniques. This article provides a comprehensive guide, exploring various approaches and highlighting the nuances involved. We'll delve into the exact method, discuss approximation methods for practical purposes, and examine the implications for different applications.
Understanding the Problem: √0.52 as a Fraction
The core challenge lies in converting the decimal 0.52 into a fraction before calculating its square root. This avoids working directly with decimals within the square root calculation, which can often lead to cumbersome or less precise results. We aim to find a fraction x/y such that (x/y)² = 0.52.
Method 1: Converting Decimal to Fraction
The first step is transforming 0.52 into a fraction. This involves recognizing that 0.52 represents 52 hundredths. Therefore:
0.52 = 52/100
This fraction can be simplified by finding the greatest common divisor (GCD) of 52 and 100. Both numbers are divisible by 4:
52/100 = (52 ÷ 4) / (100 ÷ 4) = 13/25
Now our problem becomes finding the square root of 13/25:
√(13/25)
Method 2: Simplifying the Square Root
The square root of a fraction is simply the square root of the numerator divided by the square root of the denominator:
√(13/25) = √13 / √25
Since √25 = 5, this simplifies to:
√13 / 5
Method 3: Approximating the Square Root of 13
Unfortunately, √13 is an irrational number—it cannot be expressed as a simple fraction. It has an infinite, non-repeating decimal representation. To express √0.52 as a fraction, we need to approximate √13.
There are several methods for approximating square roots:
-
Babylonian Method (or Heron's Method): This iterative method refines an initial guess to progressively closer approximations of the square root.
-
Linear Approximation: This simpler method uses the tangent line of the square root function at a nearby known perfect square to estimate the square root.
-
Calculator/Software: The most straightforward method is using a calculator or mathematical software to find a decimal approximation of √13.
Let's assume we use a calculator and obtain the approximation √13 ≈ 3.60555.
Method 4: Combining and Expressing as a Fraction
Substituting our approximation into the expression from Method 2:
√13 / 5 ≈ 3.60555 / 5 ≈ 0.72111
To express this decimal approximation as a fraction, we can convert it using the same principle as before:
0.72111 ≈ 72111/100000
This fraction is an approximation of √0.52. The accuracy depends on the precision of the approximation used for √13.
Exploring Different Approximation Levels
The accuracy of our fractional representation hinges on the precision of the √13 approximation. Let's explore a few scenarios:
Scenario 1: Using a less precise approximation: If we approximated √13 as 3.6, our calculation would be:
3.6 / 5 = 0.72 = 72/100 = 18/25
This is a less accurate but simpler fraction.
Scenario 2: Using a more precise approximation: Using a more precise approximation of √13, like 3.60555, leads to a more accurate (but more complex) fraction.
Scenario 3: Continued Fractions: A more sophisticated approach involves using continued fractions to represent irrational numbers like √13. This provides a series of increasingly accurate rational approximations. This method is beyond the scope of a basic explanation but is worth noting for advanced mathematical exploration.
Implications and Applications
Understanding how to represent the square root of 0.52 as a fraction is relevant in various contexts:
-
Geometric Calculations: In geometry, you might encounter situations where calculating the length of a diagonal or the area of a figure requires the square root of a decimal. Expressing this as a fraction might simplify further calculations or provide a more easily understood representation.
-
Engineering and Physics: Many engineering and physics problems involve calculations with square roots. Depending on the required precision, using fractional approximations might suffice, offering a more manageable form for analysis and design.
-
Computer Programming: When working with computer programs, representing numbers as fractions can sometimes offer better accuracy or efficiency in certain algorithms, especially those dealing with rational numbers.
Conclusion: A Multifaceted Approach
Finding the exact fractional representation of √0.52 is impossible because √13 is irrational. However, by converting the decimal to a fraction and approximating the square root, we can obtain increasingly accurate fractional representations. The choice of approximation method depends on the required level of precision and the practical context of the problem. Remembering the fundamental principles of decimal-to-fraction conversion and square root simplification will allow you to tackle similar problems effectively. The method outlined here provides a solid foundation for understanding and applying these concepts in various mathematical and real-world scenarios. Further exploration into advanced approximation techniques like continued fractions would yield even more accurate and insightful representations.
Latest Posts
Latest Posts
-
Diagonal Lines On The Pavement Warn Drivers Of
Apr 05, 2025
-
How Many Cups Is 200 Ml Water
Apr 05, 2025
-
Is 79 A Prime Or Composite Number
Apr 05, 2025
-
What Is One Of The Characteristics Of A Strong Theme
Apr 05, 2025
-
How Many Cups In 1 Pound Of Macaroni
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Square Root Of 0.52 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
