The Difference Of A Number And 5
Arias News
May 08, 2025 · 5 min read
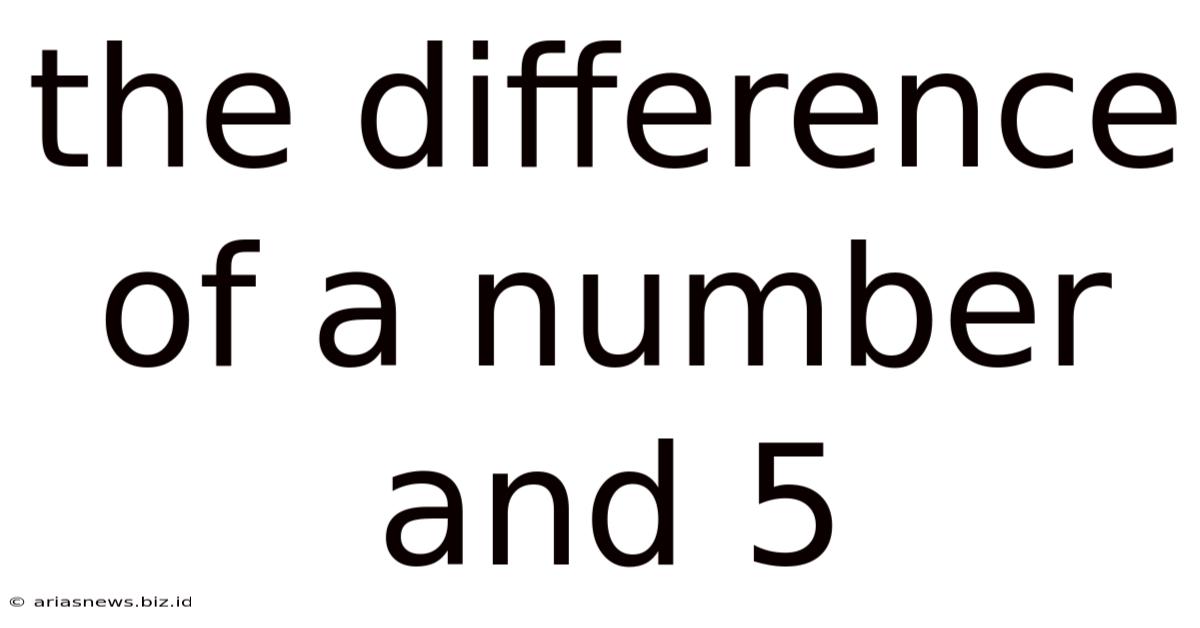
Table of Contents
The Fascinating World of Numbers: Exploring the Difference Between a Number and 5
The seemingly simple concept of "the difference between a number and 5" opens a surprisingly vast and fascinating world of mathematical exploration. This seemingly basic arithmetic operation underpins countless applications across various fields, from elementary school arithmetic to advanced calculus and beyond. This article delves deep into this concept, exploring its various interpretations, applications, and implications across different mathematical contexts. We'll explore how this seemingly simple operation forms the foundation for more complex mathematical ideas.
Understanding the Fundamental Concept
At its core, the difference between a number and 5 is simply the result of subtracting 5 from that number. If we represent the number as 'x', the expression becomes x - 5. This difference can be positive, negative, or zero, depending on the value of 'x'.
-
Positive Difference: If x > 5, the difference (x - 5) will be a positive number. For example, if x = 10, then x - 5 = 5. This indicates that x is 5 units greater than 5.
-
Negative Difference: If x < 5, the difference (x - 5) will be a negative number. For instance, if x = 2, then x - 5 = -3. This means x is 3 units less than 5.
-
Zero Difference: If x = 5, the difference (x - 5) will be 0. This signifies that x is equal to 5.
Visualizing the Difference
Imagine a number line. The number 5 serves as a reference point. The difference between any other number and 5 represents the distance between that number and 5 on the number line. A positive difference indicates a number to the right of 5, while a negative difference points to a number to the left. This visual representation simplifies the understanding of the concept, especially for beginners.
Applications Across Different Mathematical Domains
The seemingly simple operation of finding the difference between a number and 5 has far-reaching applications across numerous mathematical domains:
1. Elementary Arithmetic and Algebra
This forms the foundation of basic arithmetic operations. Students learn to calculate differences, apply it to word problems, and gradually progress to solving algebraic equations involving this concept. For example, solving an equation like x - 5 = 10 involves finding the value of x which, when reduced by 5, gives 10. The answer is easily derived by adding 5 to both sides of the equation (x = 15).
2. Number Theory
In number theory, the difference between a number and 5 plays a role in various concepts, such as modular arithmetic. For example, considering numbers modulo 5 means examining their remainders when divided by 5. The difference between a number and 5 is directly related to its remainder when divided by 5.
3. Calculus and Analysis
While seemingly basic, the concept of a difference forms the foundation of calculus. The derivative of a function at a point is essentially the instantaneous rate of change, calculated by considering the difference in function values over increasingly small intervals. Understanding differences is a crucial stepping stone towards mastering more advanced concepts in calculus.
4. Statistics and Probability
In statistics, finding the difference between a number and a mean (average) is frequently used to calculate deviations from the mean. This helps determine the spread or variability of data. In probability, understanding differences is vital for determining the probability of events occurring within specific ranges.
5. Linear Algebra
In linear algebra, the difference between a number and 5 can be applied to vector operations and matrix manipulations. The concept underlies the idea of vector subtraction, where the difference between two vectors is computed element-wise.
Exploring Different Scenarios and Examples
Let's explore some real-world scenarios where the difference between a number and 5 plays a significant role:
1. Temperature Differences
Suppose the temperature outside is 20°C. The difference between this temperature and 5°C is 15°C (20 - 5 = 15), indicating the temperature is 15°C warmer than 5°C.
2. Financial Transactions
Imagine you have $10 in your account. After withdrawing $5, the difference between your initial balance and the amount withdrawn is $5. This illustrates a simple subtraction calculation.
3. Measuring Distances
Consider two points on a map, one located at 5km and another at 12km. The difference between their distances (12 - 5 = 7) is 7km. This shows a practical application in spatial measurements.
Advanced Concepts and Extensions
The core concept can be extended to more complex scenarios:
1. Absolute Difference
The absolute difference between a number and 5 is the magnitude of the difference, regardless of its sign. It's represented as |x - 5|. This ensures the result is always non-negative. For example, the absolute difference between 2 and 5 is |2 - 5| = |-3| = 3.
2. Percentage Difference
The percentage difference between a number and 5 is calculated by dividing the absolute difference by 5 and multiplying by 100. This expresses the difference as a percentage of 5. For example, the percentage difference between 10 and 5 is [(10 - 5) / 5] * 100 = 100%.
3. Generalization to Other Numbers
The concept can be generalized beyond 5. The difference between any two numbers, 'a' and 'b', is simply 'a - b'. This highlights the versatility of the fundamental principle of subtraction.
Conclusion: The Enduring Importance of a Simple Concept
While seemingly simple, the difference between a number and 5 is a fundamental concept that permeates various mathematical branches. Its applications extend far beyond elementary arithmetic, touching upon complex areas like calculus, statistics, and linear algebra. Understanding this seemingly basic operation lays a crucial foundation for grasping more advanced mathematical concepts and applying mathematical principles to real-world scenarios. The exploration of this concept serves as a powerful reminder of how seemingly simple ideas can have profound and far-reaching implications across the mathematical landscape. The continuing relevance of this concept underscores its fundamental importance in the study of numbers and mathematics in general. Mastering this fundamental concept sets the stage for a deeper appreciation and understanding of the broader mathematical world.
Latest Posts
Latest Posts
-
How Many Pounds Is A Quarter Of A Ton
May 08, 2025
-
The Shapes Of The Horizontal Cross Sections Of The Cone Below
May 08, 2025
-
How Much Does A Georgia Bulldog Cost
May 08, 2025
-
What Is A 51 Out Of 60
May 08, 2025
-
What Is A 65 Out Of 80
May 08, 2025
Related Post
Thank you for visiting our website which covers about The Difference Of A Number And 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.