What Are The Common Factors Of 24 And 40
Arias News
Apr 02, 2025 · 5 min read
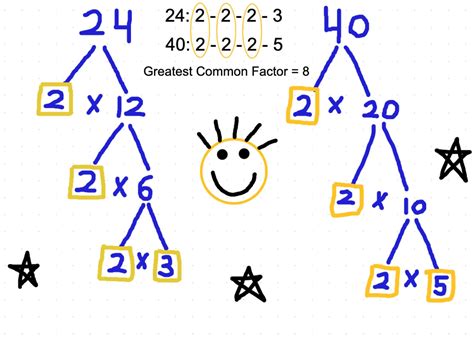
Table of Contents
Unveiling the Secrets of Common Factors: A Deep Dive into 24 and 40
Finding the common factors of two numbers might seem like a simple arithmetic exercise, but it opens a gateway to understanding fundamental concepts in number theory, paving the way for more advanced mathematical explorations. This comprehensive guide delves into the common factors of 24 and 40, exploring various methods to find them, their significance in mathematics, and practical applications. We'll go beyond a simple answer, providing a robust understanding of the underlying principles.
Understanding Factors and Common Factors
Before diving into the specifics of 24 and 40, let's solidify our understanding of key terminology.
Factors: A factor of a number is any whole number that divides the number evenly without leaving a remainder. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12.
Common Factors: When considering two or more numbers, common factors are the numbers that are factors of all the given numbers. For example, the common factors of 12 and 18 are 1, 2, 3, and 6.
Method 1: Prime Factorization – Unveiling the Building Blocks
Prime factorization is a powerful technique to determine the factors of a number. It involves expressing a number as a product of its prime factors – numbers divisible only by 1 and themselves (e.g., 2, 3, 5, 7, 11...).
Let's apply this to 24 and 40:
Prime Factorization of 24:
24 = 2 x 2 x 2 x 3 = 2³ x 3
Prime Factorization of 40:
40 = 2 x 2 x 2 x 5 = 2³ x 5
Now, to find the common factors, we look for the prime factors that appear in both factorizations. In this case, we see that both 24 and 40 share three factors of 2.
Identifying Common Factors from Prime Factorization:
From the prime factorizations, we can derive the common factors:
- 2¹ (or 2): Since both numbers have at least one 2.
- 2² (or 4): Since both numbers have at least two 2s.
- 2³ (or 8): Since both numbers have at least three 2s.
- 1: Every number has 1 as a factor.
Therefore, the common factors of 24 and 40 are 1, 2, 4, and 8.
Method 2: Listing Factors – A Systematic Approach
This method involves listing all the factors of each number and then identifying the common ones. While seemingly less elegant than prime factorization, it's a straightforward approach, especially for smaller numbers.
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
Factors of 40: 1, 2, 4, 5, 8, 10, 20, 40
By comparing the two lists, we see that the common factors are 1, 2, 4, and 8.
Method 3: Using the Greatest Common Divisor (GCD) – Efficiency at its Finest
The greatest common divisor (GCD), also known as the greatest common factor (GCF), is the largest number that divides both numbers without leaving a remainder. Finding the GCD provides a shortcut to finding all common factors. There are several ways to calculate the GCD:
-
Euclidean Algorithm: This efficient algorithm repeatedly applies division with remainder until the remainder is zero. The last non-zero remainder is the GCD.
-
Listing Factors (as above): The largest number that appears in both lists is the GCD.
-
Prime Factorization: The GCD is the product of the common prime factors raised to the lowest power.
Let’s apply the Euclidean algorithm:
- Divide 40 by 24: 40 = 24 x 1 + 16
- Divide 24 by 16: 24 = 16 x 1 + 8
- Divide 16 by 8: 16 = 8 x 2 + 0
The last non-zero remainder is 8, so the GCD of 24 and 40 is 8.
Once we have the GCD, we know that all common factors will be divisors of the GCD. Therefore, the common factors of 24 and 40 are 1, 2, 4, and 8 (the divisors of 8).
The Significance of Common Factors
Understanding common factors has broad implications across various mathematical fields and practical applications:
-
Simplifying Fractions: Finding the GCD is crucial for simplifying fractions to their lowest terms. For instance, the fraction 24/40 can be simplified to 3/5 by dividing both the numerator and the denominator by their GCD (8).
-
Solving Equations: In algebra, finding common factors helps in factoring polynomials and solving equations.
-
Modular Arithmetic: Common factors play a significant role in modular arithmetic, which has applications in cryptography and computer science.
-
Geometry: Common factors are used in determining the dimensions of shapes and objects that can be tiled or arranged in a regular pattern. Imagine tiling a floor with square tiles – the dimensions of the tiles will need to be a common factor of the room's dimensions.
-
Scheduling and Logistics: In scheduling problems, the least common multiple (LCM) – which is closely related to the GCD – helps determine the time interval when events will coincide. For instance, if two machines have cycle times of 24 and 40 units, the LCM (which is 120) gives the time when they will both complete their cycles simultaneously.
Beyond the Basics: Exploring Least Common Multiples (LCM)
While we've focused on common factors, it's essential to briefly discuss the least common multiple (LCM). The LCM is the smallest number that is a multiple of both given numbers. The GCD and LCM are intimately related:
GCD(a, b) x LCM(a, b) = a x b
Therefore, knowing the GCD allows for the easy calculation of the LCM:
LCM(24, 40) = (24 x 40) / GCD(24, 40) = (24 x 40) / 8 = 120
The LCM is crucial in various applications, including:
- Finding the smallest common denominator when adding or subtracting fractions.
- Determining cycles in repeating patterns.
- Solving problems involving timing and synchronization.
Conclusion: A Foundation for Further Exploration
Finding the common factors of 24 and 40, seemingly a straightforward task, provides a stepping stone to grasping deeper mathematical concepts. Through prime factorization, listing factors, and utilizing the GCD, we've explored various methods to identify these common factors (1, 2, 4, and 8). The understanding of these methods and the significance of common factors and LCMs extends far beyond basic arithmetic, forming a crucial foundation for advanced mathematical studies and real-world applications across numerous fields. This exploration encourages a deeper appreciation for the interconnectedness and elegance of mathematical principles. The journey to mastering these concepts is a continuous process of learning and applying, further solidifying one's mathematical understanding.
Latest Posts
Latest Posts
-
How Many Books Of The Bible Are Named After Women
Apr 03, 2025
-
What Is The Gcf Of 14 35
Apr 03, 2025
-
Is Jeff Allen Related To Tim Allen
Apr 03, 2025
-
How Many Seconds Is In 9 Minutes
Apr 03, 2025
-
Is Ricky Van Shelton Related To Blake Shelton
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Are The Common Factors Of 24 And 40 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
