What Are The Factor Pairs For 15
Arias News
May 11, 2025 · 5 min read
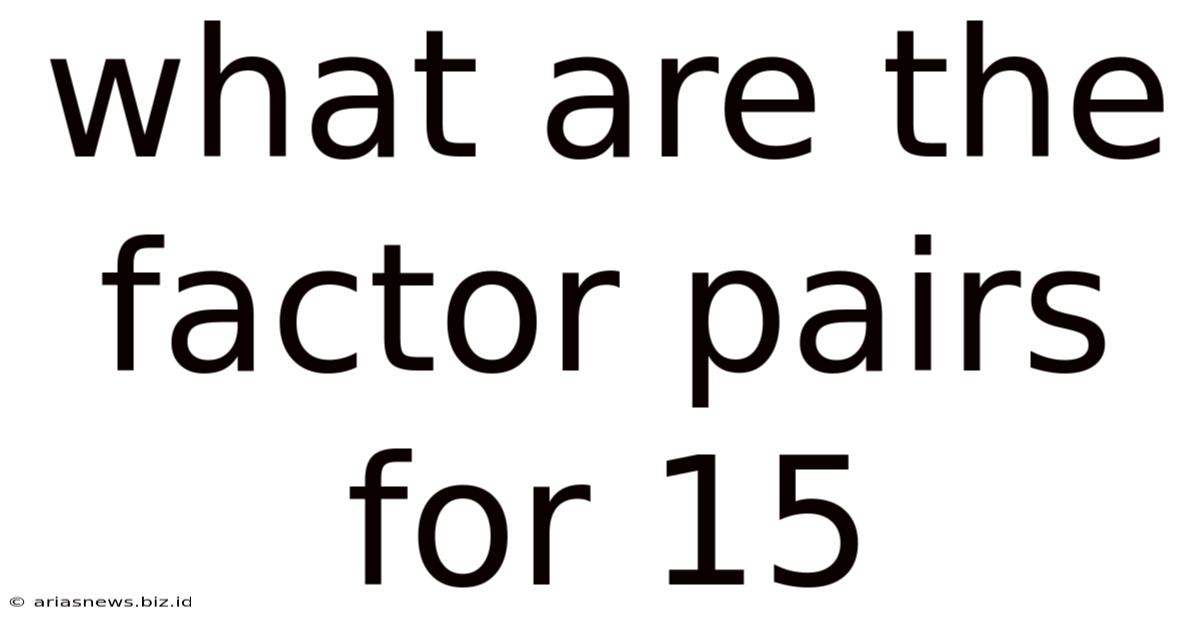
Table of Contents
What Are the Factor Pairs for 15? A Deep Dive into Number Theory
Finding the factor pairs for a number might seem like a simple task, especially for a smaller number like 15. However, understanding this concept thoroughly unlocks a deeper understanding of number theory, prime factorization, and even more complex mathematical concepts. This article will explore the factor pairs of 15 in detail, examining the underlying principles and providing a comprehensive overview suitable for students and enthusiasts alike.
Understanding Factors and Factor Pairs
Before diving into the specifics of 15, let's define key terms. A factor of a number is a whole number that divides evenly into that number without leaving a remainder. In other words, if 'a' is a factor of 'b', then b/a results in a whole number.
A factor pair consists of two factors that, when multiplied together, produce the original number. These pairs are crucial for understanding the composition of a number and its relationship to other numbers within the number system.
Finding the Factor Pairs of 15
Let's apply this to the number 15. To find its factor pairs, we need to identify all the whole numbers that divide evenly into 15. We can start by systematically checking:
- 1: 15 divided by 1 is 15. This gives us our first factor pair: (1, 15).
- 3: 15 divided by 3 is 5. This gives us our second factor pair: (3, 5).
- 5: 15 divided by 5 is 3. Notice that this pair is the same as (3,5), just reversed. This illustrates an important point: factor pairs are often presented as ordered pairs, but the order doesn't change the fundamental relationship.
- 15: 15 divided by 15 is 1. Again, this pair is the same as (1,15).
Therefore, the complete set of factor pairs for 15 is (1, 15) and (3, 5). There are no other whole numbers that divide evenly into 15.
Prime Factorization and its Relation to Factor Pairs
The concept of prime factorization is intrinsically linked to finding factor pairs. A prime number is a whole number greater than 1 that has only two factors: 1 and itself. Prime factorization involves expressing a number as the product of its prime factors.
Let's find the prime factorization of 15:
15 can be divided by 3 and 5, both of which are prime numbers. Therefore, the prime factorization of 15 is 3 x 5. This prime factorization directly informs us about its factor pairs. Each prime factor, or a combination of them, can be paired with another factor to create a factor pair. In this case:
- 3 paired with 5 gives (3,5)
- 1 (implied, as 1 is a factor of every number) paired with 15 gives (1,15)
Extending the Concept: Factor Pairs of Larger Numbers
Understanding how to find factor pairs for smaller numbers like 15 lays the foundation for tackling larger numbers. Let's consider a slightly more complex example, such as the number 24:
To find the factor pairs of 24, we systematically check the whole numbers:
- 1 x 24 = 24 => (1, 24)
- 2 x 12 = 24 => (2, 12)
- 3 x 8 = 24 => (3, 8)
- 4 x 6 = 24 => (4, 6)
- 6 x 4 = 24 => (6,4) - This is a repeat, mirroring (4,6).
The factor pairs of 24 are (1,24), (2,12), (3,8), and (4,6). Notice that as the numbers increase in complexity, the number of factor pairs also increases.
Applications of Factor Pairs
The seemingly simple concept of factor pairs has significant applications in various areas of mathematics and beyond:
- Simplifying Fractions: Finding the greatest common factor (GCF) of the numerator and denominator relies heavily on identifying factor pairs. This allows for simplification of fractions to their lowest terms. For example, simplifying 15/25 requires finding the GCF of 15 and 25, which is 5.
- Algebraic Factoring: In algebra, factoring expressions involves breaking down polynomials into simpler components, which often relies on understanding factor pairs and prime factorization.
- Number Theory: Factor pairs are fundamental to many number theory concepts, such as perfect numbers, abundant numbers, and deficient numbers. A perfect number is a number where the sum of its proper divisors (excluding itself) equals the number itself. Understanding factor pairs helps to identify such numbers.
- Cryptography: Factorization of large numbers into their prime factors plays a crucial role in modern cryptography. The difficulty of factoring large numbers forms the basis of widely used encryption algorithms.
Visualizing Factor Pairs: Factor Trees
A useful tool for understanding and visualizing the process of finding factor pairs, particularly for larger numbers, is the factor tree. A factor tree breaks down a number into its prime factors through a branching diagram. Let’s create a factor tree for 24:
24
/ \
2 12
/ \
2 6
/ \
2 3
This tree shows that the prime factorization of 24 is 2 x 2 x 2 x 3 (or 2³ x 3).
Conclusion: The Importance of Understanding Factor Pairs
While seemingly basic, the concept of factor pairs provides a robust foundation for understanding more complex mathematical principles. The ability to efficiently find and utilize factor pairs is crucial in various mathematical applications, extending beyond simple arithmetic to encompass advanced areas like algebra, number theory, and cryptography. By mastering this fundamental concept, individuals gain a deeper appreciation for the structure and relationships within the number system. The factor pairs for 15, (1,15) and (3,5), serve as a simple yet powerful illustration of these broader mathematical principles. This understanding proves vital for continued exploration and success in higher-level mathematics and related fields.
Latest Posts
Latest Posts
-
Can A Linear Equation Have An Exponent
May 12, 2025
-
Carve Your Name On Your Heart And Not On Marble
May 12, 2025
-
How Many Ounces Is 2 Pounds 7 Ounces Of Butter
May 12, 2025
-
How Long Is Velveeta Good For After Expiration Date
May 12, 2025
-
Who Accepts Wells Fargo Home Projects Card
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Are The Factor Pairs For 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.