What Can You Multiply To Get 24
Arias News
May 09, 2025 · 4 min read
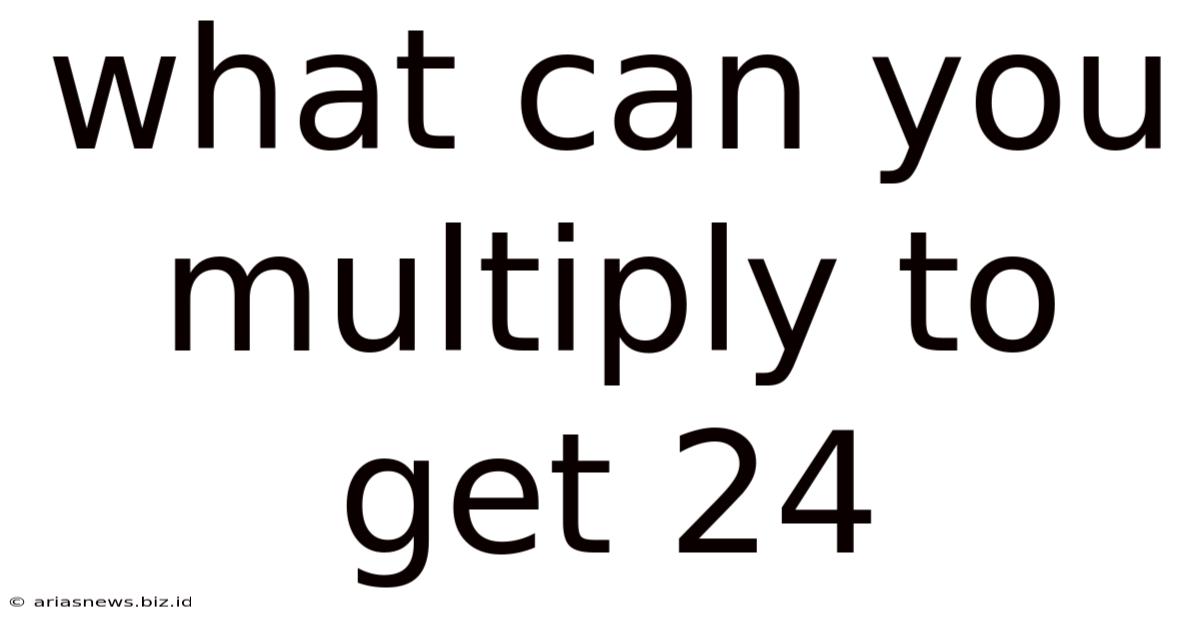
Table of Contents
What Can You Multiply to Get 24? A Deep Dive into Factors and Multiplication
Finding the numbers that multiply to 24 might seem like a simple math problem, but it opens the door to a fascinating exploration of factors, prime factorization, and even the beginnings of number theory. This article delves deep into the various ways you can obtain 24 through multiplication, exploring different approaches and showcasing the underlying mathematical concepts.
Understanding Factors and Multiples
Before we jump into the combinations that equal 24, let's establish a firm understanding of fundamental terms. A factor is a number that divides evenly into another number without leaving a remainder. Conversely, a multiple is the result of multiplying a number by an integer. In our case, 24 is the multiple, and we're looking for its factors.
Finding the Factors of 24
The most straightforward way to find the factors of 24 is to systematically consider each integer and check for divisibility. We can start with 1 and proceed upwards:
- 1 x 24 = 24
- 2 x 12 = 24
- 3 x 8 = 24
- 4 x 6 = 24
Notice that after 6, we start to repeat factors. This is because 6 x 4 is the same as 4 x 6. Therefore, the complete list of factors for 24 is 1, 2, 3, 4, 6, 8, 12, and 24.
Prime Factorization: The Building Blocks of Numbers
Prime factorization is a powerful technique that breaks down a number into its prime number components. A prime number is a whole number greater than 1 that has only two divisors: 1 and itself. The prime factorization of 24 is crucial for understanding its multiplicative properties.
To find the prime factorization of 24, we can use a factor tree:
24
/ \
2 12
/ \
2 6
/ \
2 3
This shows that 24 can be expressed as 2 x 2 x 2 x 3, or 2³ x 3. This prime factorization is unique to 24; no other set of prime numbers will multiply to give 24. This is a fundamental theorem in number theory.
Utilizing Prime Factorization to Find Combinations
Knowing the prime factorization (2³ x 3) allows us to systematically generate all possible combinations of factors that multiply to 24. We can combine different powers of 2 and the factor 3:
- 2 x 2 x 2 x 3 = 24 (The prime factorization itself)
- (2 x 2) x (2 x 3) = 4 x 6 = 24
- (2 x 2 x 2) x 3 = 8 x 3 = 24
- 2 x (2 x 2 x 3) = 2 x 12 = 24
- (2 x 3) x (2 x 2) = 6 x 4 = 24
- 2 x 12 = 24
- 3 x 8 = 24
- 4 x 6 = 24
- 1 x 24 = 24
These are all the different ways to obtain 24 using integer multiplication. We can see how the prime factorization acts as a foundation for generating these various combinations.
Exploring Negative Factors
The problem hasn't specified that we're limited to positive integers. We can also consider negative factors. Since a negative number multiplied by a negative number results in a positive number, we have even more possibilities:
- (-1) x (-24) = 24
- (-2) x (-12) = 24
- (-3) x (-8) = 24
- (-4) x (-6) = 24
And any combination of these with the positive factors.
The Significance of Factor Pairs
Each combination we've identified represents a factor pair. Factor pairs are two numbers that, when multiplied, give a specific product (in this case, 24). Understanding factor pairs helps in various mathematical applications, including simplifying fractions, solving equations, and understanding divisibility rules.
Beyond Integers: Extending to Rational Numbers
While the question implicitly suggests integer factors, we can broaden our perspective to include rational numbers (fractions and decimals). There are infinitely many combinations of rational numbers that multiply to 24. For example:
- (1/2) x 48 = 24
- (1/3) x 72 = 24
- (2/3) x 36 = 24
- 24 x 1 = 24
- 12 x 2 = 24
- 8 x 3 = 24
- 6 x 4 = 24
- 0.5 x 48 = 24
Applications of Finding Factors
The process of finding factors and multiples has far-reaching applications in various fields:
- Algebra: Factoring is crucial for solving quadratic equations and simplifying algebraic expressions.
- Geometry: Finding factors is essential when calculating areas and volumes.
- Number Theory: The study of factors and prime factorization forms the basis of number theory, a branch of mathematics dealing with the properties of integers.
- Computer Science: Algorithms related to prime factorization are used in cryptography and data security.
- Everyday Life: Understanding factors is helpful in everyday tasks, such as dividing items equally among people or calculating proportions for recipes.
Conclusion: More Than Just a Simple Math Problem
Determining the numbers that multiply to 24 is more than just a simple arithmetic exercise. It's a gateway to understanding fundamental mathematical concepts like factors, multiples, prime factorization, and the beauty of number theory. The various approaches and the infinite possibilities when extending beyond integers showcase the richness and complexity inherent within seemingly simple mathematical problems. By exploring these concepts, we gain a deeper appreciation for the interconnectedness of mathematical ideas and their applications in diverse fields. From basic arithmetic to advanced number theory, understanding the factors of 24 provides a solid foundation for further mathematical exploration. The seemingly simple question, "What can you multiply to get 24?", unveils a world of mathematical depth and intrigue.
Latest Posts
Latest Posts
-
How Old Would You Be If Born In 1989
May 09, 2025
-
How To Stick Balloons To A Wall
May 09, 2025
-
Where In The World Is It Winter Right Now
May 09, 2025
-
How Long Is A Pencil In Centimeters
May 09, 2025
-
Data In Order From The Smallest To Largest Or Vice Versa
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Can You Multiply To Get 24 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.