What Does No More Than Mean In Math
Arias News
Apr 02, 2025 · 5 min read
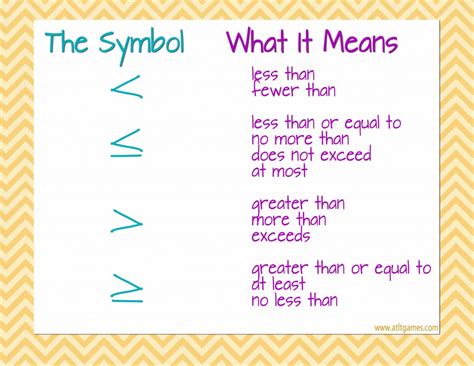
Table of Contents
What Does "No More Than" Mean in Math? A Comprehensive Guide
The phrase "no more than" in mathematics signifies a limit or constraint on a value. It's crucial to understand its precise meaning, as it directly impacts how you write and solve mathematical inequalities and word problems. This comprehensive guide will explore the nuances of "no more than" in various mathematical contexts, providing clear explanations and examples.
Understanding the Core Concept
In essence, "no more than" means a value is less than or equal to a specific number. It sets an upper bound, indicating that the value can reach that limit but cannot exceed it. This contrasts with phrases like "less than," which exclude the limiting value itself.
Let's illustrate this with simple examples:
-
"x is no more than 5" translates to
x ≤ 5. This means x can be any number from 5 down to negative infinity, including 5 itself. -
"The temperature is no more than 25 degrees Celsius" means the temperature is less than or equal to 25°C. It could be 25°C, 20°C, 10°C, or any value below 25°C.
-
"She has no more than 10 apples" implies she has 10 or fewer apples. The number of apples could be 10, 9, 8, all the way down to 0.
"No More Than" vs. Other Phrases
It's important to differentiate "no more than" from similar phrases often used in mathematical word problems:
-
"Less than": This signifies a value strictly smaller than a given number. For instance, "x is less than 5" is written as
x < 5. Note that x cannot be 5 in this case. -
"At most": This is a synonym for "no more than." Both phrases convey the same mathematical meaning. "The speed is at most 60 mph" is equivalent to "The speed is no more than 60 mph," both represented as
speed ≤ 60. -
"Maximum": While not directly interchangeable, "maximum" indicates the highest possible value. If the maximum value is 10, it's the same as saying "no more than 10."
-
"Greater than or equal to": This describes values exceeding or equaling a specific number, the opposite of "no more than." It's represented by the symbol
≥.
Applying "No More Than" in Inequalities
Inequalities are mathematical statements that compare values using symbols like <, >, ≤, and ≥. "No more than" directly translates to the "less than or equal to" symbol (≤).
Example 1: Simple Inequality
A student needs to score no more than 70 points to pass a test. Let 's' represent the student's score. The inequality representing this scenario is:
s ≤ 70
Example 2: Compound Inequality
A company's daily profit (P) should be no more than $5000 but also no less than $1000. This can be expressed using a compound inequality:
1000 ≤ P ≤ 5000
Example 3: Inequality with Variables
The sum of two numbers (x and y) is no more than 10. The inequality is:
x + y ≤ 10
Solving Word Problems with "No More Than"
Word problems often require translating real-world situations into mathematical inequalities. Here's a step-by-step approach:
-
Identify the key phrase: Locate the phrase "no more than" (or its synonyms) within the problem statement.
-
Define the variables: Assign variables to the unknown quantities.
-
Translate the phrase into an inequality: Use the
≤symbol to represent "no more than." -
Solve the inequality: Apply algebraic manipulation to isolate the variable and find the solution set.
-
Interpret the solution: Relate the mathematical solution back to the context of the word problem.
Example Word Problem:
A farmer can plant no more than 100 tomato plants in his field. Each tomato plant requires 1 square foot of space. If the farmer's field has an area of 120 square feet, how many tomato plants can he plant?
-
Key phrase: "no more than 100"
-
Variables: Let 'p' be the number of tomato plants.
-
Inequality:
p ≤ 100 -
Solution: Since the field has enough space for 120 plants but the farmer can plant only up to 100, the solution is still p ≤ 100.
-
Interpretation: The farmer can plant a maximum of 100 tomato plants, even though there's more space.
Advanced Applications
The concept of "no more than" extends to more complex scenarios involving:
-
Multiple inequalities: Systems of inequalities can represent situations with several constraints.
-
Optimization problems: "No more than" constraints often appear in linear programming problems where the goal is to maximize or minimize an objective function subject to certain limitations.
-
Calculus: In optimization problems using calculus, "no more than" translates to upper bounds on variables within constraints or domains.
Real-world examples of "No More Than"
Beyond mathematical textbooks, "no more than" appears frequently in everyday life:
-
Budgeting: "I can spend no more than $50 on groceries."
-
Time management: "I have no more than 30 minutes to complete this task."
-
Manufacturing: "The machine can produce no more than 100 units per hour."
-
Engineering: "The load-bearing capacity is no more than 5 tons."
Conclusion
Understanding the precise meaning of "no more than" is fundamental to correctly interpreting and solving mathematical problems. Its accurate translation into the ≤ symbol is crucial for building and solving inequalities, tackling word problems, and working with more advanced mathematical concepts. By grasping its meaning and applications across various mathematical domains, you'll improve your problem-solving skills significantly and handle real-world situations involving limitations and constraints effectively. Remember to practice regularly to solidify your understanding and apply this knowledge confidently in diverse contexts.
Latest Posts
Latest Posts
-
How Many Servings In A 2 Liter Of Soda
Apr 03, 2025
-
Which Of These Fair Deal Reforms Did Not Happen
Apr 03, 2025
-
Is Baking Powder The Same As Corn Starch
Apr 03, 2025
-
What Does It Feel Like To Finger Yourself
Apr 03, 2025
-
11 In Womens Is What In Mens
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Does No More Than Mean In Math . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
