What Is 1/2 Of 1/5 In Fraction
Arias News
May 12, 2025 · 4 min read
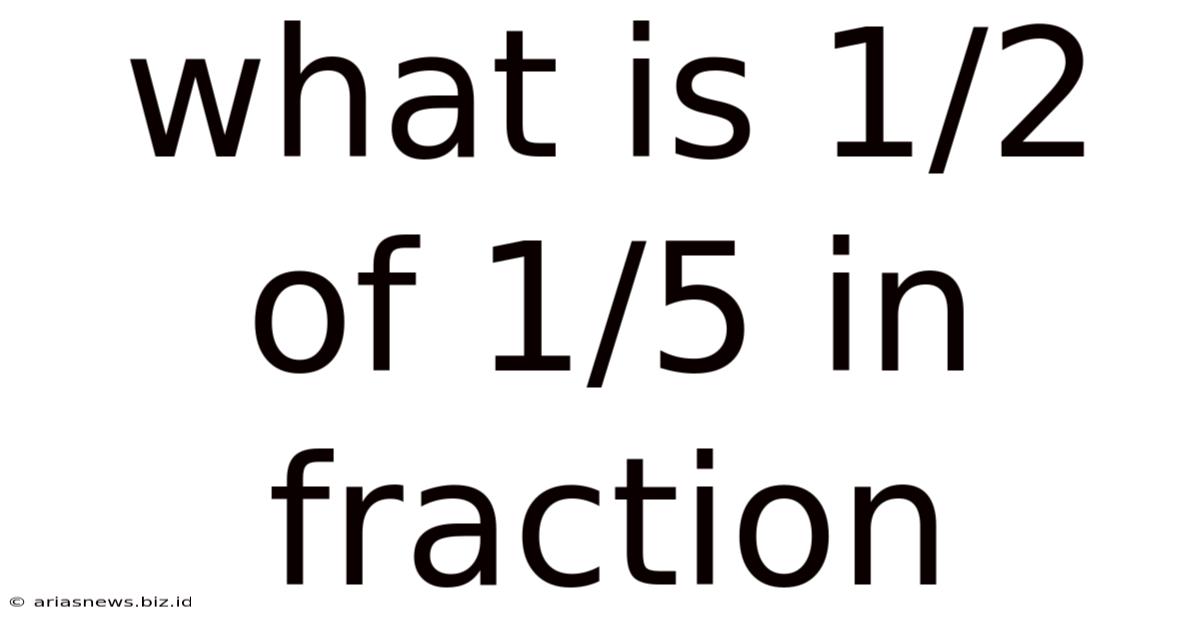
Table of Contents
What is 1/2 of 1/5 in Fraction? A Comprehensive Guide
Finding a fraction of another fraction might seem daunting at first, but it's a fundamental concept in mathematics with wide-ranging applications. This comprehensive guide will walk you through calculating 1/2 of 1/5, explaining the process step-by-step and exploring the underlying principles. We'll also delve into related concepts and offer practical examples to solidify your understanding.
Understanding Fractions
Before we tackle the problem, let's refresh our understanding of fractions. A fraction represents a part of a whole. It's written as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts we have, and the denominator indicates how many parts make up the whole.
For example, in the fraction 1/5, the numerator is 1, and the denominator is 5. This represents one out of five equal parts.
Calculating 1/2 of 1/5
To find 1/2 of 1/5, we need to multiply the two fractions. The process of multiplying fractions is relatively straightforward:
-
Multiply the numerators: Multiply the top numbers together. In this case, 1 (from 1/2) multiplied by 1 (from 1/5) equals 1.
-
Multiply the denominators: Multiply the bottom numbers together. In this case, 2 (from 1/2) multiplied by 5 (from 1/5) equals 10.
-
Combine the results: The result of multiplying the numerators becomes the new numerator, and the result of multiplying the denominators becomes the new denominator. Therefore, 1/2 of 1/5 is 1/10.
Visualizing the Calculation
Let's visualize this with a simple example. Imagine a pizza cut into 5 equal slices. 1/5 of the pizza represents one slice. Now, let's take half (1/2) of that single slice. We would effectively divide that slice into two equal parts, and take one of those parts. This represents 1/10 of the original pizza.
Different Ways to Express the Result
While 1/10 is the simplest form of the answer, it's crucial to understand that fractions can be expressed in different equivalent forms. For instance, 2/20, 3/30, and 4/40 are all equivalent to 1/10. These fractions represent the same proportion of the whole.
It's generally preferred to simplify fractions to their lowest terms, meaning the numerator and denominator have no common factors other than 1. In this case, 1/10 is already in its simplest form.
Applications of Fraction Multiplication
The concept of multiplying fractions has numerous applications in various fields, including:
-
Baking and Cooking: Scaling recipes up or down often involves multiplying fractions. For example, if a recipe calls for 1/2 cup of flour, and you want to make 1/3 of the recipe, you'd need to calculate 1/3 of 1/2 cup.
-
Construction and Engineering: Precise measurements are essential in construction and engineering projects. Fraction multiplication is crucial for accurate calculations of materials and dimensions.
-
Finance: Calculating interest, discounts, or proportions of investments frequently involves fraction multiplication.
-
Data Analysis: Understanding proportions and percentages in datasets often requires manipulating fractions.
Further Exploring Fractions: Addition and Subtraction
While we focused on multiplication, it's equally important to understand how to add and subtract fractions. To add or subtract fractions, they must have a common denominator. If they don't, you need to find the least common multiple (LCM) of the denominators and convert the fractions to equivalent fractions with that common denominator.
Example of Addition:
1/2 + 1/4 = 2/4 + 1/4 = 3/4
Example of Subtraction:
3/4 - 1/2 = 3/4 - 2/4 = 1/4
More Complex Fraction Calculations
While 1/2 of 1/5 is a relatively simple example, the same principles apply to more complex fraction calculations. For instance, calculating 3/4 of 2/5 would involve multiplying 3 by 2 (the numerators) to get 6, and multiplying 4 by 5 (the denominators) to get 20, resulting in 6/20. This fraction can then be simplified to 3/10.
Practicing with Different Fractions
To reinforce your understanding, try practicing with different fractions:
- What is 2/3 of 1/4?
- What is 3/5 of 2/7?
- What is 1/8 of 4/5?
Remember, the process remains consistent: multiply the numerators, multiply the denominators, and simplify the resulting fraction to its lowest terms.
Conclusion: Mastering Fraction Operations
Mastering fraction operations, including multiplication, addition, and subtraction, is a cornerstone of mathematical literacy. Understanding these concepts allows you to confidently tackle various problems across different fields. The seemingly simple calculation of 1/2 of 1/5 serves as a foundational example that unlocks a deeper understanding of fractions and their applications in the real world. Continuous practice and exploration of related concepts are key to building proficiency in this area. This comprehensive guide provides a solid base; continue exploring and refining your skills to become more confident with fractions.
Latest Posts
Latest Posts
-
How Much Is 3 4 Pint In Cups
May 12, 2025
-
How Much Does 17 Oz Of Water Weigh
May 12, 2025
-
What Happened To Dave Hester Of Storage Wars
May 12, 2025
-
0 76 As A Fraction In Simplest Form
May 12, 2025
-
What Color Does Grey And White Make
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 1/2 Of 1/5 In Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.