What Is 15 Percent As A Decimal
Arias News
May 11, 2025 · 5 min read
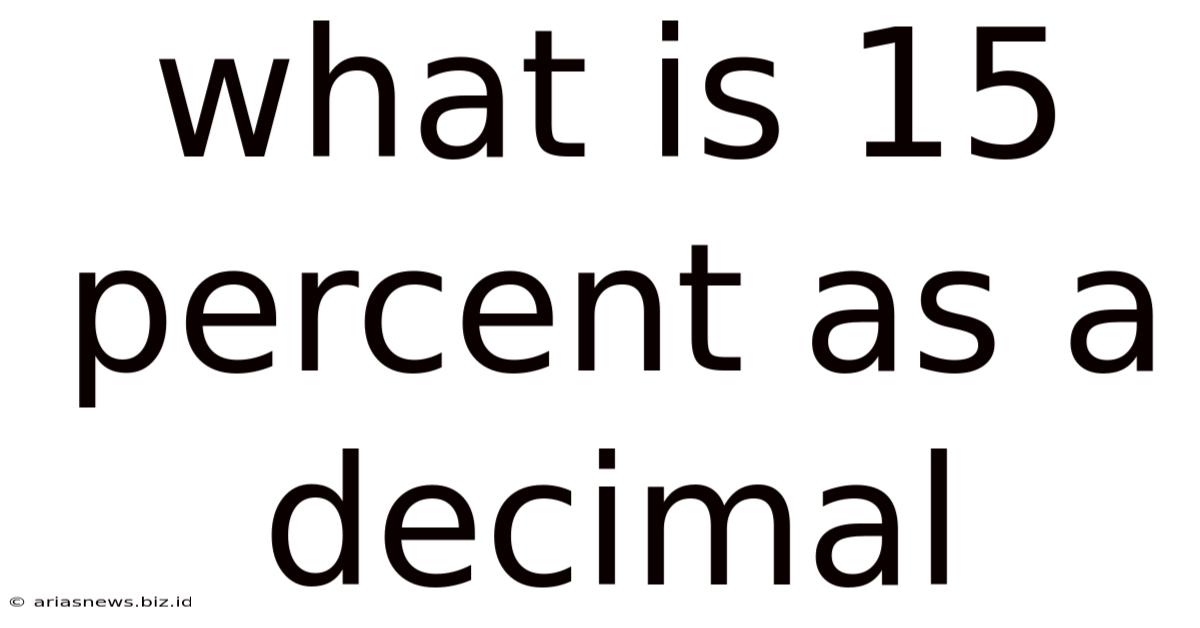
Table of Contents
What is 15 Percent as a Decimal? A Comprehensive Guide
Converting percentages to decimals is a fundamental skill in mathematics with wide-ranging applications in various fields, from finance and accounting to statistics and data analysis. Understanding this conversion is crucial for accurate calculations and data interpretation. This comprehensive guide will delve into the process of converting 15 percent to its decimal equivalent, exploring the underlying principles and providing practical examples to solidify your understanding. We'll also explore the broader context of percentage-to-decimal conversions and their significance in real-world scenarios.
Understanding Percentages and Decimals
Before diving into the specific conversion of 15 percent, let's establish a solid foundation. A percentage represents a fraction of 100. The term "percent" literally means "per hundred." For instance, 15 percent signifies 15 parts out of 100.
A decimal, on the other hand, is a way of expressing a number using a base-ten system, with a decimal point separating the whole number part from the fractional part. Decimals are commonly used to represent fractions and proportions, making them indispensable in numerical calculations.
Converting 15 Percent to a Decimal: The Simple Method
The conversion process is straightforward. To convert a percentage to a decimal, you simply divide the percentage by 100. This is because a percentage is inherently a fraction with a denominator of 100.
Therefore, to find the decimal equivalent of 15 percent, we perform the following calculation:
15% / 100 = 0.15
Therefore, 15 percent as a decimal is 0.15.
Alternative Method: Moving the Decimal Point
A quicker method involves moving the decimal point. Since percentages are always out of 100, you can simply move the decimal point two places to the left. This is equivalent to dividing by 100.
In the case of 15 percent, the implied decimal point is after the 15 (15.0). Moving the decimal point two places to the left gives us 0.15. This provides a quick and efficient way to convert percentages to decimals.
Practical Applications: Real-World Examples
The ability to convert percentages to decimals is invaluable in numerous real-world scenarios. Here are some examples:
1. Calculating Discounts:
Imagine a store offering a 15% discount on an item priced at $100. To calculate the discount amount, you would convert 15% to its decimal equivalent (0.15) and multiply it by the original price:
$100 * 0.15 = $15
The discount amount is $15.
2. Calculating Sales Tax:
Similarly, if the sales tax in your region is 15%, you would use the decimal equivalent (0.15) to calculate the tax on a purchase. For a $50 item, the sales tax would be:
$50 * 0.15 = $7.50
3. Financial Calculations:
In finance, interest rates are often expressed as percentages. Converting these percentages to decimals is crucial for calculating interest payments, loan repayments, and investment returns. For example, a 15% annual interest rate on a loan would be represented as 0.15 in calculations.
4. Data Analysis and Statistics:
Percentages are frequently used in data analysis and statistics to represent proportions and probabilities. Converting these percentages to decimals is essential for performing statistical calculations, such as calculating means, standard deviations, and correlations.
5. Scientific Calculations:
In scientific research and experiments, percentages are often used to represent experimental error, efficiency, or yield. Converting percentages to decimals allows for accurate calculations and data interpretation within scientific studies.
Beyond 15 Percent: Generalizing the Conversion
The methods outlined above are not limited to 15 percent. They can be applied to any percentage. Simply divide the percentage by 100 or move the decimal point two places to the left to obtain the decimal equivalent.
For example:
- 25% = 0.25
- 5% = 0.05
- 100% = 1.00
- 0.5% = 0.005
Common Mistakes and How to Avoid Them
While the conversion is relatively simple, some common mistakes can occur. Here are some points to remember:
- Forgetting to divide by 100: The most frequent error is forgetting to divide the percentage by 100 or failing to move the decimal point correctly. Always remember this crucial step.
- Incorrect decimal placement: Ensure you accurately place the decimal point in the decimal equivalent. A misplaced decimal point can significantly alter the result and lead to incorrect calculations.
- Confusing percentages and decimals: Keep in mind the distinction between percentages and decimals. A percentage is a fraction of 100, while a decimal uses the base-ten system. Understanding this difference is vital for accurate conversions.
Mastering Percentage-to-Decimal Conversions: Practice Exercises
Practice is key to mastering any mathematical skill. Here are a few exercises to reinforce your understanding of converting percentages to decimals:
- Convert 35% to a decimal.
- Convert 2.5% to a decimal.
- Convert 125% to a decimal.
- Convert 0.75% to a decimal.
- If a product costs $250 and has a 10% discount, calculate the discount amount using decimal conversion.
(Answers: 1. 0.35, 2. 0.025, 3. 1.25, 4. 0.0075, 5. $25)
Conclusion: The Importance of Percentage-to-Decimal Conversions
The ability to confidently convert percentages to decimals is an essential skill in a wide range of fields. This conversion allows for accurate calculations and data interpretation, making it crucial for anyone working with numerical data, whether in finance, statistics, science, or everyday life. By understanding the underlying principles and practicing the conversion methods, you can significantly improve your numerical skills and tackle various mathematical problems effectively. Remember to always double-check your calculations to ensure accuracy and avoid common errors. Mastering this simple conversion will empower you to navigate numerical tasks with increased confidence and precision.
Latest Posts
Latest Posts
-
Name Something That Is Shaped Like A Cylinder
May 12, 2025
-
Where Does Prime Rib Come From On The Cow
May 12, 2025
-
How Many Slices In A Standard Loaf Of Bread
May 12, 2025
-
How Long Does A Butterfly Stay In Its Cocoon
May 12, 2025
-
Value Of 14 Mg Of 24k Gold
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 15 Percent As A Decimal . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.