What Is 3.5 Percent Of 150 000
Arias News
May 09, 2025 · 4 min read
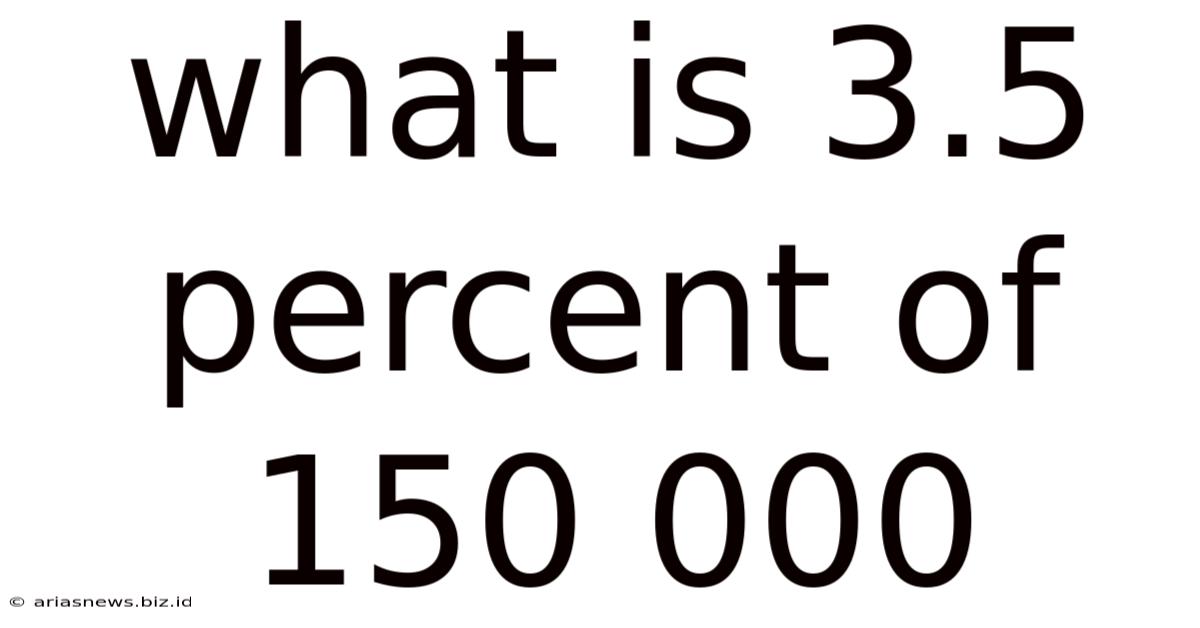
Table of Contents
What is 3.5 Percent of 150,000? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill applicable across numerous fields, from finance and budgeting to sales and statistics. Understanding how to calculate percentages efficiently and accurately is crucial for making informed decisions and solving everyday problems. This article will delve into the process of calculating 3.5 percent of 150,000, providing a detailed explanation and exploring various methods to arrive at the solution. We'll also touch upon the broader context of percentage calculations and their real-world applications.
Understanding Percentages
Before we dive into the calculation, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" is used to denote percentage. For instance, 50% means 50 out of 100, which is equivalent to ½ or 0.5. Percentages are a convenient way to represent proportions and make comparisons.
Method 1: Using Decimal Conversion
This is perhaps the most straightforward method. We convert the percentage to its decimal equivalent and then multiply it by the total value.
Step 1: Convert the Percentage to a Decimal
To convert 3.5% to a decimal, we divide it by 100:
3.5% / 100 = 0.035
Step 2: Multiply the Decimal by the Total Value
Now, we multiply the decimal equivalent (0.035) by the total value (150,000):
0.035 * 150,000 = 5250
Therefore, 3.5% of 150,000 is 5250.
Method 2: Using Fraction Conversion
This method involves converting the percentage to a fraction and then performing the multiplication.
Step 1: Convert the Percentage to a Fraction
3.5% can be written as the fraction 3.5/100. To simplify this fraction, we can multiply both the numerator and the denominator by 10 to remove the decimal:
(3.5 * 10) / (100 * 10) = 35/1000
This fraction can be further simplified by dividing both the numerator and the denominator by 5:
35/1000 = 7/200
Step 2: Multiply the Fraction by the Total Value
Now, we multiply the simplified fraction (7/200) by the total value (150,000):
(7/200) * 150,000 = (7 * 150,000) / 200 = 1,050,000 / 200 = 5250
Again, we arrive at the answer: 3.5% of 150,000 is 5250.
Method 3: Using the Proportion Method
This method uses the concept of proportions to solve the problem. We set up a proportion where 'x' represents the unknown value (3.5% of 150,000):
x / 150,000 = 3.5 / 100
To solve for 'x', we cross-multiply:
100x = 3.5 * 150,000
100x = 525,000
x = 525,000 / 100
x = 5250
Therefore, 3.5% of 150,000 is 5250.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is vital in many real-life scenarios:
-
Finance: Calculating interest on loans, savings accounts, and investments. Determining discounts on purchases, calculating taxes, and understanding profit margins are all dependent on percentage calculations. For example, if you invest $150,000 and receive a 3.5% annual return, you'd earn $5250.
-
Sales and Marketing: Analyzing sales data, tracking conversion rates, and determining the effectiveness of marketing campaigns often involve percentage calculations. For example, a company might track the percentage of website visitors who make a purchase.
-
Statistics and Data Analysis: Percentages are essential for summarizing and interpreting data. Researchers use percentages to represent proportions of a population or to compare different groups.
-
Everyday Life: Calculating tips at restaurants, determining sale prices, and understanding discounts all involve percentages.
Beyond the Basic Calculation: Understanding Percentage Increase and Decrease
While this article focuses on finding a percentage of a number, it's important to also understand how to calculate percentage increases and decreases. These calculations are frequently used to analyze changes over time.
Percentage Increase: If a value increases from an initial amount to a final amount, the percentage increase is calculated as:
[(Final Amount - Initial Amount) / Initial Amount] * 100%
Percentage Decrease: If a value decreases from an initial amount to a final amount, the percentage decrease is calculated as:
[(Initial Amount - Final Amount) / Initial Amount] * 100%
Advanced Percentage Calculations and Their Applications
More complex percentage calculations involve multiple percentages, compound interest, or percentage changes over multiple periods. For instance, calculating compound interest involves applying a percentage repeatedly over time, resulting in exponential growth. These calculations are frequently encountered in financial modeling and investment analysis.
Tips for Accurate Percentage Calculations
- Double-check your work: Always verify your calculations to avoid errors. Use a calculator to ensure accuracy.
- Understand the context: Before performing any calculation, clearly understand what the percentage represents and what the total value is.
- Use appropriate tools: Utilize calculators or spreadsheets to simplify complex calculations.
- Break down complex problems: Divide complex percentage problems into smaller, more manageable steps.
Conclusion
Calculating 3.5 percent of 150,000, which equals 5250, is a straightforward process achievable through various methods. Mastering percentage calculations is a valuable skill that extends far beyond basic arithmetic, finding widespread application in numerous aspects of personal and professional life. Understanding the different methods, along with the concepts of percentage increase and decrease, equips you with the tools to tackle a wide range of percentage-related problems with confidence and accuracy. By understanding the underlying principles and practicing regularly, you can build your proficiency and seamlessly integrate percentage calculations into your problem-solving toolkit.
Latest Posts
Latest Posts
-
What Are Quad Seats In A Car
May 10, 2025
-
Three Times A Day Is How Many Hours
May 10, 2025
-
Which Word Best Describes The Mood Of This Passage
May 10, 2025
-
How Do You Write The Sound Of A Whistle
May 10, 2025
-
Sample Leniency Letter To Judge For My Son
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is 3.5 Percent Of 150 000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.