What Is 3 To The 5 Power
Arias News
Mar 28, 2025 · 5 min read
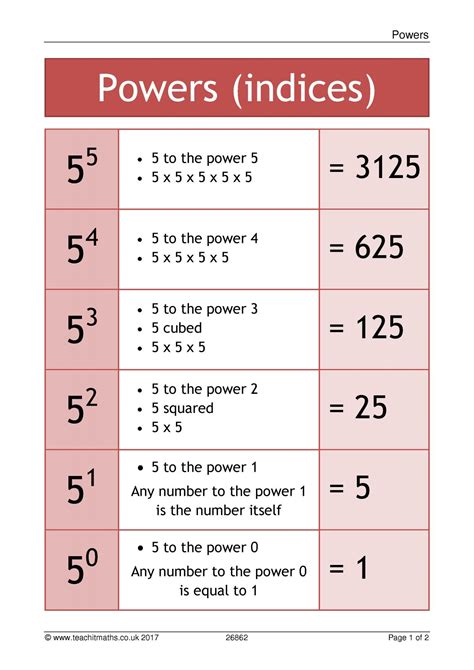
Table of Contents
What is 3 to the 5th Power? A Deep Dive into Exponents and Their Applications
Understanding exponents is fundamental to mathematics and has wide-ranging applications in various fields. This article delves into the meaning of "3 to the 5th power," exploring the concept of exponents, demonstrating its calculation, and showcasing its relevance in diverse contexts. We'll explore related mathematical concepts and provide practical examples to solidify your understanding.
Understanding Exponents: The Basics
Before diving into 3 to the 5th power, let's establish a solid foundation in exponential notation. An exponent, also known as a power or index, indicates how many times a number (the base) is multiplied by itself. It's written as a superscript to the right of the base.
For example, in the expression b<sup>n</sup>, 'b' represents the base, and 'n' represents the exponent. This expression means 'b' multiplied by itself 'n' times.
Examples:
- 2<sup>3</sup> means 2 * 2 * 2 = 8
- 5<sup>2</sup> means 5 * 5 = 25
- 10<sup>4</sup> means 10 * 10 * 10 * 10 = 10,000
Calculating 3 to the 5th Power (3<sup>5</sup>)
Now, let's address the central question: What is 3 to the 5th power, or 3<sup>5</sup>? This means multiplying the base, 3, by itself five times:
3<sup>5</sup> = 3 * 3 * 3 * 3 * 3 = 243
Therefore, 3 to the 5th power is 243.
Expanding the Concept: Properties of Exponents
Understanding the properties of exponents is crucial for manipulating and solving equations involving exponential expressions. Here are some key properties:
-
Product of Powers: When multiplying two numbers with the same base, add the exponents: b<sup>m</sup> * b<sup>n</sup> = b<sup>(m+n)</sup>
-
Quotient of Powers: When dividing two numbers with the same base, subtract the exponents: b<sup>m</sup> / b<sup>n</sup> = b<sup>(m-n)</sup>
-
Power of a Power: When raising a power to another power, multiply the exponents: (b<sup>m</sup>)<sup>n</sup> = b<sup>(m*n)</sup>
-
Power of a Product: When raising a product to a power, raise each factor to that power: (ab)<sup>n</sup> = a<sup>n</sup>b<sup>n</sup>
-
Power of a Quotient: When raising a quotient to a power, raise both the numerator and denominator to that power: (a/b)<sup>n</sup> = a<sup>n</sup>/b<sup>n</sup>
-
Zero Exponent: Any non-zero base raised to the power of zero equals 1: b<sup>0</sup> = 1
-
Negative Exponent: A negative exponent indicates the reciprocal of the base raised to the positive exponent: b<sup>-n</sup> = 1/b<sup>n</sup>
These properties are essential for simplifying complex exponential expressions and solving equations.
Real-World Applications of Exponents
Exponents aren't just abstract mathematical concepts; they have far-reaching applications in numerous fields:
1. Compound Interest:
Compound interest calculations heavily rely on exponents. The formula for compound interest is: A = P(1 + r/n)^(nt), where:
- A = the future value of the investment/loan, including interest
- P = the principal investment amount (the initial deposit or loan amount)
- r = the annual interest rate (decimal)
- n = the number of times that interest is compounded per year
- t = the number of years the money is invested or borrowed for
The exponent (nt) determines the effect of compounding over time. The more frequent the compounding (larger 'n'), the faster the growth.
2. Population Growth:
Exponential growth models are frequently used to describe population growth (both human and animal populations). If a population grows at a constant rate, its size after a certain period can be modeled using an exponential equation.
3. Radioactive Decay:
Radioactive decay follows an exponential decay model. The amount of a radioactive substance remaining after a specific time can be calculated using an exponential function. This is crucial in fields like nuclear physics, medicine (radioactive dating), and environmental science.
4. Computer Science:
Exponents are fundamental in computer science, especially in algorithms and data structures. The complexity of certain algorithms is expressed using Big O notation, which often involves exponential functions. For example, the time it takes to search an unsorted list is O(n), while searching a sorted list using binary search is O(log n).
5. Physics and Engineering:
Exponents appear frequently in physics and engineering equations. For instance, in physics, calculations involving energy, acceleration, and other physical phenomena often involve exponential expressions.
6. Finance:
Beyond compound interest, exponents are used in various financial models, including present value calculations, discounted cash flow analysis, and options pricing.
Beyond 3<sup>5</sup>: Exploring Larger Exponents
While we've focused on 3<sup>5</sup>, the principles extend to much larger exponents. Consider calculating 3<sup>10</sup>:
3<sup>10</sup> = (3<sup>5</sup>)<sup>2</sup> = 243<sup>2</sup> = 59049
This demonstrates how the properties of exponents simplify calculations with larger numbers.
Tackling More Complex Exponential Expressions
Let's explore some more complex scenarios involving exponents:
Example 1: Simplify (2<sup>3</sup> * 2<sup>4</sup>) / 2<sup>2</sup>
Using the properties of exponents:
- First, address the numerator: 2<sup>3</sup> * 2<sup>4</sup> = 2<sup>(3+4)</sup> = 2<sup>7</sup>
- Then, perform the division: 2<sup>7</sup> / 2<sup>2</sup> = 2<sup>(7-2)</sup> = 2<sup>5</sup> = 32
Example 2: Solve for x: 5<sup>x</sup> = 125
Since 125 = 5<sup>3</sup>, we can rewrite the equation as:
5<sup>x</sup> = 5<sup>3</sup>
Therefore, x = 3
Conclusion: The Significance of 3 to the 5th Power and Beyond
Understanding "3 to the 5th power," or 3<sup>5</sup> = 243, is just a starting point for comprehending the broader world of exponents. This seemingly simple calculation opens the door to a vast realm of mathematical applications, impacting diverse fields from finance and biology to computer science and physics. By mastering the fundamental concepts and properties of exponents, you equip yourself with a powerful tool for solving complex problems and interpreting data across numerous disciplines. The ability to manipulate and understand exponential expressions is invaluable for anyone seeking a deeper understanding of the quantitative world around us. Remember that continued practice and exploration are key to fully grasping the power and versatility of exponents.
Latest Posts
Latest Posts
-
Is 43 A Prime Or Composite Number
Mar 31, 2025
-
How Many Feet Are In A City Block
Mar 31, 2025
-
How Many Slices Of Pizza In A 9 Inch
Mar 31, 2025
-
How Many Miles Is 10 Minutes Driving
Mar 31, 2025
-
Average Bench Press For 13 Year Old
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is 3 To The 5 Power . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
