What Is 33 1/3 Percent As A Fraction
Arias News
May 12, 2025 · 5 min read
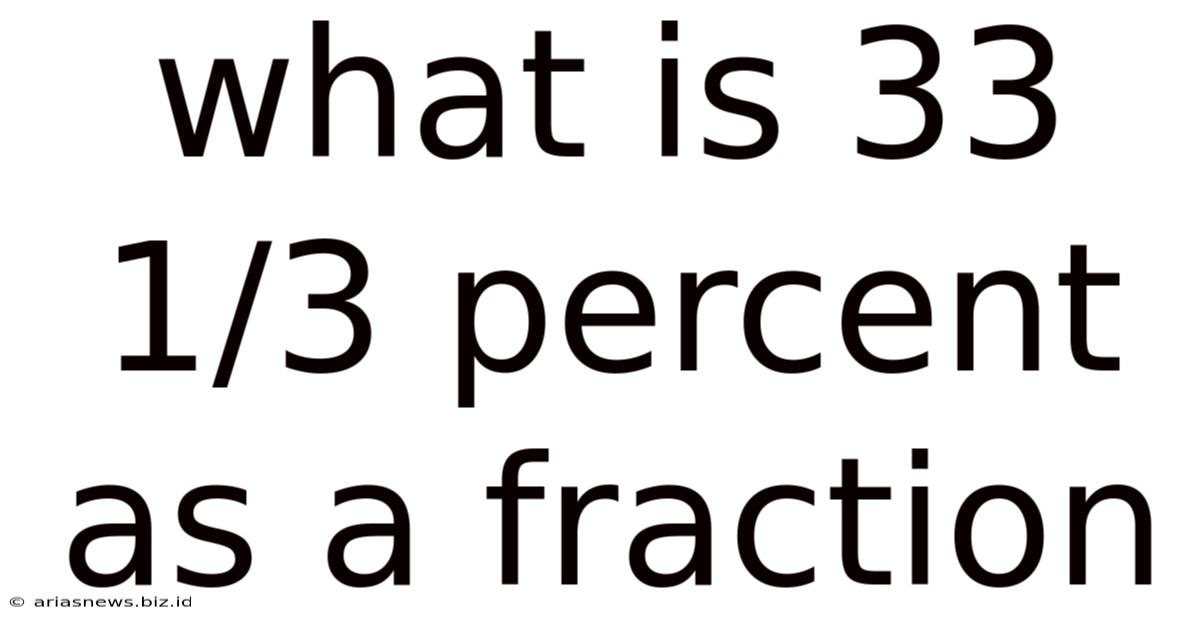
Table of Contents
What is 33 1/3 Percent as a Fraction? A Comprehensive Guide
Understanding percentages, fractions, and decimals is crucial for various aspects of life, from everyday budgeting to complex scientific calculations. This comprehensive guide will delve deep into converting the percentage 33 1/3% into its fractional equivalent, exploring the underlying mathematical concepts and offering practical applications. We'll also cover related conversions and problem-solving techniques to build a solid foundation in this area.
Understanding Percentages and Fractions
Before we tackle the conversion of 33 1/3%, let's establish a firm understanding of percentages and fractions.
Percentages: A percentage represents a fraction of 100. The word "percent" literally means "out of 100" (per centum in Latin). For instance, 50% means 50 out of 100, which can be written as the fraction 50/100.
Fractions: A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts you have, and the denominator indicates how many parts make up the whole. For example, in the fraction 1/4, 1 is the numerator and 4 is the denominator. This means you have one part out of four equal parts.
The Relationship: Percentages and fractions are intrinsically linked. Any percentage can be expressed as a fraction, and vice versa. The key is understanding that the percentage is always a fraction with a denominator of 100.
Converting 33 1/3% to a Fraction: Step-by-Step
Converting 33 1/3% to a fraction involves several steps:
1. Convert the Mixed Number to an Improper Fraction:
The percentage 33 1/3% contains a mixed number (33 1/3). First, we need to convert this mixed number into an improper fraction. To do this, multiply the whole number (33) by the denominator (3), add the numerator (1), and place the result over the original denominator (3):
(33 * 3) + 1 = 100
So, 33 1/3 becomes 100/3.
2. Express the Percentage as a Fraction:
Now that we have the improper fraction, we can express the percentage as a fraction:
33 1/3% = 100/3%
3. Convert the Percentage to a Decimal:
Remember, the percent sign (%) means "divided by 100". Therefore, we divide the improper fraction by 100:
100/3% = (100/3) / 100
4. Simplify the Fraction:
To simplify the complex fraction, we multiply the numerator by the reciprocal of the denominator:
(100/3) * (1/100) = 100/300
Now, we can simplify this fraction by dividing both the numerator and the denominator by their greatest common divisor (GCD), which is 100:
100/300 = 1/3
Therefore, 33 1/3% as a fraction is 1/3.
Understanding the Result: 1/3
The fraction 1/3 represents one part out of three equal parts. This is a recurring decimal (0.333...), meaning the decimal representation goes on infinitely. This is why 33 1/3% is often used instead of 0.333... because it offers a precise and concise representation of the fraction.
Practical Applications of 33 1/3% and its Fractional Equivalent
The fraction 1/3, representing 33 1/3%, has numerous applications in various fields:
-
Cooking and Baking: Recipes often call for fractional measurements. Understanding 1/3 is essential for accurately measuring ingredients.
-
Construction and Engineering: Calculations involving proportions and ratios frequently utilize fractions, including 1/3.
-
Finance: Calculating discounts, interest rates, and portions of investments often involve fractional calculations.
-
Data Analysis: Data analysis frequently uses fractions and percentages to represent proportions within datasets.
-
Everyday Life: Dividing items equally among three people, calculating one-third of a distance, or understanding sales discounts all involve the practical application of 1/3.
Converting Other Percentages to Fractions: A General Approach
The method used for converting 33 1/3% can be generalized to convert any percentage to a fraction:
-
Express the percentage as a fraction with a denominator of 100: For example, 75% becomes 75/100.
-
Simplify the fraction: Divide both the numerator and the denominator by their greatest common divisor (GCD). In the case of 75/100, the GCD is 25, so the simplified fraction is 3/4.
-
Handle mixed numbers: If the percentage contains a mixed number, convert it to an improper fraction before proceeding.
Converting Fractions to Percentages: The Reverse Process
The reverse process, converting a fraction to a percentage, is equally important:
-
Divide the numerator by the denominator: For example, to convert 1/4 to a percentage, divide 1 by 4, resulting in 0.25.
-
Multiply the result by 100: Multiply 0.25 by 100 to get 25%.
This simple process allows you to easily convert between fractions and percentages, enhancing your mathematical flexibility.
Advanced Applications: Working with Complex Percentages and Fractions
Beyond simple conversions, understanding percentages and fractions becomes crucial when dealing with more complex mathematical operations. For example:
-
Calculating percentage increases and decreases: Understanding how to calculate percentage changes is essential in various financial and statistical contexts.
-
Solving problems involving proportions: Proportions are frequently expressed using fractions, and the ability to work with these proportions is vital for problem-solving.
-
Working with compound interest: Calculating compound interest involves repeated calculations involving percentages and fractions.
Conclusion: Mastering Percentages and Fractions
Mastering the conversion between percentages and fractions is a fundamental skill with broad applications. Understanding the process of converting 33 1/3% to 1/3, as well as the general methods for converting other percentages and fractions, provides a powerful tool for navigating various mathematical challenges in both academic and real-world scenarios. This understanding lays a solid foundation for tackling more complex mathematical problems involving percentages, fractions, and their applications in various fields. By practicing these conversions and applying them to practical problems, you'll enhance your mathematical proficiency and confidence in tackling numerical challenges. Remember, consistent practice and a clear understanding of the underlying principles are key to mastering these essential mathematical concepts.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is 33 1/3 Percent As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.