What Is 6 1/2 As A Fraction
Arias News
May 11, 2025 · 5 min read
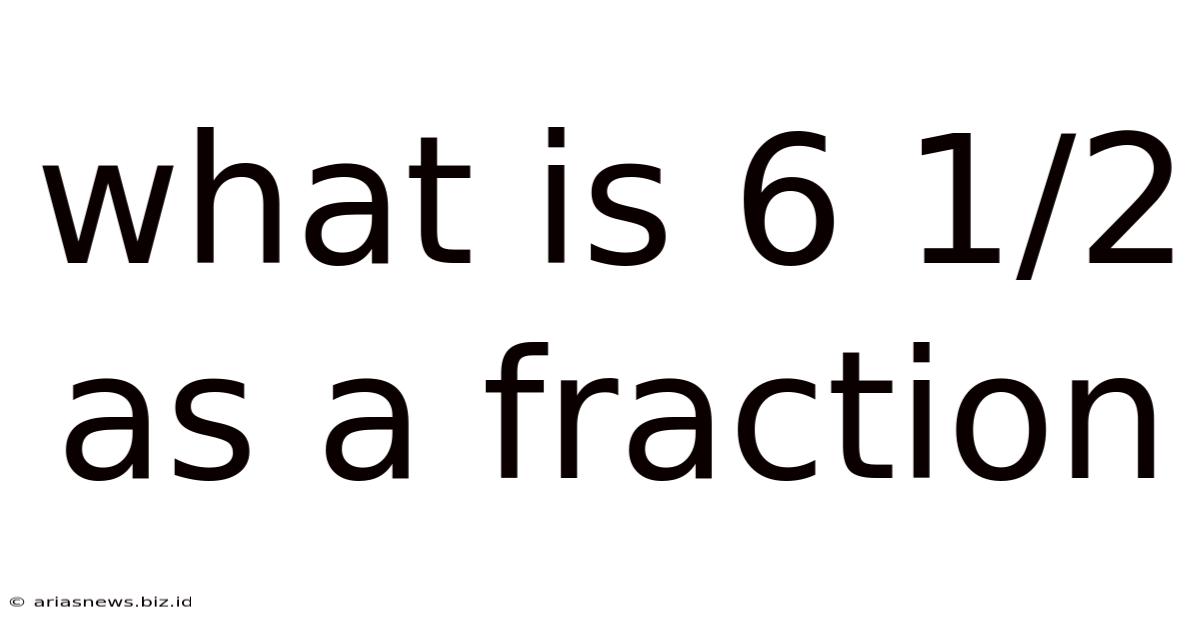
Table of Contents
What is 6 1/2 as a Fraction? A Comprehensive Guide
Understanding fractions is a fundamental skill in mathematics, impacting various aspects of life from cooking to construction. This article delves into the seemingly simple question, "What is 6 1/2 as a fraction?" but expands on the concept to provide a comprehensive understanding of mixed numbers, improper fractions, and their conversions. We'll explore the process, provide examples, and offer tips for tackling similar problems with confidence.
Understanding Mixed Numbers and Improper Fractions
Before diving into the conversion, let's clarify the terminology. The number "6 1/2" is a mixed number. A mixed number combines a whole number (6 in this case) and a proper fraction (1/2). A proper fraction has a numerator (top number) smaller than the denominator (bottom number). Conversely, an improper fraction has a numerator equal to or greater than the denominator. Converting 6 1/2 into a fraction means transforming it into an improper fraction.
Why Convert to an Improper Fraction?
Converting mixed numbers to improper fractions is crucial for various mathematical operations, particularly addition, subtraction, multiplication, and division of fractions. Working with improper fractions simplifies these calculations, providing a more efficient and accurate approach.
Converting 6 1/2 to an Improper Fraction: A Step-by-Step Guide
The conversion process involves two simple steps:
Step 1: Multiply the whole number by the denominator.
In our case, the whole number is 6, and the denominator of the fraction 1/2 is 2. Therefore, we multiply 6 x 2 = 12.
Step 2: Add the numerator to the result from Step 1.
The numerator of our fraction is 1. Adding this to the result from Step 1 (12), we get 12 + 1 = 13.
Step 3: Keep the denominator the same.
The denominator remains unchanged throughout the conversion process. Therefore, the denominator remains 2.
Therefore, 6 1/2 as an improper fraction is 13/2.
Visualizing the Conversion
Imagine you have six whole pizzas and half a pizza. To represent this as a single fraction, consider cutting each of the six whole pizzas into two equal halves. This gives you 6 x 2 = 12 halves. Adding the extra half, you have a total of 12 + 1 = 13 halves. Since each piece represents one-half of a pizza, the total number of halves (13) becomes the numerator, and the size of each piece (half) remains the denominator (2). This illustrates why 6 1/2 is equivalent to 13/2.
Practical Applications: Real-world Examples
The conversion of mixed numbers to improper fractions isn't just a theoretical exercise. It has many practical applications:
-
Cooking: A recipe calls for 2 1/4 cups of flour. To accurately measure this using a 1/4 cup measuring cup, you need to know that 2 1/4 cups is equivalent to 9/4 cups (2 x 4 + 1 = 9, denominator remains 4).
-
Construction: Calculating the length of a material requires converting mixed numbers to improper fractions for precise measurements. If a carpenter needs 3 3/8 feet of wood, converting this to an improper fraction (27/8 feet) ensures accurate cutting.
-
Sewing: Patterns often use fractional measurements. Converting mixed numbers to improper fractions simplifies calculations when working with fabrics.
-
Finance: Calculating interest or dividing assets often involves fractions. Converting mixed numbers to improper fractions simplifies calculations, leading to greater accuracy.
Beyond 6 1/2: Mastering Fraction Conversions
The method used to convert 6 1/2 to an improper fraction applies to all mixed numbers. Let's explore a few more examples to solidify your understanding:
Example 1: Convert 3 2/5 to an improper fraction.
- Multiply the whole number by the denominator: 3 x 5 = 15
- Add the numerator: 15 + 2 = 17
- Keep the denominator: The denominator remains 5.
Therefore, 3 2/5 as an improper fraction is 17/5.
Example 2: Convert 10 3/4 to an improper fraction.
- Multiply the whole number by the denominator: 10 x 4 = 40
- Add the numerator: 40 + 3 = 43
- Keep the denominator: The denominator remains 4.
Therefore, 10 3/4 as an improper fraction is 43/4.
Example 3: Convert 1 1/8 to an improper fraction
- Multiply the whole number by the denominator: 1 x 8 = 8
- Add the numerator: 8 + 1 = 9
- Keep the denominator: The denominator remains 8.
Therefore, 1 1/8 as an improper fraction is 9/8
Converting Improper Fractions back to Mixed Numbers
It's equally important to be able to convert improper fractions back into mixed numbers. This involves dividing the numerator by the denominator.
Example: Convert 17/5 to a mixed number.
- Divide the numerator (17) by the denominator (5): 17 ÷ 5 = 3 with a remainder of 2.
- The quotient (3) becomes the whole number.
- The remainder (2) becomes the numerator of the fraction.
- The denominator remains the same (5).
Therefore, 17/5 as a mixed number is 3 2/5.
Troubleshooting Common Mistakes
-
Forgetting to add the numerator: A common mistake is to only multiply the whole number by the denominator and forget to add the numerator. Remember, the whole number represents a certain number of whole units, and the numerator represents the additional part of a unit.
-
Changing the denominator: The denominator should always remain the same during the conversion process. It represents the size of the fractional parts.
Conclusion: Mastering Fractions for Everyday Life
Understanding how to convert mixed numbers like 6 1/2 into improper fractions (13/2) is a fundamental skill applicable across numerous areas of life. By mastering this conversion and understanding the underlying concepts of mixed numbers, proper fractions, and improper fractions, you'll build a strong foundation in mathematics, making calculations simpler and more accurate in both academic and real-world scenarios. Practice these conversions regularly, and you’ll quickly gain confidence and proficiency in working with fractions. Remember to visualize the concept using real-world examples to reinforce your understanding.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is 6 1/2 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.