What Is 8 Out Of 30 As A Percentage
Arias News
May 07, 2025 · 4 min read
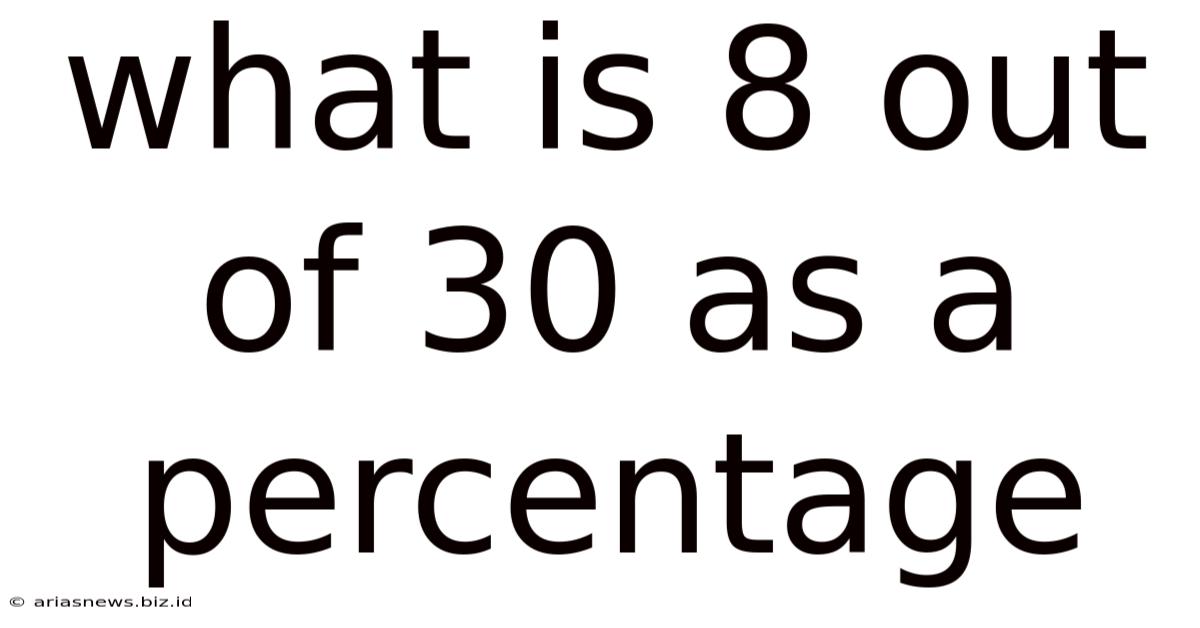
Table of Contents
What is 8 out of 30 as a Percentage? A Comprehensive Guide
Calculating percentages is a fundamental skill with wide-ranging applications, from everyday budgeting and shopping to more complex scenarios in finance, science, and data analysis. Understanding how to convert fractions into percentages is crucial for interpreting data, making informed decisions, and communicating information effectively. This comprehensive guide delves into the process of calculating "8 out of 30 as a percentage," exploring the underlying principles, providing multiple methods of calculation, and demonstrating practical applications.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred." Therefore, 25% means 25 out of 100, which can also be expressed as the fraction 25/100 or the decimal 0.25. Percentages are used extensively because they provide a standardized and easily comparable way to represent proportions and ratios.
Method 1: Using the Formula
The most straightforward method to calculate "8 out of 30 as a percentage" involves using the basic percentage formula:
(Part / Whole) x 100% = Percentage
In this case:
- Part: 8 (the number we're interested in)
- Whole: 30 (the total number)
Substituting these values into the formula:
(8 / 30) x 100% = Percentage
This simplifies to:
0.266666... x 100% ≈ 26.67%
Therefore, 8 out of 30 is approximately 26.67%. The recurring decimal is usually rounded to two decimal places for practical purposes.
Understanding the Calculation
The formula works by first determining the proportion of the part to the whole (8/30). This fraction represents the ratio of the specific value (8) to the total value (30). Multiplying this ratio by 100% converts the fraction into a percentage, expressing it as a number out of 100.
Method 2: Using Decimal Conversion
An alternative method involves converting the fraction to a decimal first, and then multiplying by 100%.
- Convert the fraction to a decimal: Divide the part (8) by the whole (30): 8 ÷ 30 = 0.266666...
- Multiply by 100%: 0.266666... x 100% ≈ 26.67%
This method yields the same result as the previous method, confirming the accuracy of the calculation.
Method 3: Simplifying the Fraction (Optional)
Before performing the calculation, you can simplify the fraction 8/30 by finding the greatest common divisor (GCD) of 8 and 30. The GCD of 8 and 30 is 2. Dividing both the numerator and the denominator by 2 simplifies the fraction to 4/15.
Now, apply the percentage formula:
(4 / 15) x 100% ≈ 26.67%
Simplifying the fraction beforehand can make the calculation slightly easier, especially with larger numbers, but it's not strictly necessary.
Practical Applications: Real-World Examples
The ability to calculate percentages like "8 out of 30" has numerous practical applications in various fields:
1. Academic Performance:
Imagine a student scoring 8 out of 30 on a quiz. Converting this score to a percentage (26.67%) provides a clearer understanding of their performance relative to the total possible marks. This allows for easier comparison with other assessments and helps identify areas for improvement.
2. Business and Finance:
Businesses use percentages extensively. For instance, if a company sold 8 out of 30 units of a product, calculating the percentage (26.67%) helps assess sales performance, predict future demand, and adjust inventory accordingly. Similarly, calculating profit margins, discounts, and interest rates all involve percentage calculations.
3. Data Analysis and Statistics:
In statistical analysis, percentages are fundamental for representing data proportions. For example, if a survey of 30 people showed that 8 preferred a specific brand, the percentage (26.67%) provides a concise summary of the preferences within the sample population. This is crucial for making generalizations about larger populations.
4. Everyday Life:
Even everyday tasks involve percentages. Calculating sales tax, discounts at stores, or the percentage of a bill you need to pay are all practical applications of percentage calculations.
Advanced Concepts and Considerations
While the basic methods are straightforward, understanding some advanced concepts can enhance your ability to work with percentages:
-
Rounding: As seen in the calculations, percentages often involve decimal values. Rounding to an appropriate number of decimal places is crucial for clarity and practical application. The level of precision required depends on the context.
-
Percentage Increase/Decrease: This involves calculating the percentage change between two values. For example, if the number increased from 8 to 12, the percentage increase would be calculated as: ((12-8)/8) * 100% = 50%.
-
Percentage Points: It's important to distinguish between percentage points and percentage change. A change from 20% to 28% is an increase of 8 percentage points, but an increase of 40% (8/20 * 100%).
-
Using Calculators and Spreadsheets: For more complex calculations or large datasets, using calculators or spreadsheet software (like Excel or Google Sheets) is highly recommended. These tools can efficiently handle percentage calculations, freeing you to focus on interpreting the results.
Conclusion
Calculating "8 out of 30 as a percentage" is a simple yet essential skill with broad applications. Understanding the different methods, their underlying principles, and practical applications empowers you to confidently interpret data, make informed decisions, and communicate information effectively in various contexts. Whether in academics, business, data analysis, or everyday life, mastering percentage calculations is a valuable asset. By practicing these methods and exploring the advanced concepts discussed, you can significantly enhance your numerical literacy and problem-solving capabilities.
Latest Posts
Latest Posts
-
Greatest Common Factor Of 13 And 26
May 11, 2025
-
1 Lb Cheese Equals How Many Cups
May 11, 2025
-
How Many Cups Of Sliced Jalapenos In A Pound
May 11, 2025
-
How Many Ml Is A Glass Of Water
May 11, 2025
-
What Is A Childrens Size 4 In Womens
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is 8 Out Of 30 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.