What Is Half Of 2 Plus 2
Arias News
May 09, 2025 · 5 min read
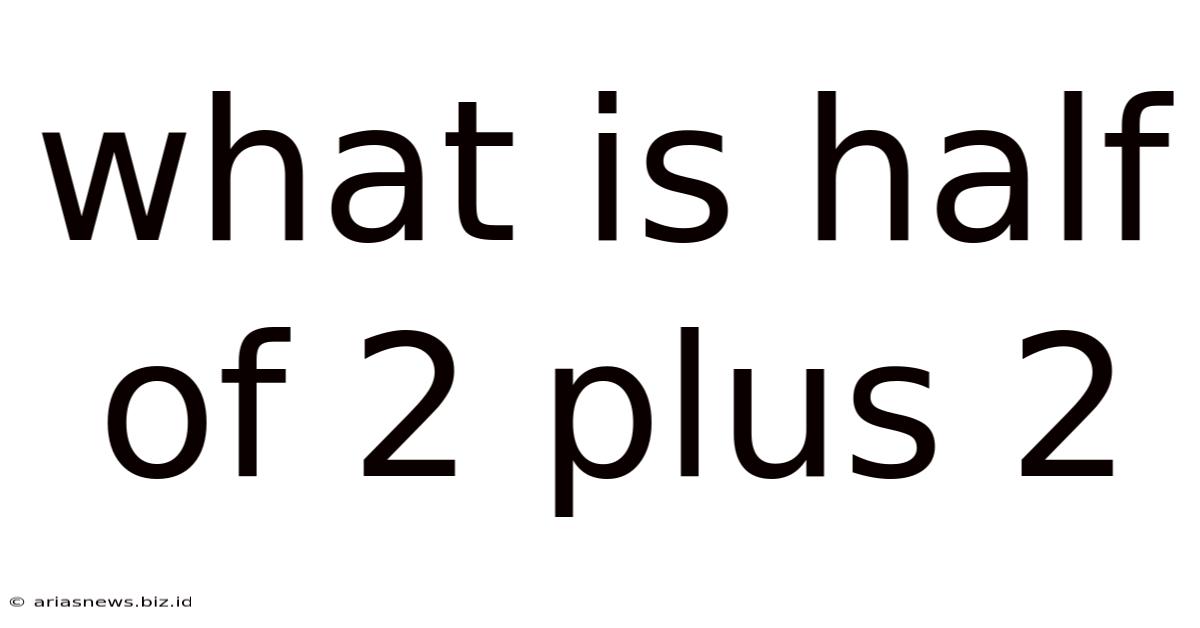
Table of Contents
What is Half of 2 Plus 2? A Deep Dive into Simple Arithmetic and its Implications
The seemingly simple question, "What is half of 2 plus 2?", while easily solvable at a glance, opens a door to exploring fundamental mathematical concepts, their practical applications, and even their philosophical implications. This seemingly elementary problem touches upon order of operations, the building blocks of algebra, and the importance of precision in mathematics. Let's delve into this seemingly simple equation and uncover its hidden depths.
Understanding the Order of Operations (PEMDAS/BODMAS)
Before we even begin to calculate "half of 2 plus 2," we must acknowledge the crucial role of the order of operations. This set of rules, commonly remembered by the acronyms PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) or BODMAS (Brackets, Orders, Division and Multiplication, Addition and Subtraction), dictates the sequence in which we perform calculations within an equation. Without adhering to these rules, we risk arriving at an incorrect answer.
In our case, the equation "half of 2 plus 2" can be written as:
(1/2 * 2) + 2
Following PEMDAS/BODMAS, we prioritize multiplication (or division) before addition.
1. Multiplication: Half of 2 is (1/2 * 2) = 1
2. Addition: Now we add the result to 2: 1 + 2 = 3
Therefore, the solution to "half of 2 plus 2" is definitively 3.
Beyond the Calculation: Exploring Mathematical Concepts
While the calculation itself is straightforward, let's explore the broader mathematical concepts underpinning this simple equation:
1. Fractions and Decimals:
The term "half" introduces the concept of fractions, representing parts of a whole. Understanding fractions is essential for numerous applications, from baking recipes to advanced engineering calculations. Fractions can also be expressed as decimals (0.5 in this case), expanding the range of numerical representations and simplifying some calculations. The ability to convert between fractions and decimals is a fundamental skill in mathematics.
2. Multiplication and Division:
Our equation employs both multiplication and division. These are inverse operations, meaning they "undo" each other. Mastering multiplication and division is crucial for tackling more complex equations and understanding ratios and proportions. These concepts are used extensively in fields such as finance, physics, and computer science.
3. Addition and Subtraction:
Addition and subtraction form the foundation of arithmetic. They are the building blocks upon which more complex mathematical operations are constructed. Understanding these operations is critical for everyday tasks, from balancing a checkbook to calculating the total cost of groceries.
4. Order of Operations and Ambiguity:
The order of operations highlights the importance of clear and unambiguous mathematical notation. Without a standardized order, different interpretations of the same equation could lead to vastly different results. This principle extends to all fields involving quantitative analysis, emphasizing the need for precision and consistency in communication.
Real-World Applications: From Everyday Life to Advanced Science
The principles demonstrated by this simple equation extend far beyond the realm of theoretical mathematics. Let's consider some practical applications:
1. Everyday Calculations:
From splitting a restaurant bill evenly to calculating discounts, the concepts of fractions, addition, and division are used constantly in everyday life. The ability to perform these calculations accurately and quickly is a valuable life skill.
2. Baking and Cooking:
Recipes often rely on fractions and ratios. Understanding how to halve or double a recipe requires a solid grasp of these fundamental mathematical concepts. Precise calculations are essential to ensure the desired outcome.
3. Finance and Economics:
Financial calculations rely heavily on addition, subtraction, multiplication, and division. From calculating interest rates to budgeting expenses, mathematical proficiency is crucial for responsible financial management. Understanding percentages, a direct application of fractions, is also critical in this field.
4. Engineering and Physics:
Engineering and physics heavily rely on mathematical principles. Precise calculations are essential for designing structures, predicting the behavior of systems, and ensuring safety. From calculating forces to designing circuits, a thorough understanding of mathematics is indispensable.
5. Computer Science and Programming:
Programming relies heavily on mathematical logic and precision. Computer algorithms and data structures often involve complex calculations, and understanding the order of operations is crucial for writing efficient and error-free code.
The Philosophical Implications: Precision and Clarity in Thought
Beyond its practical applications, this simple arithmetic problem underscores the importance of precision and clarity in thinking. Mathematics demands unambiguous language and precise execution. The potential for error when neglecting the order of operations highlights the need for careful consideration and systematic approaches in all aspects of life, not just mathematics.
The seemingly simple equation becomes a microcosm of the larger world: a reminder that even the smallest details can have significant consequences. This idea extends to problem-solving in general, emphasizing the importance of a structured and methodical approach to achieve accurate and reliable results.
Extending the Problem: Variations and Further Exploration
Let's consider variations of the original problem to further explore these concepts:
-
What is 2 plus half of 2? This changes the order of operations, leading to a different result (2 + (1/2 * 2) = 3). This demonstrates the critical impact of the order in which operations are performed.
-
What is half of (2 plus 2)? This introduces parentheses, altering the grouping and the order of operations, resulting in a different solution ((1/2) * (2 + 2) = 2). This highlights the role of parentheses in controlling the sequence of calculations.
-
What is half of (2 multiplied by 2) plus 2? This problem introduces multiplication before addition, requiring careful application of PEMDAS/BODMAS, leading to the solution: ((1/2) * (2 * 2)) + 2 = 4.
These variations showcase how seemingly small changes in the equation can lead to significantly different outcomes. This emphasizes the importance of carefully interpreting mathematical notation and correctly applying the rules of arithmetic.
Conclusion: A Simple Problem with Profound Implications
In conclusion, while the solution to "What is half of 2 plus 2?" is a simple 3, the underlying concepts and their real-world applications are far-reaching. This seemingly elementary problem serves as a powerful reminder of the fundamental importance of mathematics in our lives, emphasizing the need for precision, clarity, and a structured approach to problem-solving. From everyday calculations to advanced scientific endeavors, the principles illustrated in this simple equation are indispensable tools for navigating the complexities of the world around us. The ability to understand and apply these principles empowers us to tackle more complex challenges with confidence and accuracy.
Latest Posts
Latest Posts
-
What Year Would I Be If I Was 16
May 10, 2025
-
Why Do Justices Use Precedents In Majority Opinions And Dissents
May 10, 2025
-
How Many Sweet Potatoes Makes 3 Cups
May 10, 2025
-
How Do You Say 29 In Spanish
May 10, 2025
-
What Does Tutti A Tavola A Mangiare Mean
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is Half Of 2 Plus 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.