What Is One Half Of Two Thirds
Arias News
May 09, 2025 · 5 min read
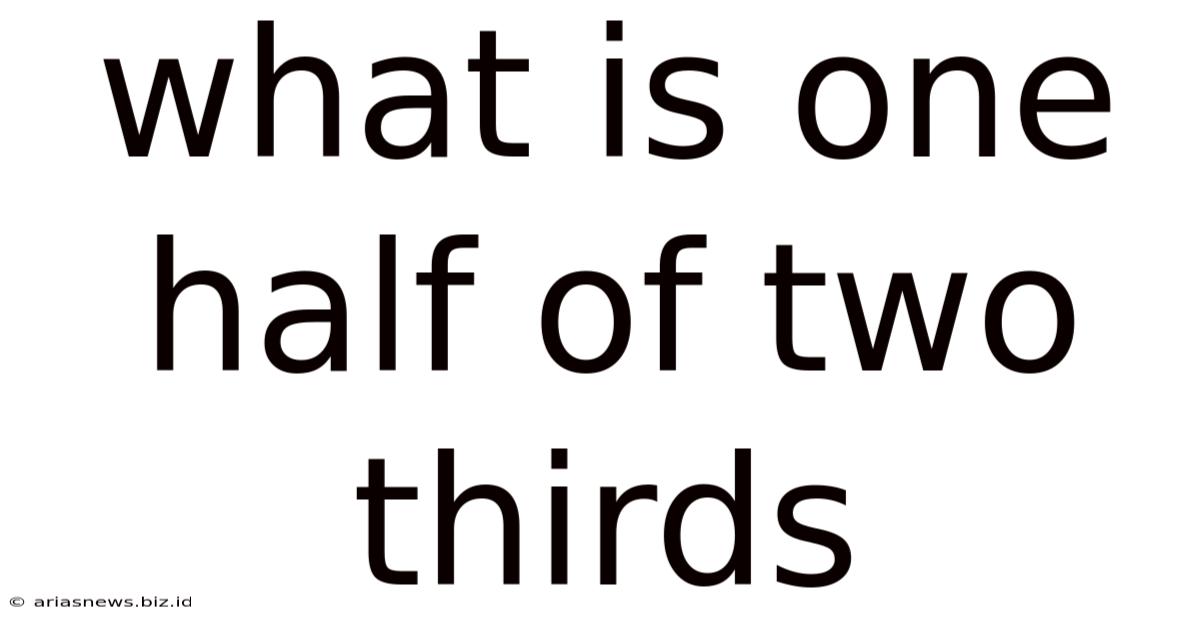
Table of Contents
What is One Half of Two Thirds? A Deep Dive into Fractions
This seemingly simple question, "What is one half of two thirds?", opens the door to a fascinating exploration of fractions, their manipulation, and their real-world applications. While the answer itself is straightforward, understanding the underlying principles solidifies a crucial foundation in mathematics and problem-solving. This article will not only provide the answer but will delve into the "why" and "how," exploring various methods to solve the problem and highlighting the importance of fractional arithmetic.
Understanding the Problem: Deconstructing "One Half of Two Thirds"
The phrase "one half of two thirds" translates directly into a mathematical expression: (1/2) * (2/3). This signifies multiplication of two fractions. Let's break down each component:
-
One half (1/2): This represents one part out of two equal parts of a whole. Think of cutting a pizza into two equal slices; one half is one of those slices.
-
Two thirds (2/3): This represents two parts out of three equal parts of a whole. Imagine a chocolate bar divided into three equal pieces; two thirds is two of those pieces.
The problem asks us to find what portion of the whole is represented by taking half of the two-thirds portion.
Method 1: Direct Multiplication of Fractions
The most straightforward approach is to multiply the two fractions directly:
(1/2) * (2/3) = (1 * 2) / (2 * 3) = 2/6
This simplifies to 1/3. Therefore, one half of two thirds is one third.
Simplifying Fractions: A Crucial Step
The result 2/6 is not in its simplest form. Simplifying fractions involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it. In this case, the GCD of 2 and 6 is 2. Dividing both the numerator and denominator by 2 yields the simplified fraction 1/3. Always simplify fractions to their lowest terms for clarity and ease of understanding.
Method 2: Visual Representation with Diagrams
Visualizing the problem using diagrams can enhance understanding, particularly for those who find abstract mathematical concepts challenging.
Imagine a rectangle representing the whole. Divide this rectangle into three equal vertical sections. Two thirds (2/3) of the rectangle would represent two of these sections.
Now, divide each of the three vertical sections into two equal horizontal sections. This divides the entire rectangle into six equal smaller rectangles. One half (1/2) of the two thirds (2/3) section corresponds to two of these smaller rectangles (one half of each of the two vertical thirds).
This visual representation clearly demonstrates that one half of two thirds is equal to two out of six equal parts, which simplifies to one third (1/3).
Method 3: Using the Concept of "Of" as Multiplication
The word "of" in mathematics often indicates multiplication. So, "one half of two thirds" is equivalent to (1/2) multiplied by (2/3). This reinforces the understanding of the mathematical translation of the problem statement.
Real-World Applications: Where Fractions Matter
Fractional arithmetic isn't confined to textbooks; it's essential for numerous real-world applications:
-
Cooking and Baking: Recipes often require fractional amounts of ingredients. Understanding how to work with fractions ensures accurate measurements and successful culinary endeavors. For example, if a recipe calls for two-thirds of a cup of flour, and you only want to make half the recipe, you need to calculate one half of two thirds of a cup.
-
Construction and Engineering: Precise measurements are crucial in construction and engineering. Fractions are used to represent dimensions, quantities of materials, and proportions in designs and blueprints. Calculations involving fractions guarantee accuracy and structural integrity.
-
Finance and Budgeting: Managing personal finances or business accounts frequently involves fractional amounts. Calculating percentages, interest rates, and portions of budgets all require a solid grasp of fractions. For instance, understanding what one half of two thirds of your monthly budget represents can greatly improve financial planning.
-
Data Analysis and Statistics: In various fields, data is often represented and analyzed using fractions and percentages. Statistical calculations frequently involve fractional components to represent probabilities, proportions, and trends in data sets.
Beyond the Basics: Expanding Fractional Understanding
Understanding "one half of two thirds" is a stepping stone to more complex fractional operations:
-
Addition and Subtraction of Fractions: This involves finding a common denominator before adding or subtracting the numerators. Mastering this skill is essential for solving more intricate fractional problems.
-
Division of Fractions: Dividing by a fraction is equivalent to multiplying by its reciprocal. This is a crucial concept for solving more advanced problems and for understanding the inverse relationship between multiplication and division.
-
Mixed Numbers and Improper Fractions: Learning to convert between mixed numbers (a whole number and a fraction) and improper fractions (where the numerator is larger than the denominator) is vital for flexibility in problem-solving.
-
Fractions and Decimals: Understanding the relationship between fractions and decimals, and the ability to convert between them, expands problem-solving capabilities significantly.
Mastering Fractions: A Continuous Journey
The question, "What is one half of two thirds?", while seemingly simple, provides a gateway to a deeper understanding of fractions and their multifaceted role in mathematics and real-world applications. By mastering basic fractional operations and exploring different approaches to solving problems, you lay a solid foundation for tackling more complex mathematical challenges. Continuous practice and exploration of diverse problem-solving methods are key to achieving proficiency in working with fractions. The ability to confidently manipulate fractions translates to increased competence across a wide array of subjects and real-world scenarios. The seemingly simple answer of one-third unlocks a world of mathematical possibilities.
Latest Posts
Latest Posts
-
60 Tens Is The Same As How Many Hundreds
May 11, 2025
-
The Shortest Wavelength Within The Visible Spectrum Is
May 11, 2025
-
How Many Ounces In A Brick Of Cream Cheese
May 11, 2025
-
How Much Is 3 Quarts In Liters
May 11, 2025
-
How Long Did It Take Aang To Master The Elements
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Is One Half Of Two Thirds . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.