What Is The Decimal For 2 1/2
Arias News
Mar 30, 2025 · 5 min read
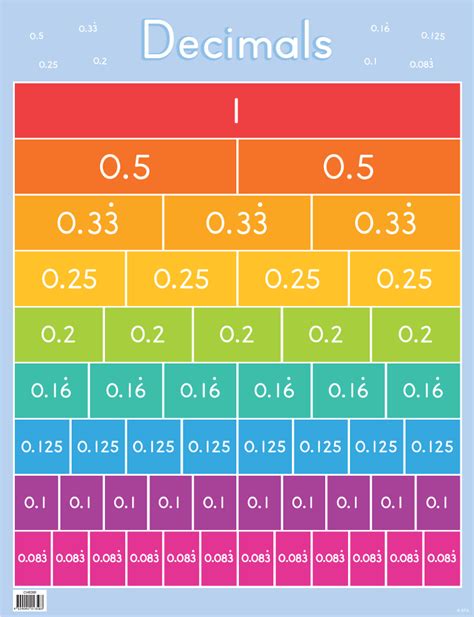
Table of Contents
What is the Decimal for 2 1/2? A Deep Dive into Fraction-to-Decimal Conversion
The seemingly simple question, "What is the decimal for 2 1/2?" opens a door to a broader understanding of fraction-to-decimal conversion, a fundamental concept in mathematics with wide-ranging applications. While the answer itself is straightforward, exploring the why behind the conversion process provides valuable insights for students and anyone looking to strengthen their numerical skills. This article delves deep into this seemingly simple problem, exploring various methods of conversion, practical applications, and addressing common misconceptions.
Understanding Fractions and Decimals
Before tackling the conversion, let's establish a clear understanding of fractions and decimals.
Fractions represent parts of a whole. They consist of a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts we have, while the denominator indicates how many equal parts the whole is divided into. In the fraction 2 1/2, '2' represents the whole numbers and '1/2' represents a half or one part out of two equal parts.
Decimals are another way of representing parts of a whole. They use a base-ten system, with digits to the right of the decimal point representing tenths, hundredths, thousandths, and so on. For instance, 0.5 represents five-tenths, and 0.25 represents twenty-five hundredths.
Converting 2 1/2 to a Decimal: The Simple Method
The most straightforward way to convert 2 1/2 to a decimal involves understanding that the fraction 1/2 represents half, or 0.5. Therefore, 2 1/2 is simply 2 + 0.5 = 2.5.
Alternative Conversion Methods: Expanding Our Understanding
While the above method is efficient for this specific fraction, understanding alternative methods provides a more robust foundation for converting more complex fractions.
Method 1: Dividing the Numerator by the Denominator
This method is a cornerstone of fraction-to-decimal conversion. It involves dividing the numerator of the fraction by its denominator. However, for 2 1/2, we first need to convert the mixed number (a whole number and a fraction) into an improper fraction.
-
Step 1: Convert to an Improper Fraction: To convert 2 1/2 to an improper fraction, we multiply the whole number (2) by the denominator (2) and add the numerator (1). This gives us (2 * 2) + 1 = 5. The denominator remains the same, resulting in the improper fraction 5/2.
-
Step 2: Perform the Division: Now, we divide the numerator (5) by the denominator (2): 5 ÷ 2 = 2.5. This confirms our initial answer.
This method is universally applicable to all fractions, making it a powerful tool in your mathematical arsenal.
Method 3: Using Decimal Equivalents of Common Fractions
Memorizing the decimal equivalents of common fractions like 1/2 (0.5), 1/4 (0.25), 1/5 (0.2), and 1/10 (0.1) can significantly speed up conversions. This method is particularly useful for mental calculations and estimations. Since we know 1/2 = 0.5, we can directly apply this knowledge to convert 2 1/2 to 2.5.
Practical Applications of Fraction-to-Decimal Conversion
The ability to convert fractions to decimals is crucial in numerous contexts:
-
Financial Calculations: Calculating interest rates, discounts, and profit margins often involves working with fractions and decimals. Converting between these formats ensures accuracy and clarity.
-
Measurement and Engineering: Many measurements, especially in engineering and construction, are expressed in both fractional and decimal forms. Converting between the two is essential for compatibility and precision.
-
Data Analysis and Statistics: Data analysis often involves working with numerical data, which may be presented as fractions or decimals. Converting between formats allows for consistent analysis and interpretation.
-
Scientific Calculations: Scientific computations frequently involve fractions and decimals. The ability to convert between these formats ensures accuracy and facilitates the use of scientific calculators and software.
-
Everyday Life: From cooking (following recipes with fractional measurements) to calculating tips, fraction-to-decimal conversions are commonly used in daily life.
Addressing Common Misconceptions
Several common misconceptions surround fraction-to-decimal conversions:
-
Assuming all fractions have finite decimal representations: While many common fractions have terminating decimals (like 1/2 = 0.5), some fractions result in repeating decimals (like 1/3 = 0.333...). Understanding this distinction is critical for accurate conversions.
-
Incorrectly applying the division process: A frequent error is incorrectly dividing the numerator by the denominator. Always ensure you're performing the division correctly to avoid inaccuracies.
-
Forgetting to convert mixed numbers to improper fractions: When dealing with mixed numbers, it’s essential to convert them to improper fractions before applying the division method. Failing to do so leads to incorrect results.
Beyond 2 1/2: Mastering Fraction-to-Decimal Conversions
While this article focused on the specific example of 2 1/2, the principles and methods discussed are universally applicable. By mastering these techniques, you can confidently convert any fraction to its decimal equivalent, opening up a world of mathematical possibilities.
Remember to practice regularly. Start with simple fractions and gradually progress to more complex ones. The more you practice, the more proficient you'll become.
Here are some practice exercises:
- Convert 3 1/4 to a decimal.
- Convert 1 2/5 to a decimal.
- Convert 7/8 to a decimal.
- Convert 5/6 to a decimal (note this will result in a repeating decimal).
- Convert 2 3/10 to a decimal.
By tackling these exercises and applying the methods described in this article, you'll strengthen your understanding of fraction-to-decimal conversions and build a solid foundation for future mathematical endeavors. This fundamental skill is invaluable across numerous academic, professional, and everyday scenarios. Mastering it opens doors to a more comprehensive understanding of numbers and their diverse representations.
Latest Posts
Latest Posts
-
A Desk Is How Many Meters High
Apr 01, 2025
-
How Many Ounces Of Peanut Butter Are In A Cup
Apr 01, 2025
-
How Many Times Does 7 Go Into 9
Apr 01, 2025
-
What Does Your Dream Mean When You Get Shot
Apr 01, 2025
-
1 Cup Of Sour Cream Is How Many Ounces
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Decimal For 2 1/2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
