What Is The Gcf Of 56 And 64
Arias News
May 12, 2025 · 5 min read
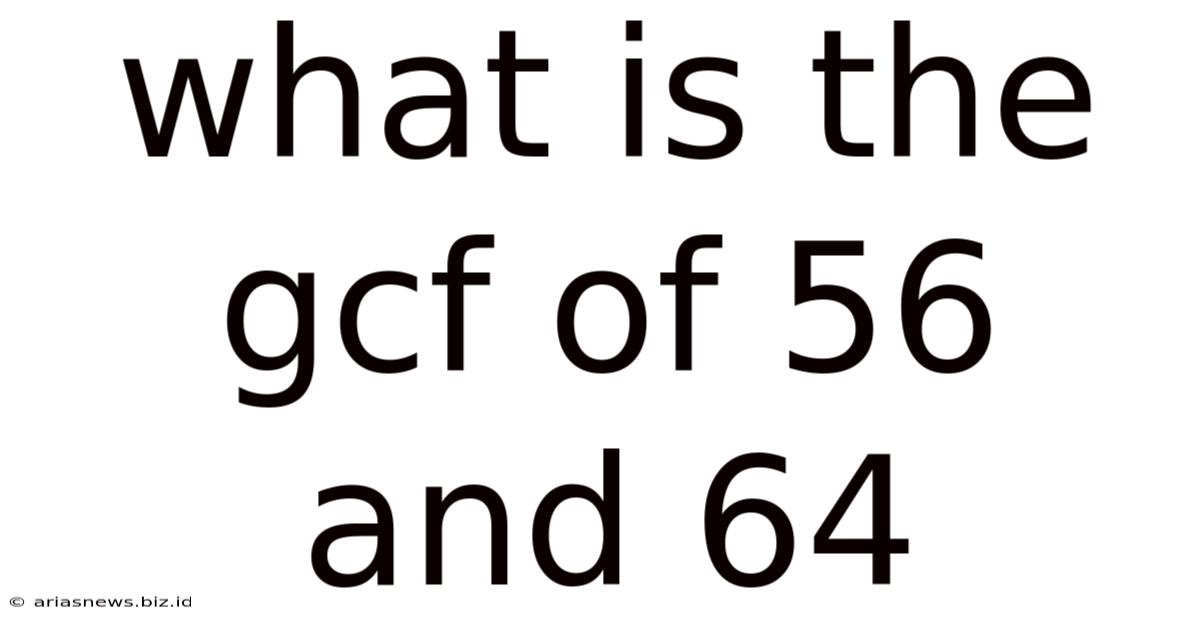
Table of Contents
What is the GCF of 56 and 64? A Deep Dive into Finding the Greatest Common Factor
Finding the greatest common factor (GCF) of two numbers might seem like a simple arithmetic task, but understanding the underlying principles and different methods involved can be surprisingly insightful. This in-depth exploration delves into the question: What is the GCF of 56 and 64? We'll not only find the answer but also examine various approaches to determine the GCF, exploring their practical applications and solidifying your understanding of fundamental number theory concepts.
Understanding the Greatest Common Factor (GCF)
Before we tackle the specific problem of finding the GCF of 56 and 64, let's clarify what the GCF actually represents. The greatest common factor (also known as the greatest common divisor or GCD) of two or more integers is the largest positive integer that divides each of the integers without leaving a remainder. In simpler terms, it's the biggest number that goes evenly into both numbers.
Think of it like finding the largest common building block of two numbers. Just as we can build structures using various sized bricks, numbers can be built using their factors. The GCF identifies the largest factor they share.
Method 1: Prime Factorization
The prime factorization method is a robust and reliable approach for determining the GCF, especially when dealing with larger numbers. It involves breaking down each number into its prime factors—numbers divisible only by 1 and themselves.
Let's apply this method to find the GCF of 56 and 64:
1. Prime Factorization of 56:
56 can be broken down as follows:
- 56 = 2 x 28
- 56 = 2 x 2 x 14
- 56 = 2 x 2 x 2 x 7
- Therefore, the prime factorization of 56 is 2³ x 7
2. Prime Factorization of 64:
64 can be broken down as follows:
- 64 = 2 x 32
- 64 = 2 x 2 x 16
- 64 = 2 x 2 x 2 x 8
- 64 = 2 x 2 x 2 x 2 x 4
- 64 = 2 x 2 x 2 x 2 x 2 x 2
- Therefore, the prime factorization of 64 is 2⁶
3. Identifying Common Factors:
Now, compare the prime factorizations of 56 (2³ x 7) and 64 (2⁶). The only common prime factor is 2.
4. Determining the GCF:
To find the GCF, we take the lowest power of the common prime factor(s). In this case, the lowest power of 2 is 2³.
Therefore, the GCF of 56 and 64 is 2³ = 8.
Method 2: Listing Factors
This method is suitable for smaller numbers and provides a visual understanding of the factors. It involves listing all the factors of each number and identifying the largest common factor.
1. Factors of 56: 1, 2, 4, 7, 8, 14, 28, 56
2. Factors of 64: 1, 2, 4, 8, 16, 32, 64
3. Common Factors: By comparing the lists, the common factors are 1, 2, 4, and 8.
4. Greatest Common Factor: The largest of these common factors is 8.
Method 3: Euclidean Algorithm
The Euclidean algorithm is an efficient method, particularly useful for larger numbers. It's based on the principle that the GCF of two numbers does not change if the larger number is replaced by its difference with the smaller number. This process is repeated until the two numbers are equal, which represents the GCF.
Let's apply the Euclidean algorithm to 56 and 64:
- 64 - 56 = 8
- Now we find the GCF of 56 and 8.
- 56 - 8 x 7 = 0
Since the remainder is 0, the GCF is the last non-zero remainder, which is 8. This method elegantly avoids the need for prime factorization.
Applications of Finding the GCF
The seemingly simple task of finding the greatest common factor has numerous practical applications across various fields:
-
Simplification of Fractions: The GCF is crucial for simplifying fractions to their lowest terms. Dividing both the numerator and denominator by their GCF results in an equivalent fraction in its simplest form. For example, simplifying 56/64 would involve dividing both by their GCF (8), resulting in 7/8.
-
Algebraic Simplification: In algebra, the GCF is used to factor expressions, simplifying them and making them easier to work with.
-
Geometry and Measurement: The GCF plays a role in solving problems related to areas, perimeters, and volumes involving integer measurements.
-
Cryptography: Number theory concepts, including the GCF, are fundamental to many cryptographic algorithms used to secure data.
-
Computer Science: GCF calculations are used in various algorithms and data structures, including those used in computer graphics and data compression.
Beyond the Basics: Extending the Concept
The GCF concept extends beyond two numbers. We can find the GCF of three or more numbers by applying the same methods. For example, to find the GCF of 56, 64, and 72, we could use prime factorization to find the common prime factors and their lowest powers. Alternatively, we could use the Euclidean algorithm iteratively.
Furthermore, the concept of the least common multiple (LCM) is closely related to the GCF. The LCM is the smallest positive integer that is divisible by all the numbers in a set. There's a useful relationship between the GCF and LCM of two numbers (a and b):
LCM(a, b) x GCF(a, b) = a x b
Understanding this relationship allows for efficient calculation of either the LCM or GCF if the other is known.
Conclusion: Mastering the GCF
Finding the GCF of 56 and 64, as we've demonstrated, yields the answer 8. However, the true value of this exercise lies in understanding the different methods available and their broader applicability. Whether you use prime factorization, listing factors, or the Euclidean algorithm, the choice depends on the numbers involved and the context of the problem. Mastering these methods equips you with valuable tools not only for simple arithmetic tasks but also for tackling more complex problems in mathematics and its diverse applications. The seemingly simple concept of the GCF opens up a fascinating world of number theory and its practical implications.
Latest Posts
Latest Posts
-
Explain Two Advances In Education During The Middle Ages
May 12, 2025
-
How Many Lines Of Reflectional Symmetry Does The Trapezoid Have
May 12, 2025
-
Compare And Contrast Mechanical Weathering And Chemical Weathering
May 12, 2025
-
What Percentage Of Golfers Shoot Under 100
May 12, 2025
-
At The Moment Of Impact In A Vehicle Collision
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is The Gcf Of 56 And 64 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.