What Is The Gcf Of 9 And 15
Arias News
May 10, 2025 · 5 min read
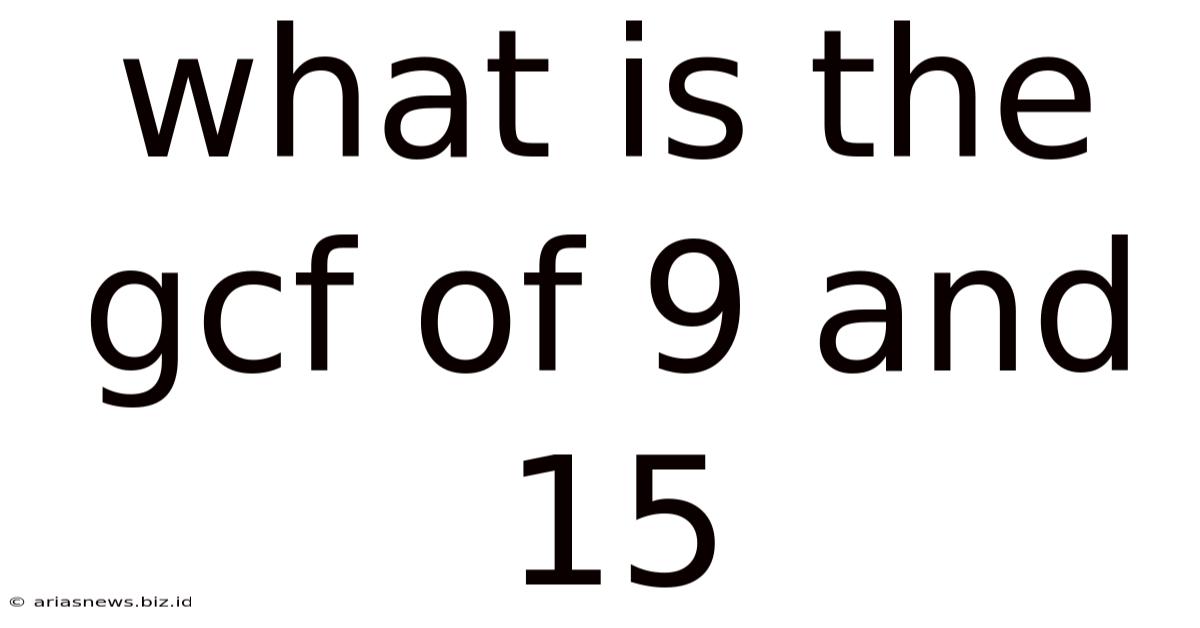
Table of Contents
What is the GCF of 9 and 15? A Deep Dive into Greatest Common Factors
Finding the greatest common factor (GCF) of two numbers might seem like a simple arithmetic problem, but understanding the underlying concepts and different methods for solving it opens doors to more advanced mathematical concepts. This comprehensive guide will not only answer the question, "What is the GCF of 9 and 15?" but will also explore the various techniques for finding the GCF, their applications, and their significance in mathematics.
Understanding Greatest Common Factors (GCF)
The greatest common factor (GCF), also known as the greatest common divisor (GCD), is the largest positive integer that divides each of the integers without leaving a remainder. In simpler terms, it's the biggest number that goes evenly into both numbers. Understanding GCFs is fundamental to simplifying fractions, solving algebraic equations, and working with various mathematical concepts.
Why are GCFs Important?
GCFs are more than just a mathematical curiosity. They have practical applications in various fields, including:
-
Simplifying Fractions: Finding the GCF of the numerator and denominator allows you to reduce a fraction to its simplest form. For example, simplifying 15/9 requires finding the GCF (which is 3), resulting in the simplified fraction 5/3.
-
Algebraic Simplification: GCFs are crucial in simplifying algebraic expressions. Factoring out the GCF from an expression allows you to simplify it and make it easier to manipulate.
-
Geometry and Measurement: GCFs are used in solving geometric problems involving area and perimeter calculations, particularly when dealing with dimensions that need to be divided into equal parts.
-
Number Theory: GCFs form the foundation of many concepts in number theory, such as modular arithmetic and the Euclidean algorithm.
Methods for Finding the GCF of 9 and 15
Let's explore several methods to find the GCF of 9 and 15.
1. Listing Factors Method
This method involves listing all the factors of each number and identifying the largest common factor.
- Factors of 9: 1, 3, 9
- Factors of 15: 1, 3, 5, 15
By comparing the two lists, we see that the common factors are 1 and 3. The largest common factor is 3. Therefore, the GCF of 9 and 15 is 3.
2. Prime Factorization Method
This method involves finding the prime factorization of each number and then multiplying the common prime factors raised to the lowest power.
- Prime factorization of 9: 3 x 3 = 3²
- Prime factorization of 15: 3 x 5
The only common prime factor is 3, and it appears to the lowest power of 1 in both factorizations. Therefore, the GCF is 3.
3. Euclidean Algorithm
The Euclidean algorithm is a more efficient method for finding the GCF of larger numbers. It involves repeatedly applying the division algorithm until the remainder is 0. The last non-zero remainder is the GCF.
Let's apply the Euclidean algorithm to find the GCF of 9 and 15:
- Divide the larger number (15) by the smaller number (9): 15 ÷ 9 = 1 with a remainder of 6.
- Replace the larger number with the smaller number (9) and the smaller number with the remainder (6): 9 ÷ 6 = 1 with a remainder of 3.
- Repeat the process: 6 ÷ 3 = 2 with a remainder of 0.
Since the last non-zero remainder is 3, the GCF of 9 and 15 is 3.
Expanding the Concept: GCFs and Their Applications in Advanced Mathematics
The concept of GCF extends beyond simple arithmetic problems. It plays a critical role in various areas of higher-level mathematics:
1. Abstract Algebra
In abstract algebra, the concept of GCF is generalized to the notion of the greatest common divisor (GCD) in more abstract algebraic structures like rings and ideals. The Euclidean algorithm can be adapted to find GCDs in these more general settings.
2. Cryptography
The Euclidean algorithm, which is used to find the GCF, is a fundamental component of many cryptographic algorithms, including RSA encryption. The efficiency of the Euclidean algorithm is crucial for the security and practicality of these systems.
3. Modular Arithmetic
GCFs are fundamental in understanding modular arithmetic, where operations are performed modulo some integer. The GCF is used to determine the existence and properties of solutions to linear congruences.
4. Continued Fractions
Continued fractions are a way to represent real numbers as an infinite sum of fractions. The GCF plays a crucial role in understanding the convergence properties of continued fractions.
Solving Problems using GCFs: Real-world Examples
Let's explore some practical applications of GCFs:
Example 1: Simplifying Fractions
Suppose you have the fraction 27/18. To simplify this fraction, you need to find the GCF of 27 and 18.
- Prime factorization of 27: 3³
- Prime factorization of 18: 2 x 3²
The GCF is 3². Dividing both the numerator and denominator by 9 (3²), we get the simplified fraction 3/2.
Example 2: Dividing Objects Equally
You have 15 red marbles and 9 blue marbles. You want to divide them into identical bags, with each bag containing the same number of red and blue marbles. To find the maximum number of bags you can create, you need to find the GCF of 15 and 9. As we've already established, the GCF is 3. Therefore, you can create 3 identical bags, each containing 5 red marbles and 3 blue marbles.
Example 3: Geometric Problems
You need to tile a rectangular floor with dimensions 15 feet by 9 feet using square tiles of equal size. To find the largest possible size of square tiles you can use without cutting any tiles, you need to find the GCF of 15 and 9. The GCF is 3, so you can use 3x3 feet square tiles.
Conclusion: Mastering GCFs for Mathematical Proficiency
Understanding the greatest common factor is essential for a solid foundation in mathematics. This concept, seemingly simple at first glance, opens up doors to more advanced mathematical concepts and finds applications in various fields. From simplifying fractions to solving complex algebraic equations and even securing cryptographic systems, the GCF is a fundamental tool in the mathematician's toolbox. By mastering the various methods for calculating the GCF, you equip yourself with a powerful problem-solving skill applicable across diverse mathematical domains. The seemingly simple question, "What is the GCF of 9 and 15?" thus becomes a gateway to a deeper understanding of the fascinating world of numbers and their relationships.
Latest Posts
Latest Posts
-
If Your 40 What Year Was You Born
May 10, 2025
-
How Long Is 168 Hours In Days
May 10, 2025
-
How Do You Say Grandma In Dutch
May 10, 2025
-
How Much Is 300 Denarii Worth Today
May 10, 2025
-
How Many Ounces Is One Slice Of Bread
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is The Gcf Of 9 And 15 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.