What Is The Greatest Common Factor Of 60 And 20
Arias News
May 12, 2025 · 5 min read
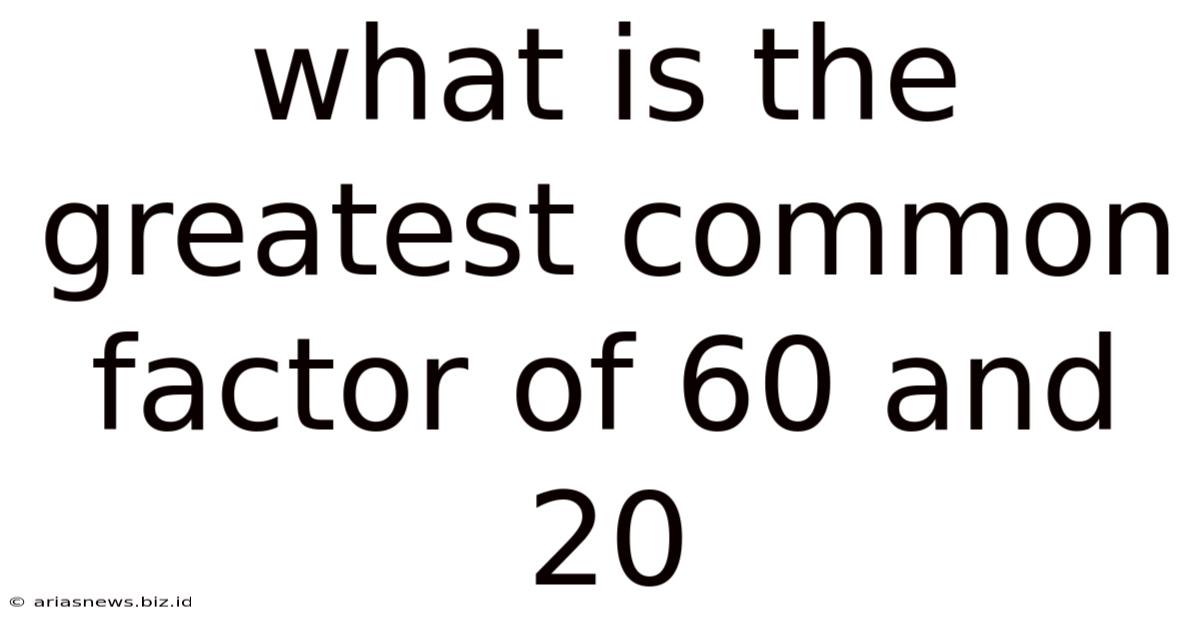
Table of Contents
What is the Greatest Common Factor of 60 and 20? A Deep Dive into Number Theory
Finding the greatest common factor (GCF) of two numbers might seem like a simple arithmetic task, but it's a concept with far-reaching implications in mathematics and beyond. Understanding GCFs is fundamental to simplifying fractions, solving algebraic equations, and even understanding more advanced mathematical concepts. This article will explore the GCF of 60 and 20 in detail, examining various methods to find it and highlighting its significance in different mathematical contexts.
Understanding Greatest Common Factor (GCF)
The greatest common factor (GCF), also known as the greatest common divisor (GCD), of two or more integers is the largest positive integer that divides each of the integers without leaving a remainder. In simpler terms, it's the biggest number that goes evenly into both numbers. Finding the GCF is a crucial skill in various mathematical operations and problem-solving scenarios.
Why is Finding the GCF Important?
The GCF plays a vital role in several mathematical applications, including:
-
Simplifying Fractions: Reducing a fraction to its simplest form requires finding the GCF of the numerator and the denominator. For example, simplifying 12/18 involves finding the GCF of 12 and 18, which is 6. This allows us to simplify the fraction to 2/3.
-
Solving Equations: GCF is often used in solving algebraic equations, particularly when dealing with factoring polynomials. Finding the GCF of the terms in a polynomial helps simplify the expression.
-
Geometry and Measurement: GCF finds applications in geometry when dealing with problems involving area, volume, and finding the largest possible square or cube that can fit into a larger area or volume.
-
Number Theory: GCF is a fundamental concept in number theory, laying the groundwork for understanding more complex concepts like the least common multiple (LCM) and modular arithmetic.
Methods for Finding the Greatest Common Factor
Several methods can be employed to determine the GCF of two numbers. Let's explore some of the most common approaches, focusing on finding the GCF of 60 and 20.
1. Listing Factors Method
This method involves listing all the factors of each number and identifying the largest factor common to both.
Factors of 60: 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, 60
Factors of 20: 1, 2, 4, 5, 10, 20
Comparing the lists, we find that the common factors are 1, 2, 4, 5, 10, and 20. The greatest common factor is 20.
2. Prime Factorization Method
This method involves expressing each number as a product of its prime factors. The GCF is then found by multiplying the common prime factors raised to the lowest power.
Prime factorization of 60: 2² x 3 x 5
Prime factorization of 20: 2² x 5
The common prime factors are 2² and 5. Therefore, the GCF is 2² x 5 = 4 x 5 = 20. This method is particularly efficient for larger numbers.
3. Euclidean Algorithm
The Euclidean algorithm is an efficient method for finding the GCF of two numbers, especially when dealing with larger numbers. It's based on the principle that the GCF of two numbers doesn't change if the larger number is replaced by its difference with the smaller number. This process is repeated until the two numbers are equal.
Let's apply the Euclidean algorithm to 60 and 20:
- 60 = 20 x 3 + 0
Since the remainder is 0, the GCF is the smaller number, which is 20.
The Significance of the GCF of 60 and 20
The fact that the GCF of 60 and 20 is 20 tells us several things:
-
20 is the largest number that divides both 60 and 20 without leaving a remainder. This is the core definition of the GCF.
-
60 is a multiple of 20 (60 = 20 x 3). This relationship is inherent in the concept of the GCF.
-
Simplifying fractions involving 60 and 20 is straightforward. For example, the fraction 60/20 simplifies directly to 3/1 or simply 3.
-
Applications in problem-solving. If you were working with a problem involving 60 objects and 20 objects and needed to divide them into equal groups of the largest possible size, the GCF of 20 would provide the answer – groups of 20.
Expanding the Concept: Least Common Multiple (LCM)
While the GCF focuses on the largest common divisor, the least common multiple (LCM) addresses the smallest common multiple of two or more numbers. The GCF and LCM are closely related. The product of the GCF and LCM of two numbers always equals the product of the two numbers.
In the case of 60 and 20:
- GCF(60, 20) = 20
- LCM(60, 20) = 60
Notice that GCF(60, 20) x LCM(60, 20) = 20 x 60 = 1200, which is equal to 60 x 20.
Real-World Applications of GCF and LCM
Beyond the realm of abstract mathematics, GCF and LCM have practical applications in various real-world scenarios:
-
Scheduling: Determining the timing of events that occur at different intervals. For example, two buses arrive at a station at intervals of 60 minutes and 20 minutes respectively. The LCM of 60 and 20 (60 minutes) would be the time they arrive simultaneously.
-
Cutting materials: Calculating the maximum size of identical pieces that can be cut from materials of different lengths. Imagine cutting pieces of wood of lengths 60 cm and 20 cm. The GCF would determine the longest possible identical pieces that can be cut.
-
Organizing groups: Dividing a group of people into smaller groups of equal size. If you have 60 students and 20 teachers, the GCF would give the largest possible group size where the ratio of students to teachers remains constant.
Conclusion: The GCF – A Cornerstone of Number Theory
The seemingly simple task of finding the greatest common factor of 60 and 20 reveals a fundamental concept in mathematics with wide-ranging applications. From simplifying fractions and solving equations to tackling real-world problems involving scheduling, cutting materials, and organizing groups, the GCF and its closely related concept, the LCM, are essential tools for anyone working with numbers. Understanding these concepts not only strengthens one's mathematical foundation but also enhances problem-solving skills across various fields. Mastering the different methods for finding the GCF, such as listing factors, prime factorization, and the Euclidean algorithm, provides a versatile toolkit for tackling numerical challenges with efficiency and confidence. The GCF, therefore, is far more than a simple arithmetic operation; it's a cornerstone of number theory and a practical tool with diverse real-world applications.
Latest Posts
Latest Posts
-
How Many 1 8 Teaspoons In 1 4 Teaspoon
May 12, 2025
-
Napa 21348 Oil Filter Fits What Vehicle
May 12, 2025
-
How To Say My Love In Portuguese
May 12, 2025
-
How Long Does It Take Pasta To Digest
May 12, 2025
-
How To Round To The Nearest 10 Thousand
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is The Greatest Common Factor Of 60 And 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.