What Is The Square Root Of 2 Squared
Arias News
May 10, 2025 · 5 min read
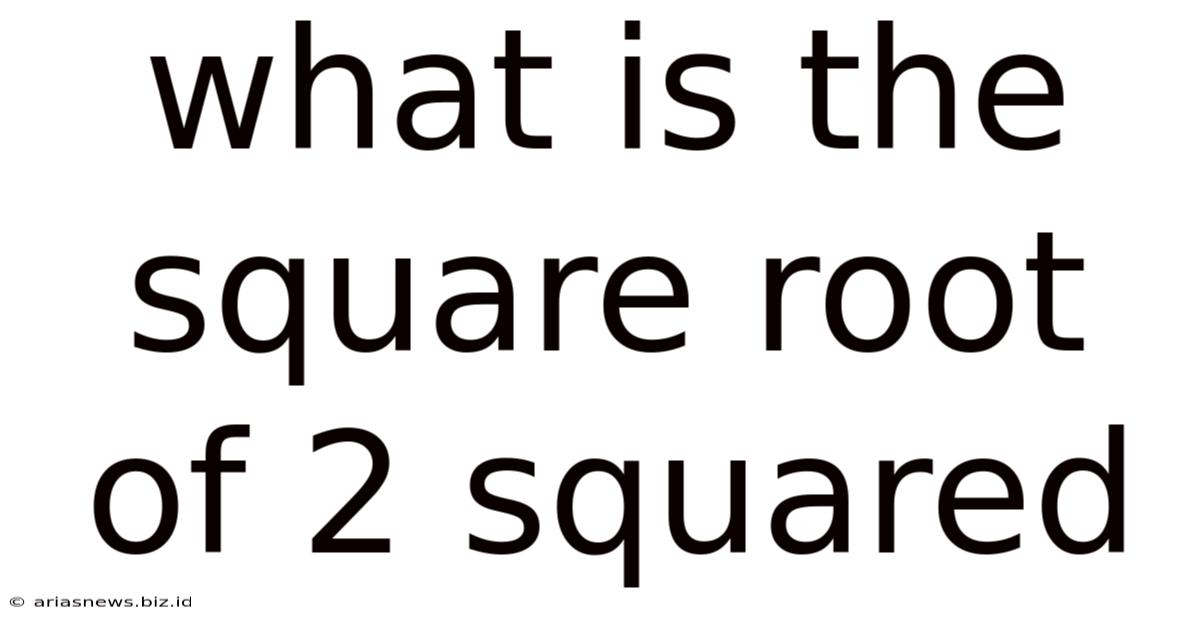
Table of Contents
What is the Square Root of 2 Squared? A Deep Dive into Mathematical Concepts
The question, "What is the square root of 2 squared?" might seem deceptively simple at first glance. However, exploring this seemingly basic problem opens doors to a deeper understanding of fundamental mathematical concepts, including squares, square roots, and the properties of real numbers. This article will not only answer the question directly but also delve into the underlying principles, offering a comprehensive exploration suitable for anyone from high school students to those looking to refresh their mathematical knowledge.
Understanding Squares and Square Roots
Before tackling the core question, let's solidify our understanding of the concepts involved: squares and square roots.
What is a Square?
In mathematics, squaring a number means multiplying the number by itself. For instance:
- 2 squared (written as 2²) = 2 * 2 = 4
- 5 squared (written as 5²) = 5 * 5 = 25
- x squared (written as x²) = x * x
Squaring a number always results in a positive value (or zero if the original number is zero). This is because multiplying two positive numbers or two negative numbers always yields a positive result.
What is a Square Root?
The square root of a number is a value that, when multiplied by itself, equals the original number. It's the inverse operation of squaring. For example:
- The square root of 4 (written as √4) is 2, because 2 * 2 = 4.
- The square root of 25 (written as √25) is 5, because 5 * 5 = 25.
It's crucial to note that while every positive number has two square roots (one positive and one negative), the principal square root (the one usually referred to) is always the positive value. For example, while both 2 and -2, when squared, result in 4, the principal square root of 4 is 2.
Answering the Core Question: √(2²)
Now, let's address the central question: What is the square root of 2 squared?
The expression √(2²) can be simplified using the order of operations (PEMDAS/BODMAS), which dictates that we perform the operations within the parentheses first.
-
Squaring 2: 2² = 2 * 2 = 4
-
Taking the square root: √4 = 2
Therefore, the square root of 2 squared is 2.
Exploring Further: Generalizing the Concept
The relationship between squaring and taking the square root is an important one in mathematics. We can generalize this concept:
For any non-negative real number 'x':
√(x²) = |x|
The absolute value symbol (| |) is crucial here. It ensures that the result is always non-negative, reflecting the fact that the principal square root is always positive or zero. For example:
- √(3²) = |3| = 3
- √((-3)²) = |-3| = 3
This generalization emphasizes the fundamental inverse relationship between squaring and the principal square root.
Beyond the Basics: Implications and Applications
The seemingly simple equation √(2²) = 2 has significant implications and broad applications across various mathematical fields. Let's explore some of these:
1. Simplifying Algebraic Expressions:
Understanding this relationship is fundamental to simplifying algebraic expressions. For example, consider the equation:
√(x² + 4x + 4)
This can be factored as √((x+2)²). Using our understanding of square roots and squares, we can simplify this expression to |x + 2|. This simplification greatly reduces complexity in further calculations.
2. Geometry and Pythagorean Theorem:
The Pythagorean theorem, a cornerstone of geometry, utilizes the concept of squares and square roots. The theorem states that in a right-angled triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. To find the length of a side, we often need to take the square root.
3. Calculus and Derivatives:
In calculus, the concept of square roots and squares is fundamental to finding derivatives and integrals. Understanding how to manipulate these expressions efficiently is crucial for solving complex calculus problems.
4. Complex Numbers:
While our initial discussion focused on real numbers, the concepts of squares and square roots extend into the realm of complex numbers. Complex numbers have a real and an imaginary part, and squaring and taking square roots of complex numbers involves different rules and interpretations.
5. Computer Science and Programming:
The concept of square roots is critical in computer science and programming. Many algorithms and calculations utilize square roots for various purposes, such as calculating distances, image processing, and game development. The efficiency of square root calculations is a significant aspect of optimizing program performance.
Addressing Potential Misconceptions
It's essential to address potential misconceptions surrounding the square root operation:
-
√(x²) ≠ x: This is only true if x is non-negative. If x is negative, √(x²) = |x| = -x.
-
√(a*b) ≠ √a * √b: This is incorrect, generally speaking. It's only valid for non-negative values of a and b.
-
Incorrect Order of Operations: Failure to follow the correct order of operations (PEMDAS/BODMAS) can lead to incorrect results. Always perform the operations within parentheses (or brackets) before dealing with exponents, roots, or other operations.
Conclusion: A Simple Question, Deep Understanding
The seemingly simple question, "What is the square root of 2 squared?" has led us on a journey through fundamental mathematical concepts, highlighting the critical relationship between squares and square roots. Understanding these concepts is crucial not just for solving basic problems but also for tackling more complex mathematical challenges in various fields. This exploration underscores the importance of a strong foundational understanding in mathematics and how seemingly simple concepts can unlock a deeper appreciation for the elegance and power of mathematical principles. By mastering these fundamentals, we build a stronger base for tackling more advanced mathematical and scientific pursuits. The seemingly simple question, therefore, serves as a powerful reminder of the interconnectedness of mathematical ideas and their wide-ranging applications.
Latest Posts
Latest Posts
-
Does Llc Need A Period After It
May 10, 2025
-
What Is The Gcf Of 28 And 48
May 10, 2025
-
How Much Is 1 Sprig Of Thyme
May 10, 2025
-
How Much Tequila In A Margarita Mix
May 10, 2025
-
How Long Is 157 Minutes In Hours
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is The Square Root Of 2 Squared . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.