What Percent Is 17 Out Of 19
Arias News
May 08, 2025 · 4 min read
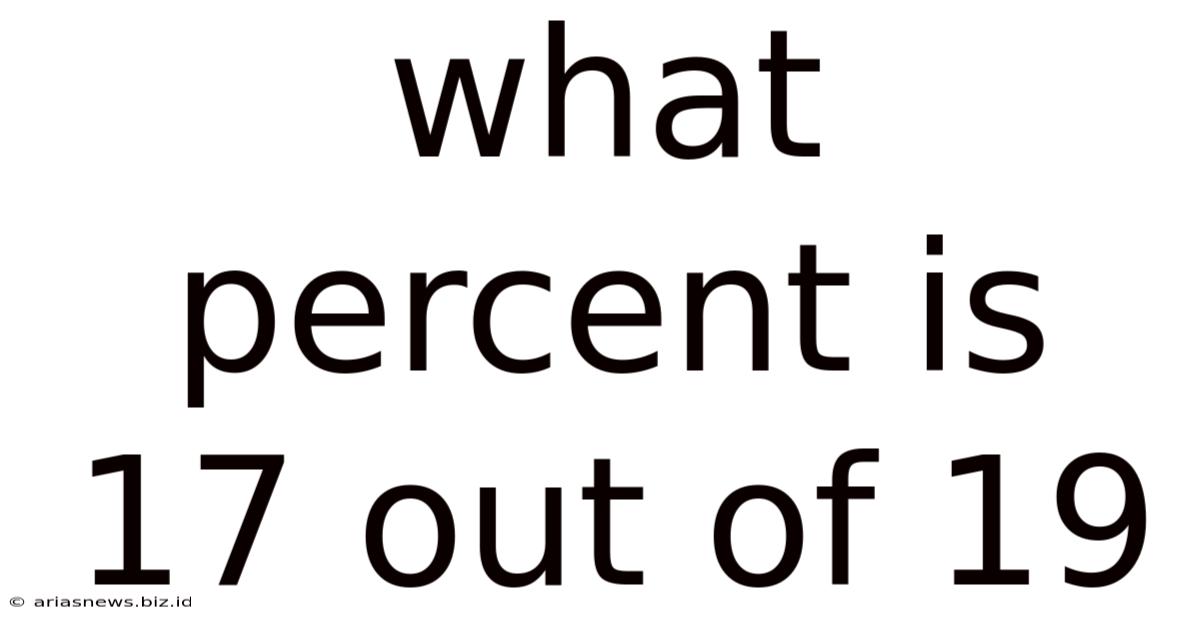
Table of Contents
What Percent is 17 out of 19? A Deep Dive into Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from everyday budgeting to complex scientific analysis. This article will meticulously explore how to calculate what percent 17 is out of 19, providing a comprehensive understanding of the underlying principles and offering various approaches to solve similar problems. We'll delve beyond the simple calculation, examining the context of percentages, practical applications, and troubleshooting common misconceptions.
Understanding Percentages: The Basics
Before tackling the specific problem of calculating the percentage of 17 out of 19, let's solidify our understanding of percentages. A percentage represents a fraction of 100. The term "percent" literally means "out of 100" (per centum in Latin). Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
This fundamental understanding allows us to convert any fraction into a percentage and vice versa. The key is to express the fraction as a value out of 100.
Method 1: The Direct Calculation Method
This method involves directly applying the formula for calculating percentages:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 17
- Whole: 19
Therefore, the calculation is:
(17 / 19) * 100% = 89.47% (approximately)
Thus, 17 out of 19 is approximately 89.47%.
This method is straightforward and easily applicable to various scenarios. However, understanding the underlying logic is crucial for tackling more complex percentage problems.
Method 2: Using Proportions
This method utilizes the concept of proportions to solve for the unknown percentage. We can set up a proportion:
17/19 = x/100
Where 'x' represents the unknown percentage. To solve for 'x', we cross-multiply:
17 * 100 = 19 * x
1700 = 19x
x = 1700 / 19
x ≈ 89.47
This method reinforces the understanding of the relationship between fractions and percentages, highlighting the equivalence between the two representations.
Method 3: Decimal Conversion
This approach involves converting the fraction into a decimal first, then multiplying by 100 to obtain the percentage.
-
Convert the fraction to a decimal: 17 / 19 ≈ 0.8947
-
Multiply the decimal by 100: 0.8947 * 100% = 89.47%
This method is particularly useful when dealing with more complex fractions or when using a calculator. The decimal representation provides a clear picture of the fraction's magnitude before converting it to a percentage.
Practical Applications: Where Percentages Matter
Understanding percentage calculations has far-reaching applications in diverse areas:
-
Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns. For instance, understanding what percentage 17 represents out of a total investment of 19 could be vital in assessing the performance of that investment.
-
Science: Expressing experimental results, analyzing data, and determining statistical significance. Percentage changes in scientific measurements are often crucial in interpreting trends and drawing conclusions.
-
Everyday Life: Calculating tips, sales tax, and discounts while shopping. Understanding percentages helps in making informed financial decisions and avoiding overspending.
-
Education: Calculating grades, assessing student performance, and interpreting standardized test scores. Percentages provide a standardized measure for comparing student performance across different tests and assignments.
-
Data Analysis: Representing data in charts and graphs, making comparisons between different data sets, and drawing meaningful insights from data. Percentages provide a clear and concise method to visualize data trends and patterns.
Rounding and Precision: Addressing the Approximation
Notice that in our calculations, we obtained an approximate value of 89.47%. This is because the result (17/19) is a non-terminating decimal. The level of precision needed depends on the context of the problem.
In some situations, rounding to the nearest whole number (90%) might suffice. In others, retaining more decimal places (e.g., 89.47368%) might be necessary for greater accuracy. The key is to choose a level of precision that appropriately balances accuracy with simplicity.
Common Mistakes to Avoid
Several common errors can arise when calculating percentages:
-
Incorrectly identifying the part and the whole: Ensuring correct identification of the 'part' and the 'whole' is crucial for accurate calculations. Misinterpreting these values will lead to an incorrect percentage.
-
Order of operations: Following the correct order of operations (PEMDAS/BODMAS) is crucial for accurate calculations, especially when dealing with more complex expressions involving percentages.
-
Improper rounding: Incorrect rounding can lead to significant errors, especially when dealing with financial calculations or scientific data analysis. Always consider the context and the required level of precision before rounding.
Advanced Applications and Extensions
While this article focuses on the basic calculation of what percent 17 is out of 19, the principles extend to more complex percentage problems:
-
Percentage change: Calculating the percentage increase or decrease between two values.
-
Percentage points: Understanding the difference between percentage change and percentage points, which are often confused.
-
Compound interest: Calculating the effect of compound interest over time.
-
Statistical analysis: Applying percentages in hypothesis testing and confidence intervals.
Conclusion: Mastering Percentage Calculations
Calculating what percent 17 is out of 19, and more broadly, mastering percentage calculations is essential for numerous applications. Understanding the underlying principles, using various methods (direct calculation, proportions, decimal conversion), and being mindful of potential errors will allow for accurate and confident percentage calculations in various contexts. Remember to choose the level of precision appropriate for the specific situation and always double-check your work to ensure accuracy. By mastering percentages, you equip yourself with a powerful tool for navigating the quantitative aspects of the world around you.
Latest Posts
Latest Posts
-
How Much Does A 16 X 16 Paver Weigh
May 08, 2025
-
What Is 2 To The 8 Power
May 08, 2025
-
What Is 10 Percent Of 10000 Dollars
May 08, 2025
-
Which Story Event Best Illustrates Crusoes Dominance
May 08, 2025
-
How Many Bottled Water Is 1 Liter
May 08, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 17 Out Of 19 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.