What Percentage Is 46 Out Of 60
Arias News
May 09, 2025 · 5 min read
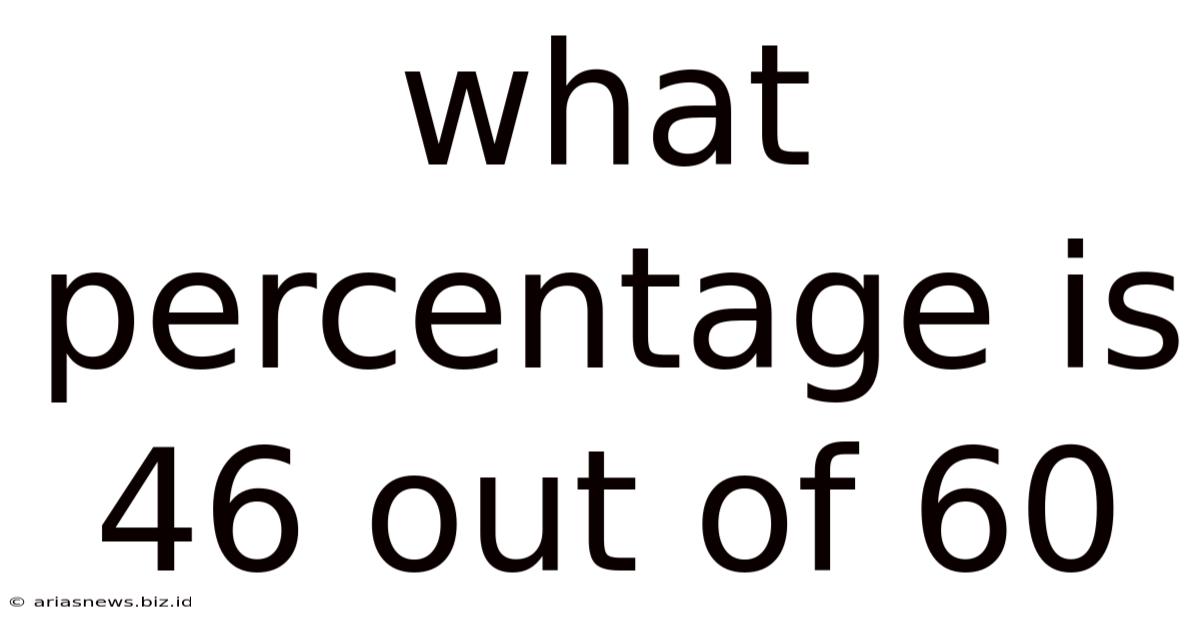
Table of Contents
What Percentage is 46 out of 60? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with wide-ranging applications in various aspects of life, from academic studies and professional settings to everyday decision-making. Understanding how to determine what percentage one number represents of another is crucial for interpreting data, making comparisons, and solving problems efficiently. This comprehensive guide will delve into the process of calculating what percentage 46 is of 60, providing step-by-step instructions, alternative methods, and real-world examples to solidify your understanding.
Understanding Percentages
Before diving into the calculation, let's briefly revisit the concept of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" is used to represent percentages. For instance, 50% means 50 out of 100, which is equivalent to 1/2 or 0.5.
Calculating "What Percentage is 46 out of 60?"
There are several ways to calculate what percentage 46 represents of 60. Let's explore the most common methods:
Method 1: Using the Formula
The most straightforward approach involves using the basic percentage formula:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 46
- Whole: 60
Substituting these values into the formula:
(46 / 60) * 100% = 76.67%
Therefore, 46 is 76.67% of 60.
Method 2: Converting to a Decimal
This method involves converting the fraction 46/60 into a decimal and then multiplying by 100% to express it as a percentage.
- Divide the part by the whole: 46 ÷ 60 = 0.766666...
- Multiply the decimal by 100%: 0.766666... * 100% ≈ 76.67%
Again, we arrive at the same result: 46 is approximately 76.67% of 60. Note that we round the result to two decimal places for practical purposes.
Method 3: Using Proportions
This method utilizes the concept of proportions to solve for the unknown percentage. We can set up a proportion as follows:
46/60 = x/100
Where 'x' represents the percentage we want to find. To solve for 'x', we cross-multiply:
60x = 4600
x = 4600 / 60
x ≈ 76.67
This method confirms our previous calculations: 46 is approximately 76.67% of 60.
Real-World Applications
Understanding percentage calculations is crucial in numerous real-world situations. Here are a few examples:
-
Academic Performance: If a student scores 46 out of 60 on a test, their percentage score is 76.67%. This allows for easy comparison with other students and assessment of performance.
-
Sales and Discounts: A store offering a discount might advertise a sale of 20% off. Calculating the final price after a 20% discount requires understanding percentage calculations. For example, if an item costs $60, a 20% discount would reduce the price by $12 (20% of $60), resulting in a final price of $48.
-
Financial Analysis: In finance, percentage changes are used to track investment performance, inflation rates, and other economic indicators. For example, a 5% increase in the value of a stock represents a significant change in its worth.
-
Surveys and Statistics: Percentages are frequently used to present survey results and statistical data. For instance, if 46 out of 60 respondents answered "yes" to a particular question, the percentage of "yes" responses is 76.67%.
-
Recipe Scaling: In cooking, scaling recipes up or down requires understanding proportions and percentages. If a recipe calls for 46 grams of flour out of a total of 60 grams of dry ingredients, this represents 76.67% of the dry ingredients.
Beyond the Basic Calculation: Understanding the Context
While the calculation itself is straightforward, the interpretation of the result is equally important. The context in which the percentage is calculated significantly impacts its meaning. Consider these points:
-
Sample Size: The reliability of a percentage depends on the sample size. A 76.67% success rate based on a sample of 60 might be less reliable than the same percentage based on a sample of 600. Larger sample sizes generally lead to more accurate and representative results.
-
Margin of Error: In statistical studies, it's crucial to consider the margin of error, which accounts for the uncertainty inherent in sampling. The margin of error indicates the range within which the true population percentage is likely to fall.
-
Comparison with Other Data: The meaning of 76.67% depends on the context. A score of 76.67% on a difficult exam might be considered excellent, while the same score on an easy exam might be less impressive. Always compare the percentage to relevant benchmarks or other data points.
Advanced Percentage Calculations
While this article focuses on the basic percentage calculation of 46 out of 60, let's briefly touch upon some more complex percentage scenarios:
-
Percentage Increase/Decrease: Calculating the percentage increase or decrease between two numbers involves finding the difference between the two numbers, dividing that difference by the original number, and multiplying by 100%.
-
Percentage of a Percentage: Calculating a percentage of a percentage involves multiplying the two percentages together. For instance, finding 20% of 50% would be calculated as (20/100) * (50/100) = 0.10 or 10%.
-
Compounding Percentages: This involves calculating the effect of multiple percentages applied sequentially, such as compound interest in finance.
Conclusion: Mastering Percentage Calculations
Understanding how to calculate percentages is a valuable skill that extends far beyond the classroom. From everyday tasks to complex professional applications, the ability to accurately and efficiently calculate percentages allows for informed decision-making and effective problem-solving. By mastering the basic methods and understanding the underlying principles, you can confidently navigate a wide range of situations requiring percentage calculations, ensuring accurate interpretations and effective communication of numerical data. Remember that the context surrounding the percentage is equally important as the calculation itself, ensuring a comprehensive understanding of the implications of your results.
Latest Posts
Latest Posts
-
How Much Is 8 Oz Of Meat
May 11, 2025
-
What Is The Greatest Common Factor Of 48 And 80
May 11, 2025
-
Is It Bad Luck To Eat A Koi Fish
May 11, 2025
-
Cuanto Es 24 Grados Centigrados En Fahrenheit
May 11, 2025
-
Greatest Common Factor Of 3 And 15
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 46 Out Of 60 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.