10 Out Of 20 As A Percentage
Arias News
May 11, 2025 · 5 min read
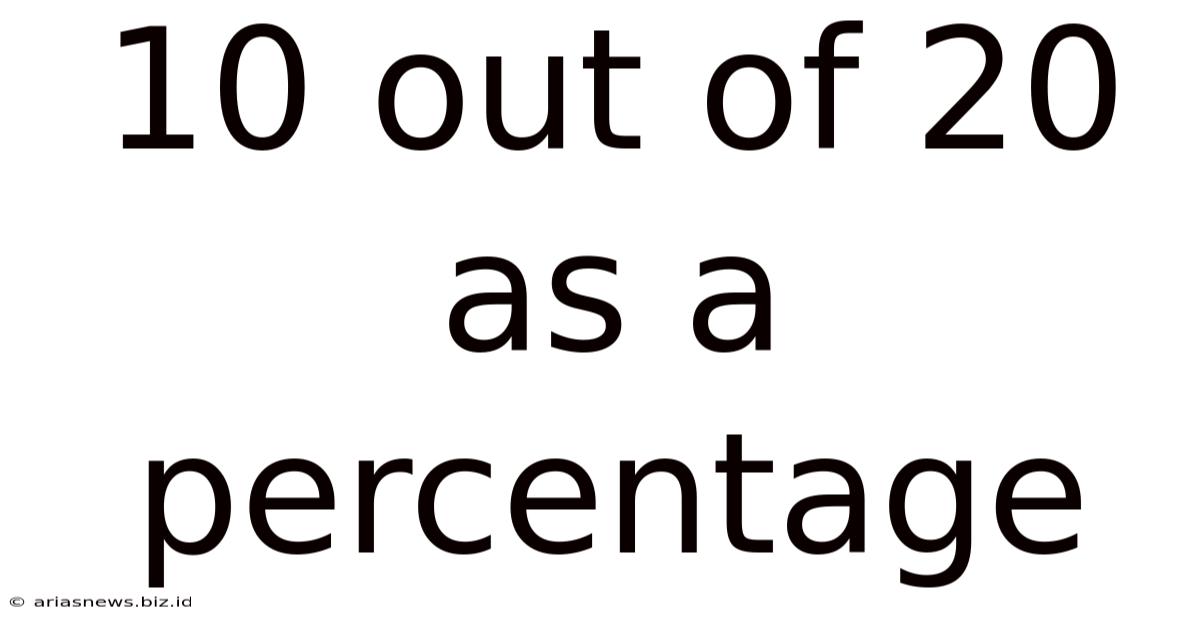
Table of Contents
10 Out of 20 as a Percentage: A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide will delve into the calculation of "10 out of 20 as a percentage," explaining the process step-by-step and exploring various related concepts. We'll also cover practical applications and common mistakes to avoid.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred" ("per cent" from Latin). Percentages are widely used because they provide a standardized way to compare proportions and make numerical data easier to understand.
Key Components:
- The Part: This represents the specific amount you're considering (in our case, 10).
- The Whole: This represents the total amount (in our case, 20).
- The Percentage: This is the fraction expressed as a number out of 100.
Calculating 10 Out of 20 as a Percentage: The Step-by-Step Process
There are several ways to calculate 10 out of 20 as a percentage. Let's explore the most common methods:
Method 1: Using the Fraction Method
- Express as a Fraction: Write the problem as a fraction: 10/20.
- Simplify the Fraction: Reduce the fraction to its simplest form by dividing both the numerator (top) and the denominator (bottom) by their greatest common divisor (GCD), which is 10 in this case: 10/20 simplifies to 1/2.
- Convert to a Decimal: Divide the numerator by the denominator: 1 ÷ 2 = 0.5
- Convert to a Percentage: Multiply the decimal by 100 and add the "%" symbol: 0.5 x 100 = 50%.
Therefore, 10 out of 20 is 50%.
Method 2: Using the Proportion Method
This method directly establishes a proportion between the part, the whole, and the percentage.
- Set up a Proportion: We can set up a proportion: 10/20 = x/100, where 'x' represents the percentage we want to find.
- Cross-Multiply: Cross-multiply the proportion: 10 * 100 = 20 * x
- Solve for x: This simplifies to 1000 = 20x. Divide both sides by 20: x = 50.
- Add the Percentage Symbol: x = 50%, thus 10 out of 20 is 50%.
Method 3: Using the Percentage Formula
The general formula for calculating a percentage is:
(Part / Whole) * 100%
- Substitute Values: Substitute the values from our problem: (10 / 20) * 100%
- Calculate: (0.5) * 100% = 50%
This confirms that 10 out of 20 is 50%.
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in many real-world situations. Here are some examples:
1. Academic Performance:
Imagine a student scores 10 out of 20 on a test. Using the methods above, we can easily determine that their score is 50%. This allows for a clear understanding of their performance relative to the total possible marks.
2. Sales and Discounts:
A store offers a 50% discount on an item. If the original price is $20, the discount amount is 50% of $20, which is ($50/100) * $20 = $10. The final price after the discount would be $20 - $10 = $10.
3. Financial Calculations:
Interest rates, tax calculations, and investment returns are all expressed as percentages. Understanding percentages helps in making informed financial decisions. For instance, if an investment earns 10% interest on a $1000 investment, the interest earned is $100.
4. Data Analysis:
Percentages are essential for analyzing data in various fields. For example, if a survey shows that 10 out of 20 respondents prefer a particular product, it indicates a 50% preference rate.
5. Everyday Life:
Percentages are encountered in everyday activities such as calculating tips, understanding sales tax, and even comparing the nutritional value of foods.
Common Mistakes to Avoid When Calculating Percentages
While calculating percentages seems straightforward, some common mistakes can lead to incorrect results. Let's address these:
1. Incorrect Order of Operations:
When using the formula (Part / Whole) * 100%, ensure that the division is performed before the multiplication. Failing to follow the order of operations will yield an incorrect result.
2. Misunderstanding the "Whole":
Accurately identifying the "whole" is crucial. The "whole" represents the total amount against which the part is being compared. Misidentifying the "whole" will lead to an inaccurate percentage.
3. Decimal Point Errors:
When converting decimals to percentages, remember to multiply by 100. Forgetting to do so or misplacing the decimal point is a common error.
4. Incorrect Simplification of Fractions:
When simplifying fractions, ensure that you divide both the numerator and the denominator by their greatest common divisor. Incorrect simplification will lead to an incorrect percentage.
Advanced Percentage Calculations and Related Concepts
Beyond the basic calculation of 10 out of 20, let's explore some more advanced concepts:
1. Percentage Increase and Decrease:
These calculations are used to determine the percentage change between two values. The formula for percentage increase is: [(New Value - Old Value) / Old Value] * 100%. The formula for percentage decrease is similar but uses the absolute difference between the old and new values.
2. Percentage Points:
It is important to differentiate between percentage change and percentage points. A change from 20% to 30% is a 10 percentage point increase, but a 50% increase in the percentage itself.
3. Compound Interest:
This involves earning interest on both the principal amount and accumulated interest. Compound interest calculations use exponential growth, making them more complex than simple interest calculations.
4. Percentage of a Percentage:
This involves finding a percentage of a percentage, which often requires multiplying two percentages together, then dividing by 100.
Conclusion: Mastering Percentage Calculations
This guide has provided a comprehensive overview of calculating "10 out of 20 as a percentage" and related concepts. Mastering percentage calculations is a valuable skill applicable in numerous contexts. By understanding the fundamental methods, avoiding common errors, and exploring advanced applications, you can confidently navigate situations requiring percentage calculations in your personal and professional life. Remember to practice regularly to enhance your proficiency and build confidence in working with percentages.
Latest Posts
Latest Posts
-
Whats 5 To The Power Of 5
May 12, 2025
-
How Much Is 2 Stalks Of Green Onion
May 12, 2025
-
How Do You Say Delicious In Chinese
May 12, 2025
-
What Travels At The Speed Of Light Figgerits
May 12, 2025
-
Least Common Multiple Of 10 And 9
May 12, 2025
Related Post
Thank you for visiting our website which covers about 10 Out Of 20 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.