Is 1 6 Bigger Than 1 4
Arias News
May 08, 2025 · 5 min read
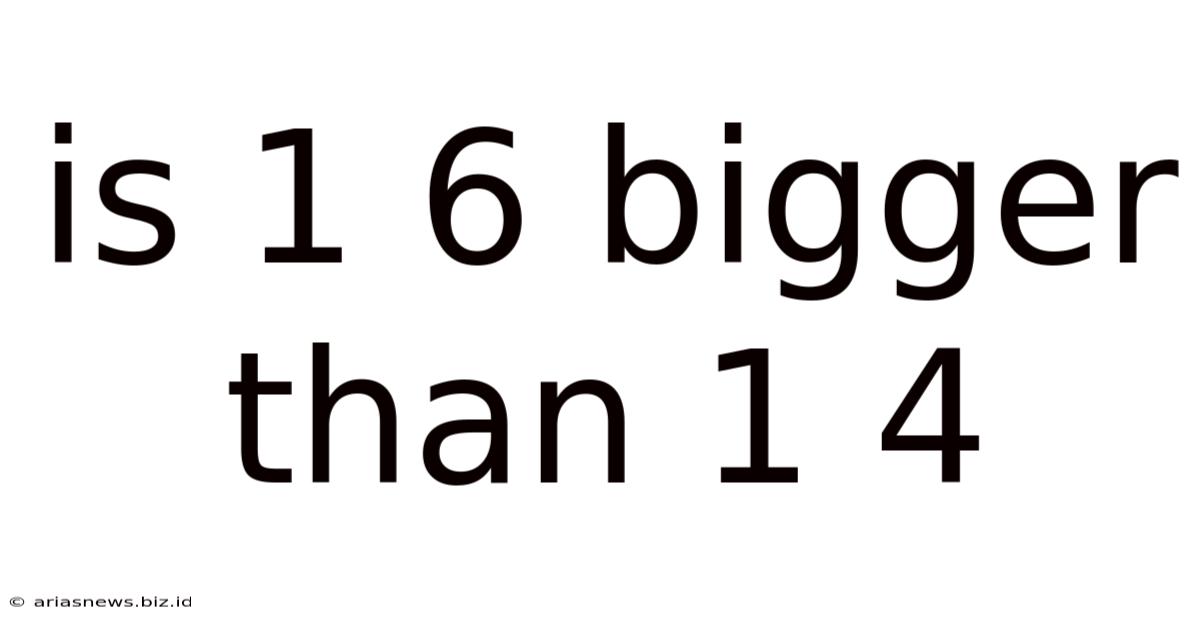
Table of Contents
Is 1/6 Bigger Than 1/4? Understanding Fractions and Comparisons
The question, "Is 1/6 bigger than 1/4?" might seem simple at first glance, but it's a fundamental concept in mathematics that underpins many more complex calculations. Understanding how to compare fractions is crucial for success in various fields, from basic arithmetic to advanced calculus. This comprehensive guide will not only answer this specific question but also equip you with the knowledge and techniques to compare any two fractions confidently.
Visualizing Fractions: A Helpful Approach
Before diving into the numerical methods, let's visualize the fractions 1/6 and 1/4. Imagine a pizza cut into six equal slices (representing 1/6) and another pizza cut into four equal slices (representing 1/4). Which pizza slice is larger? Intuitively, you'll likely realize that a slice from a pizza cut into four pieces will be bigger than a slice from a pizza cut into six pieces. This visual representation helps solidify the understanding that 1/4 is indeed larger than 1/6.
The Importance of Visual Aids in Fraction Comprehension
Visual aids, like the pizza analogy, are invaluable tools, especially when teaching children or anyone new to fractions. They offer a concrete and relatable way to grasp abstract concepts. Other visual aids could include:
- Fraction circles: These are physical or digital circles divided into different segments, visually representing various fractions.
- Number lines: Marking fractions on a number line provides a spatial understanding of their relative sizes.
- Area models: Shading portions of shapes to represent fractions allows for direct comparison.
Numerical Methods for Comparing Fractions
While visualization is helpful, mastering numerical methods is essential for more complex fraction comparisons. Here are the common approaches:
1. Finding a Common Denominator
This is the most common and reliable method. The denominator (the bottom number) represents the total number of parts, while the numerator (the top number) represents the number of parts we're considering. To compare 1/6 and 1/4, we need to find a common denominator – a number that is divisible by both 6 and 4. The least common multiple (LCM) of 6 and 4 is 12.
-
Converting to equivalent fractions: We convert both fractions to have a denominator of 12:
- 1/6 = (1 × 2) / (6 × 2) = 2/12
- 1/4 = (1 × 3) / (4 × 3) = 3/12
-
Comparing the numerators: Now that the denominators are the same, we simply compare the numerators: 2/12 and 3/12. Since 3 > 2, we conclude that 3/12 (or 1/4) is larger than 2/12 (or 1/6).
2. Converting Fractions to Decimals
Another effective method is converting fractions to decimals. This allows for easy comparison using decimal place values.
-
Converting 1/6 to a decimal: 1 ÷ 6 ≈ 0.1667
-
Converting 1/4 to a decimal: 1 ÷ 4 = 0.25
-
Comparing the decimals: Since 0.25 > 0.1667, we confirm that 1/4 is greater than 1/6.
3. Cross-Multiplication
This method is a shortcut for comparing two fractions directly without finding a common denominator.
-
Cross-multiply: Multiply the numerator of the first fraction by the denominator of the second fraction, and vice versa.
- (1 × 4) = 4
- (1 × 6) = 6
-
Compare the products: Since 4 < 6, the fraction with the smaller product (1/6) is the smaller fraction. Therefore, 1/4 is larger.
Expanding on Fraction Comparisons: More Complex Scenarios
The principles discussed above apply to comparing more complex fractions as well. Let’s consider some examples:
Comparing Fractions with Larger Numerators and Denominators
Let's compare 5/8 and 7/12. Using the common denominator method:
- Find the LCM of 8 and 12: The LCM is 24.
- Convert to equivalent fractions:
- 5/8 = (5 × 3) / (8 × 3) = 15/24
- 7/12 = (7 × 2) / (12 × 2) = 14/24
- Compare: 15/24 > 14/24, therefore, 5/8 > 7/12.
Comparing Mixed Numbers
Mixed numbers contain a whole number and a fraction (e.g., 2 1/3). To compare mixed numbers, first convert them to improper fractions (where the numerator is larger than the denominator). Then, apply the methods described above.
For instance, comparing 2 1/3 and 1 3/4:
- Convert to improper fractions:
- 2 1/3 = (2 × 3 + 1) / 3 = 7/3
- 1 3/4 = (1 × 4 + 3) / 4 = 7/4
- Find a common denominator (12):
- 7/3 = (7 × 4) / (3 × 4) = 28/12
- 7/4 = (7 × 3) / (4 × 3) = 21/12
- Compare: 28/12 > 21/12, therefore, 2 1/3 > 1 3/4.
Practical Applications of Fraction Comparison
The ability to compare fractions is essential in many real-world situations:
- Cooking and Baking: Following recipes often involves understanding fraction measurements.
- Construction and Engineering: Precise measurements and calculations rely on accurate fraction comparisons.
- Finance and Budgeting: Managing money and understanding portions of a whole involve fraction manipulation.
- Data Analysis: Interpreting data often involves comparing fractions or proportions.
Conclusion: Mastering Fraction Comparisons
Determining whether 1/6 is bigger than 1/4 involves understanding the fundamental principles of fractions. By employing visual aids, finding common denominators, converting to decimals, or cross-multiplying, we confidently conclude that 1/4 is bigger than 1/6. This seemingly simple comparison serves as a stepping stone to more advanced mathematical concepts. Mastering these techniques empowers you to handle more complex fraction comparisons with ease and confidence, opening doors to a broader understanding of mathematics and its diverse applications in the real world. Consistent practice and the utilization of various methods will solidify your understanding and improve your speed and accuracy in comparing fractions. Remember, practice makes perfect!
Latest Posts
Latest Posts
-
How To Convert 6 11 To A Decimal
May 08, 2025
-
How Much Does A 4x8 Sheet Of Plywood Weigh
May 08, 2025
-
How Many Pounds In 1 Cubic Foot
May 08, 2025
-
3am To 3pm Is How Many Hours
May 08, 2025
-
How Many 3 8 Are In 1
May 08, 2025
Related Post
Thank you for visiting our website which covers about Is 1 6 Bigger Than 1 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.