Is 42 A Prime Number Or A Composite Number
Arias News
Apr 01, 2025 · 5 min read
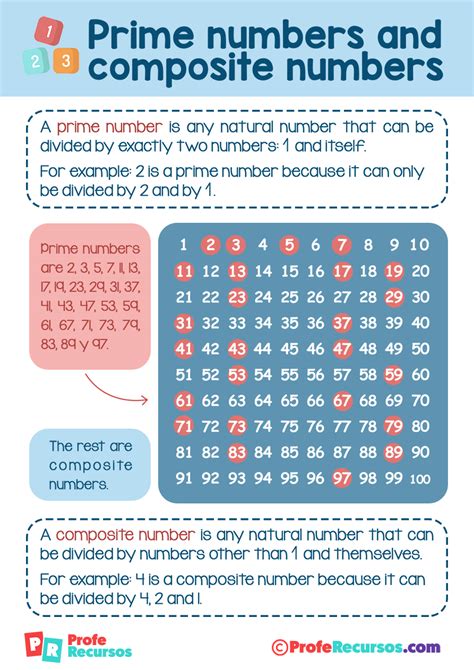
Table of Contents
Is 42 a Prime Number or a Composite Number? A Deep Dive into Number Theory
The question, "Is 42 a prime number or a composite number?" might seem simple at first glance. However, understanding the answer requires a foundational grasp of number theory, specifically the definitions of prime and composite numbers. This article will not only answer this specific question definitively but will also explore the broader concepts of prime factorization, divisibility rules, and the significance of prime numbers in mathematics.
Understanding Prime and Composite Numbers
Before we delve into the specifics of 42, let's establish a clear understanding of the terminology.
Prime numbers are whole numbers greater than 1 that have only two distinct positive divisors: 1 and themselves. This means they are not divisible by any other whole number without leaving a remainder. Examples include 2, 3, 5, 7, 11, and so on. Prime numbers are the building blocks of all other whole numbers.
Composite numbers, on the other hand, are whole numbers greater than 1 that have more than two positive divisors. In other words, they are divisible by at least one number other than 1 and themselves. Examples include 4 (divisible by 1, 2, and 4), 6 (divisible by 1, 2, 3, and 6), 9, 10, and so forth.
The number 1 is neither prime nor composite. It's considered a unique unit in number theory.
Determining if 42 is Prime or Composite
Now, let's analyze the number 42. To determine whether it's prime or composite, we need to see if it's divisible by any whole numbers other than 1 and 42. We can start by checking for divisibility by small prime numbers.
- Divisibility by 2: 42 is an even number, meaning it's divisible by 2. Therefore, 42 has at least three divisors: 1, 2, and 42.
Since 42 has more than two divisors, we can immediately conclude that 42 is a composite number. There's no need to check for divisibility by other prime numbers; the fact that it's divisible by 2 is sufficient to classify it as composite.
Prime Factorization of 42
Understanding the prime factorization of a number is crucial in number theory. Prime factorization is the process of expressing a composite number as a product of its prime factors. Let's find the prime factorization of 42:
- We know 42 is divisible by 2: 42 = 2 x 21
- Now, let's factorize 21. 21 is divisible by 3: 21 = 3 x 7
- Both 3 and 7 are prime numbers.
Therefore, the prime factorization of 42 is 2 x 3 x 7. This means 42 can be expressed as a unique product of prime numbers. This is a fundamental concept in number theory and is used in various applications, including cryptography.
Divisibility Rules: A Helpful Tool
Knowing divisibility rules can significantly speed up the process of determining whether a number is prime or composite. Here are a few commonly used divisibility rules:
- Divisibility by 2: A number is divisible by 2 if its last digit is even (0, 2, 4, 6, or 8).
- Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3.
- Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5.
- Divisibility by 11: Alternately add and subtract the digits of the number. If the result is divisible by 11, then the number is divisible by 11.
Applying these rules to 42:
- Divisibility by 2: The last digit is 2 (even), so 42 is divisible by 2.
- Divisibility by 3: 4 + 2 = 6, which is divisible by 3, so 42 is divisible by 3.
These quick checks immediately confirm that 42 is a composite number.
The Significance of Prime Numbers
Prime numbers are fundamental in mathematics and have far-reaching applications in various fields. Their significance stems from their unique properties and their role as the building blocks of all other whole numbers.
- Cryptography: Prime numbers are the cornerstone of many modern encryption algorithms. The difficulty of factoring large numbers into their prime factors is the basis of the security of these systems.
- Number Theory: Prime numbers are central to many branches of number theory, including the study of prime distribution, the Riemann hypothesis, and other profound mathematical questions.
- Computer Science: Prime numbers are used in hash table algorithms, random number generators, and other computational applications.
- Coding Theory: Prime numbers are employed in error-correcting codes, ensuring data integrity during transmission and storage.
Beyond 42: Exploring Other Numbers
Let's examine a few more examples to solidify our understanding of prime and composite numbers:
-
Is 97 a prime number? To determine this, we need to check for divisibility by prime numbers less than the square root of 97 (approximately 9.8). We find that 97 is not divisible by 2, 3, 5, 7. Further checking reveals that 97 is only divisible by 1 and 97. Therefore, 97 is a prime number.
-
Is 105 a composite number? We can use divisibility rules. 105 is divisible by 5 (ends in 5). Therefore, 105 is a composite number. Its prime factorization is 3 x 5 x 7.
-
Is 1 a prime number? No. By definition, a prime number must be greater than 1 and have only two distinct positive divisors: 1 and itself. 1 only has one divisor, which is 1. Therefore, 1 is neither prime nor composite.
Conclusion: The Composite Nature of 42
In conclusion, the answer to the question "Is 42 a prime number or a composite number?" is definitively composite. Its divisibility by 2 (and also 3 and 7) readily demonstrates this. Understanding the concepts of prime and composite numbers, along with divisibility rules and prime factorization, is crucial for grasping fundamental mathematical concepts and their applications in various fields. This exploration goes beyond a simple yes or no answer; it delves into the rich world of number theory and highlights the significance of prime numbers in mathematics and beyond. Remember to utilize divisibility rules and prime factorization techniques to quickly and efficiently determine whether any given number is prime or composite.
Latest Posts
Latest Posts
-
How Many Cups In 2 Liter Bottle Of Soda
Apr 02, 2025
-
Which Innovation Is Attributed To Geoffrey Chaucer
Apr 02, 2025
-
23 Out Of 31 As A Percentage
Apr 02, 2025
-
How Much Is 64 Ounces In Gallons
Apr 02, 2025
-
How Many Times Can 8 Go Into 60
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Is 42 A Prime Number Or A Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
