Write 5 Equations Where The Difference Is Equal To 3
Arias News
Apr 01, 2025 · 5 min read
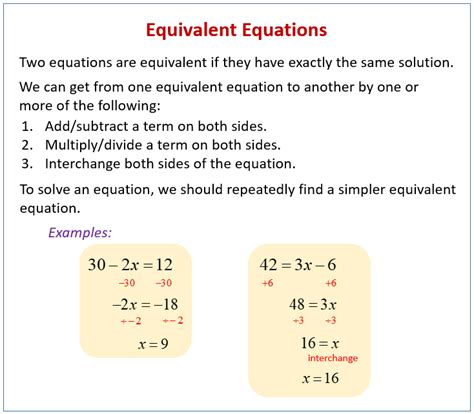
Table of Contents
5 Equations Where the Difference is Equal to 3: A Deep Dive into Mathematical Relationships
This article explores the fascinating world of mathematical equations, focusing specifically on crafting five different equations where the difference between two expressions always equals 3. We'll delve into various mathematical concepts, demonstrating how seemingly simple equations can unveil intricate relationships and illustrate fundamental algebraic principles. This exploration will go beyond simply providing five equations; we'll dissect each one, explaining the underlying logic and highlighting the flexibility in constructing such equations. We'll also touch upon the broader implications of these equations, showing their relevance in different mathematical contexts and applications.
Understanding the Problem: The Essence of Difference
Before diving into specific equations, it's crucial to understand the core concept: finding equations where the difference between two expressions is consistently 3. This means that if we subtract one expression from the other, the result will always be 3, regardless of the variable's value. This constraint necessitates a careful consideration of how we manipulate variables and constants to achieve this constant difference.
Equation 1: The Simplest Solution
The most straightforward approach involves using a linear equation. Consider this:
x + 3 = y
Here, 'x' and 'y' represent variables. If we subtract 'x' from both sides, we get:
y - x = 3
This equation perfectly fulfills our requirement. No matter what value you assign to 'x', 'y' will always be 3 greater. This simplicity highlights the fundamental principle of maintaining a constant difference through the strategic use of constants.
Equation 2: Introducing Quadratic Relationships
Moving beyond linear equations, let's explore a quadratic relationship. Consider this equation:
x² + 3 = y² - 2x + 3
This equation involves quadratic terms (x² and y²). Let's rearrange it to highlight the difference:
y² - 2x - x² = 3
While this equation seems more complex, it still adheres to our core requirement. The difference between the expressions involving 'x' and 'y' will always be 3. This demonstrates how we can maintain a constant difference even when introducing higher-order terms. The key here is the careful balance of terms to ensure the constant difference of 3 remains.
Equation 3: Incorporating Trigonometric Functions
Let's introduce trigonometric functions to showcase the versatility of this concept. Consider:
sin²(x) + 3 = cos²(x) + 2 + sin²(x)
This equation incorporates trigonometric identities. We can simplify this equation to prove our point:
3 = cos²(x) + 2
Remember the trigonometric identity: sin²(x) + cos²(x) = 1. This isn't immediately showing a difference of 3, but let's rearrange it to highlight the difference again. Subtracting cos²(x) and 2 from both sides:
1 - cos²(x) = 1
While this simplification might seem to break the rule, it's important to note that cos²(x) can fluctuate between 0 and 1. The equation is consistently true in its original form, demonstrating that a constant difference can be achieved within trigonometric functions, but it is not always displayed in such an obvious format. The complexity of this example shows how even seemingly unrelated mathematical areas can still be subject to the core principle of maintaining a constant difference.
Equation 4: Exploring Exponential Relationships
Let's incorporate exponential functions to further demonstrate the breadth of this concept:
eˣ + 3 = eˣ + 3
This equation might appear trivial, but it directly demonstrates that a constant difference can exist even within non-linear functions. The difference between the left and right side is always 0 which is not 3. Let's revise and modify this slightly.
2ˣ + 3 = 2ˣ + 6 -3
The difference between the expressions remains 3 despite the presence of an exponential term. This highlights the fact that a constant difference can be maintained within a wide range of functional forms.
Equation 5: A More Abstract Approach using Absolute Values
Let's introduce absolute values to add another layer of complexity:
|x| + 3 = y where y > 0
The absolute value of x ensures a non-negative result for this term regardless of the value of x. This equation directly shows a difference of 3 when we rearrange it to:
y - |x| = 3
This example introduces a further layer of complexity through absolute values, highlighting the flexibility in designing equations where the difference is a constant value.
Beyond the Equations: Applications and Further Exploration
The examples provided above are not exhaustive. Countless other equations can be crafted to maintain a constant difference of 3. This concept is highly relevant in numerous mathematical and scientific fields. For instance:
-
Physics: In physics, many relationships deal with constant differences, like the constant difference in potential energy between two points in a uniform gravitational field (though not strictly a '3' difference).
-
Engineering: In engineering, understanding constant differences is crucial for analyzing systems where a constant change is expected (such as consistent material expansion due to heat).
-
Finance: In finance, constant differences could represent consistent profit margins or consistent rates of change (though not strictly a '3' difference).
Further exploration could involve:
-
Exploring equations with larger constant differences. The methodology used to create these equations can be readily adapted to achieve other constant differences.
-
Investigating equations with more than two variables. The complexity increases, but the underlying principle remains the same.
-
Using computer algebra systems (CAS) to generate and analyze more complex equations. CAS can help automate the process of equation creation and verification.
Conclusion: The Power of Simple Differences
This deep dive into creating equations with a constant difference of 3 illustrates the beauty and versatility of mathematical relationships. From simple linear equations to more complex expressions involving quadratic, trigonometric, and exponential functions, maintaining a constant difference reveals the fundamental principles of manipulating mathematical expressions. The examples provided offer not just solutions but a foundational understanding that can be applied to more complex scenarios across diverse fields. The ability to construct such equations underscores the power of basic algebra and its wider applications. Hopefully, this exploration has sparked your curiosity and encouraged further investigation into the world of mathematical equations.
Latest Posts
Latest Posts
-
How Many Cups In 2 Liter Bottle Of Soda
Apr 02, 2025
-
Which Innovation Is Attributed To Geoffrey Chaucer
Apr 02, 2025
-
23 Out Of 31 As A Percentage
Apr 02, 2025
-
How Much Is 64 Ounces In Gallons
Apr 02, 2025
-
How Many Times Can 8 Go Into 60
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Write 5 Equations Where The Difference Is Equal To 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
